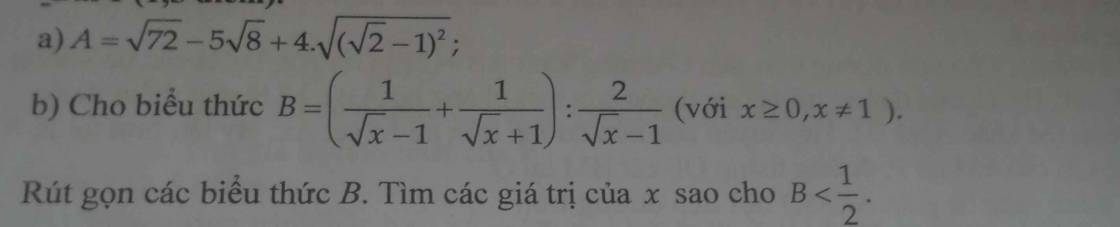Lời giải:
a.
$A=\sqrt{72}-5\sqrt{8}+4\sqrt{(\sqrt{2}-1)^2}$
$=6\sqrt{2}-10\sqrt{2}+4(\sqrt{2}-1)=-4$
b.
\(B=\frac{\sqrt{x}+1+\sqrt{x}-1}{(\sqrt{x}-1)(\sqrt{x}+1)}.\frac{\sqrt{x}-1}{2}=\frac{2\sqrt{x}}{(\sqrt{x}-1)(\sqrt{x}+1)}.\frac{\sqrt{x}-1}{2}=\frac{\sqrt{x}}{\sqrt{x}+1}\)
\(B< \frac{1}{2}\\ \Leftrightarrow \frac{\sqrt{x}}{\sqrt{x}+1}< \frac{1}{2}\\ \Leftrightarrow 2\sqrt{x}< \sqrt{x}+1\\ \Leftrightarrow \sqrt{x}<1\\ \Leftrightarrow 0\leq x< 1\)
Kết hợp với ĐKXĐ suy ra $0\leq x< 1$