Bài 2:
a: \(Q=\left(\dfrac{x+2}{x\sqrt{x}-1}+\dfrac{\sqrt{x}}{x+\sqrt{x}+1}+\dfrac{1}{1-\sqrt{x}}\right):\dfrac{\sqrt{x}-1}{5}\)
\(=\left(\dfrac{x+2}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}+\dfrac{\sqrt{x}}{x+\sqrt{x}+1}-\dfrac{1}{\sqrt{x}-1}\right)\cdot\dfrac{5}{\sqrt{x}-1}\)
\(=\dfrac{x+2+\sqrt{x}\left(\sqrt{x}-1\right)-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\cdot\dfrac{5}{\sqrt{x}-1}\)
\(=\dfrac{-\sqrt{x}+1+x-\sqrt{x}}{\left(\sqrt{x}-1\right)^2}\cdot\dfrac{5}{x+\sqrt{x}+1}\)
\(=\dfrac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}-1\right)^2}\cdot\dfrac{5}{x+\sqrt{x}+1}=\dfrac{5}{x+\sqrt{x}+1}\)
b: Khi x=4-2căn 3 thì \(Q=\dfrac{5}{4-2\sqrt{3}+\sqrt{4-2\sqrt{3}}+1}\)
\(=\dfrac{5}{5-2\sqrt{3}+\sqrt{3}-1}=\dfrac{5}{4-\sqrt{3}}=\dfrac{20+5\sqrt{3}}{13}\)
c: \(x+\sqrt{x}+1=\sqrt{x}\left(\sqrt{x}+1\right)+1>=1>0\forall x\) thỏa mãn ĐKXĐ
5>0
Do đó: \(Q=\dfrac{5}{x+\sqrt{x}+1}>0\forall x\) thỏa mãn ĐKXĐ
d: \(Q\cdot\left(\dfrac{x+\sqrt{x}+1}{\sqrt{x}+1}\right)< 1\)
=>\(\dfrac{5}{x+\sqrt{x}+1}\cdot\dfrac{x+\sqrt{x}+1}{\sqrt{x}+1}< 1\)
=>\(\dfrac{5}{\sqrt{x}+1}< 1\)
=>\(\dfrac{5-\sqrt{x}-1}{\sqrt{x}+1}< 0\)
=>\(4-\sqrt{x}< 0\)
=>\(\sqrt{x}>4\)
=>x>16








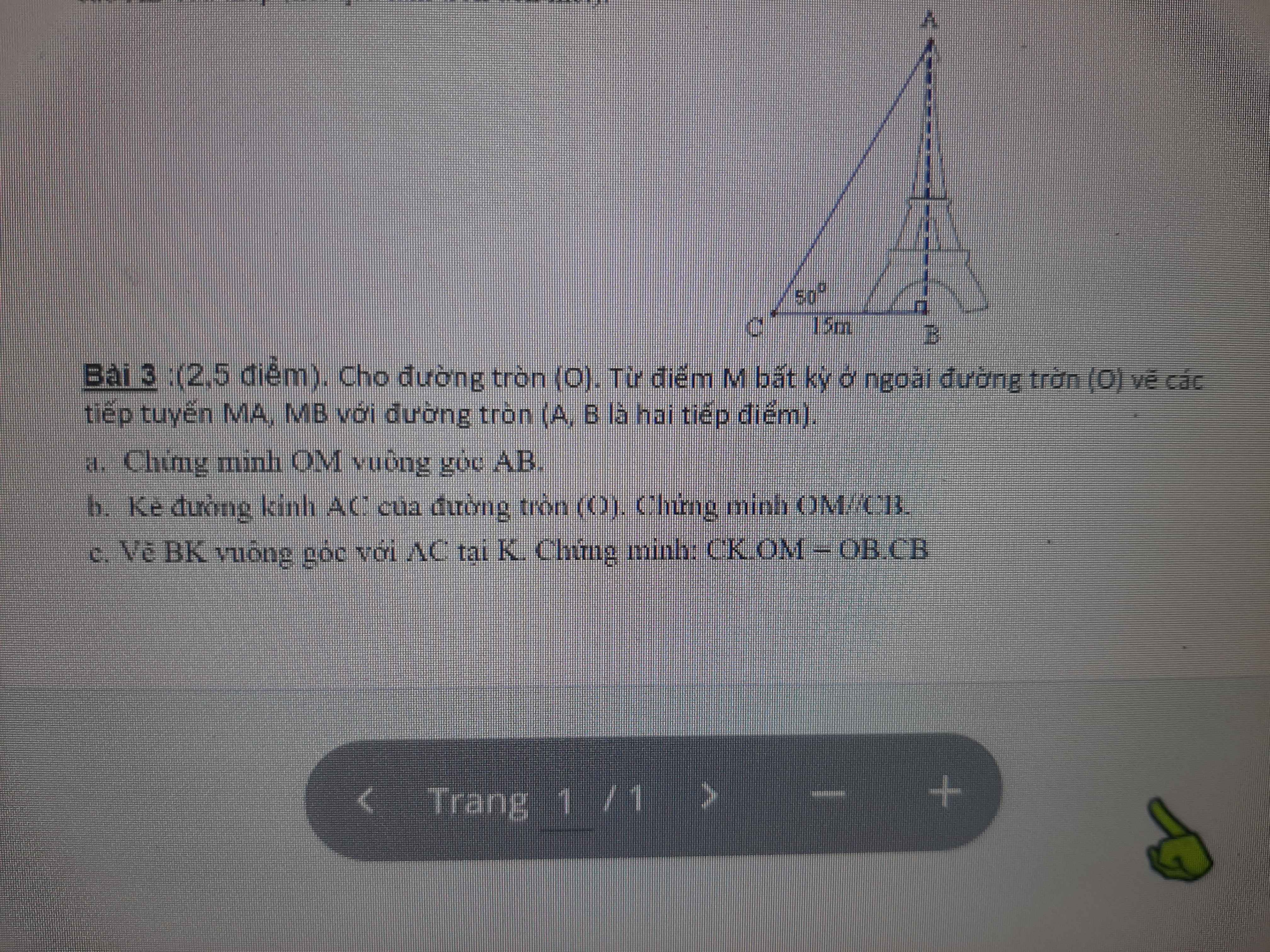


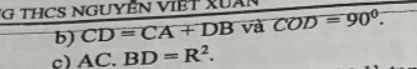 Vẽ hình và làm giúp em bài này với ạ, Em cảm ơn ạ
Vẽ hình và làm giúp em bài này với ạ, Em cảm ơn ạ







