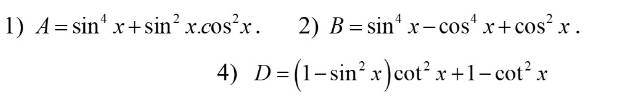1) \(A=sin^4x+sin^2x.cos^2x\)
\(\Leftrightarrow A=sin^2x.\left(sin^2x+cos^2x\right)\)
\(\Leftrightarrow A=sin^2x.1=sin^2x\)
2) \(B=sin^4x-cos^4x+cos^2x\)
\(\Leftrightarrow B=\left(sin^2x-cos^2x\right)\left(sin^2x+cos^2x\right)+cos^2x\)
\(\Leftrightarrow B=sin^2x-cos^2x+cos^2x\)
\(\Leftrightarrow B=sin^2x\)
4) \(D=\left(1-sin^2x\right)cot^2x+1-cot^2x\)
\(\Leftrightarrow D=cot^2x-sin^2xcot^2x+1-cot^2x\)
\(\Leftrightarrow D=-cos^2x+1\)
\(\Leftrightarrow D=sin^2x\)
Đúng 4
Bình luận (0)