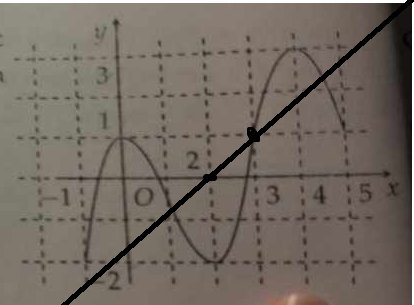
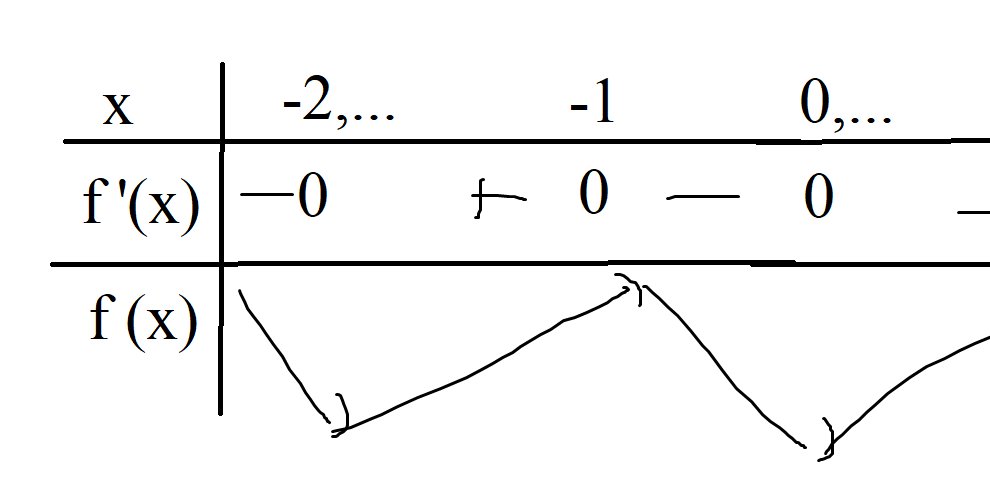
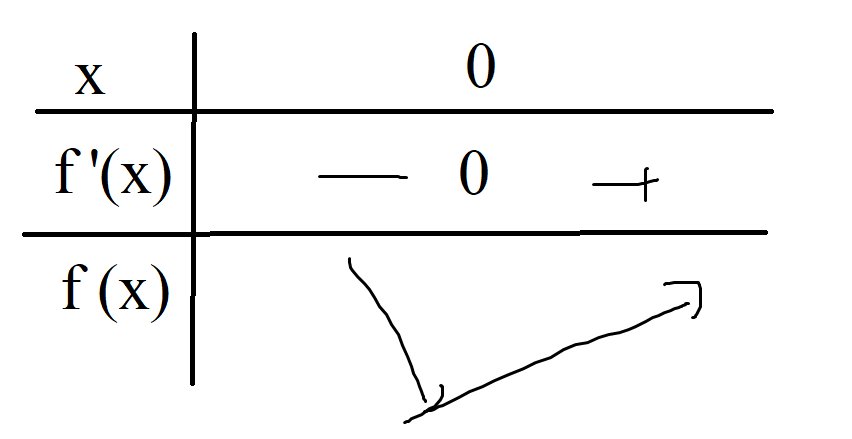
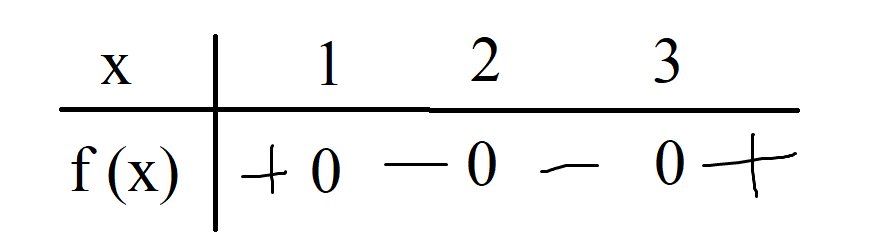Học tại trường
Chưa có thông tin
Đến từ
Đà Nẵng , Chưa có thông tin
Số lượng câu hỏi
0
Số lượng câu trả lời
820
Điểm GP
465
Điểm SP
966