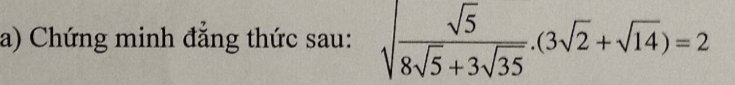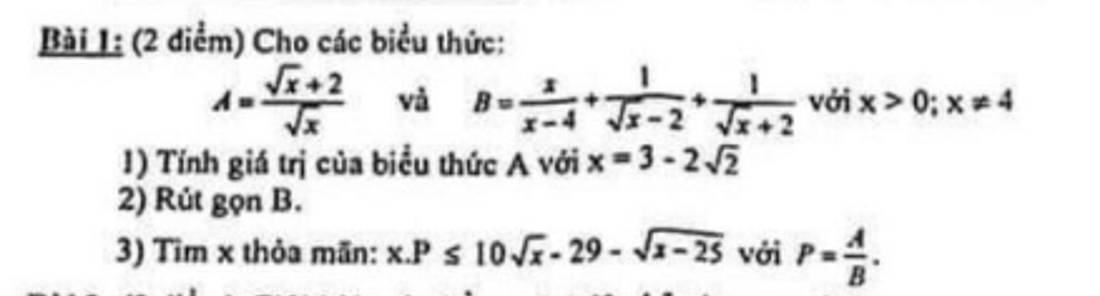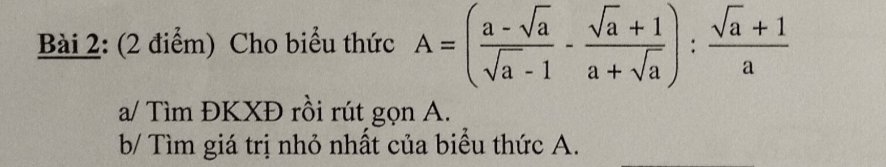Câu I:
1. \(P=\dfrac{\sqrt{x}}{\sqrt{x}-1}-\dfrac{2\sqrt{x}}{\sqrt{x}+1}+\dfrac{x-3}{x-1}\left(x\ge0;x\ne1\right)\)
\(=\dfrac{\sqrt{x}\left(\sqrt{x}+1\right)}{x-1}-\dfrac{2\sqrt{x}\left(\sqrt{x}-1\right)}{x-1}+\dfrac{x-3}{x-1}\)
\(=\dfrac{x+\sqrt{x}-2x+2\sqrt{x}+x-3}{x-1}\)
\(=\dfrac{3\sqrt{x}-3}{x-1}=\dfrac{3\left(\sqrt{x}-1\right)}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}=\dfrac{3}{\sqrt{x}+1}\)
2. \(\dfrac{1}{P}=\dfrac{4}{3}\)
\(\Leftrightarrow\dfrac{1}{\dfrac{3}{\sqrt{x}+1}}=\dfrac{4}{3}\)
\(\Leftrightarrow\dfrac{12}{\sqrt{x}+1}=3\)
\(\Leftrightarrow3\sqrt{x}+3=12\)
\(\Leftrightarrow\sqrt{x}=3\)
\(\Leftrightarrow x=9\left(Vì.x\ge0;x\ne1\right)\)
Câu II:
1. Vì đường thẳng (d) cắt trục hoành tại điểm có hoành độ bằng 2, nên đường thẳng (d) cắt trục hoành tại điểm có tọa độ (2;0)
Thay x = 2; y = 0 vào phương trình đường thẳng (d), ta được:
\(0=\left(2-m\right).2+m+1\)
\(\Leftrightarrow4-2m+m+1=0\)
\(\Leftrightarrow-m=-5\)
\(\Leftrightarrow m=5\)
Vậy nếu m = 5 thì đưởng thẳng (d) cắt trục hoành tại điểm có hoành độ bằng 2.
2. \(\left\{{}\begin{matrix}3x+2y=11\\x-2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4x=12\\x-2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\3-2y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=1\end{matrix}\right.\)
Vậy hệ phương trình có nghiệm duy nhất là (x; y) = (3; 1)
Câu III:
1. (Anh làm theo cách nhanh nhất thôi em nhé)
\(-x^2+4x-3=0\)
\(\Leftrightarrow x^2-4x+3=0\)
\(\Leftrightarrow x^2-x-3x+3=0\)
\(\Leftrightarrow x\left(x-1\right)-3\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-3\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\x-1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=1\end{matrix}\right.\)
vậy phương trình có 2 nghiệm \(S=\left\{3;1\right\}\)
(Phần 2 anh không thấy rõ đề em nhé)





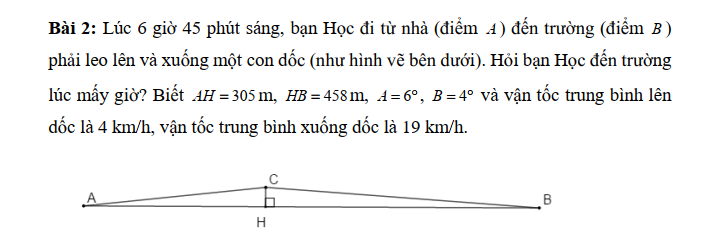
 giúp e bài 3b và bài 6 vs ạ
giúp e bài 3b và bài 6 vs ạ