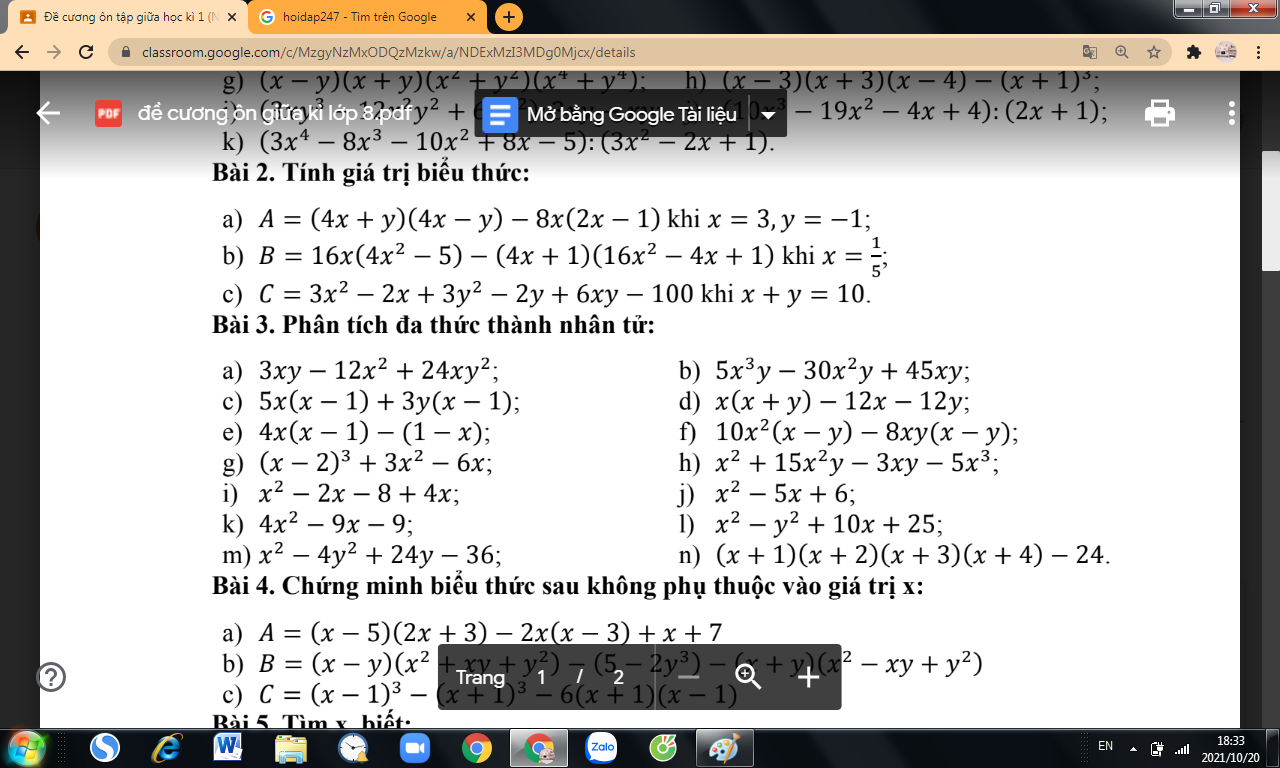
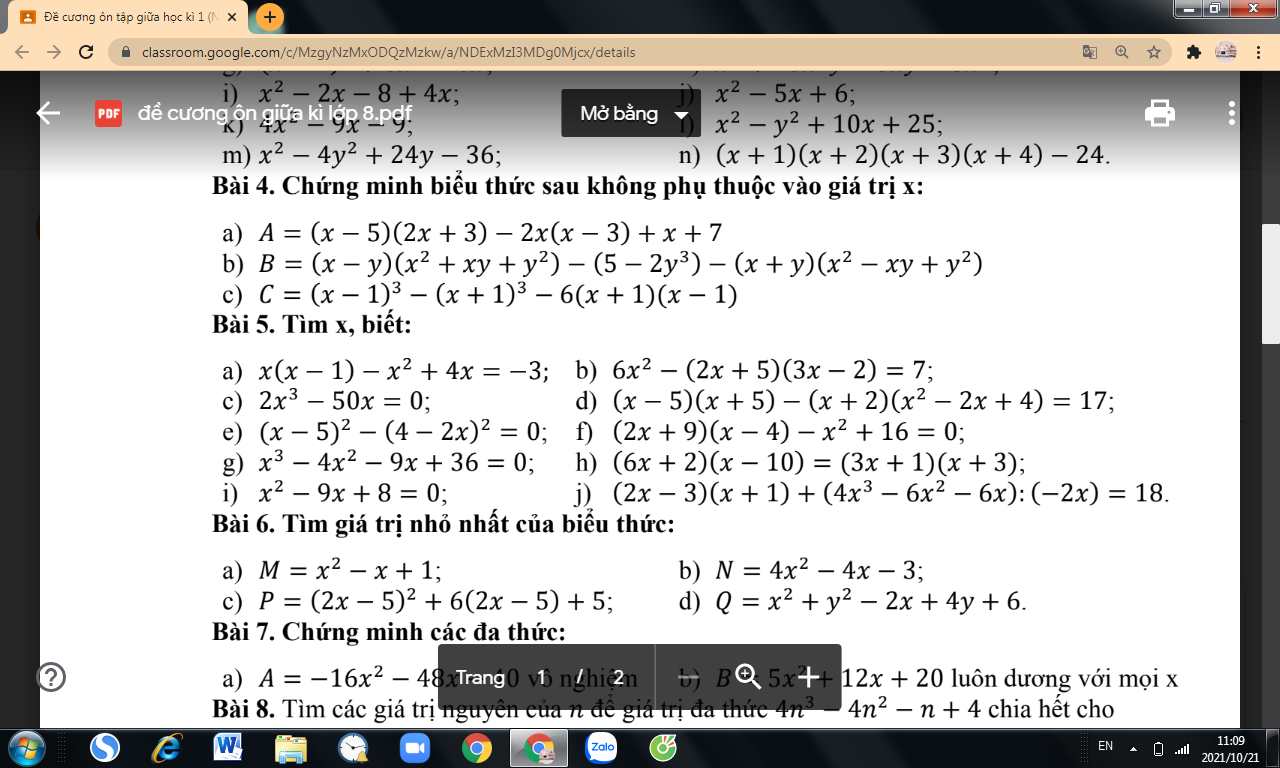
1. Thực hiện phép tính
\(2x.\left(3x^2-2\right)\)
\(=2x.3x^2+2x.\left(-2\right)\)
\(=6x^3-4x\)
\(\left(2x^2-2x+3\right).\left(x-2\right)\)
\(=x.\left(2x^2-2x+3\right)-2.\left(2x^2-2x+3\right)\)
\(=2x^3-2x^2+3x-4x^2+4x-6\)
\(=2x^3-\left(2x^2+4x^2\right)+\left(3x+4x\right)-6\)
\(=2x^3-6x^2+7x-6\)
\(\left(x-3\right)^2\)
\(=x^2-2x.3+3^2\)
\(=x^2-6x+9\)
2. Phân tích đa thức thành nhân tử:
\(x^2-y^2\)
\(=\left(x-y\right).\left(x+y\right)\)
\(7x^3-7x\)
\(=7x.\left(x^2-1\right)\)
\(=7x.\left(x-1\right).\left(x+1\right)\)
\(x^2-xy+x-y\)
\(=x^2+x-xy-y\)
\(=x.\left(x+1\right)-y.\left(x+1\right)\)
\(=\left(x-y\right).\left(x+1\right)\)
\(x^2-8x+7\)
\(=x^2-x-7x+7\)
\(=x.\left(x-1\right)-7.\left(x-1\right)\)
\(=\left(x-7\right).\left(x-1\right)\)
3. Rút gọn rồi tính giá trị biểu thức
\(x.\left(5-2x\right)-2x.\left(1-x\right)\)
\(=5x-2x^2-2x+2x^2\)
\(=\left(5x-2x\right)+\left(2x^2-2x^2\right)\)
\(=3x\)
Thay vào ta được
\(3.\frac{2}{3}=2\)
Vậy tại \(x=\frac{2}{3}\) thì giá trị của biểu thức là \(2\)
4. Chứng minh biểu thức sau không phụ thuộc vào biến x
\(2x.\left(3-x\right)-\left(5-x\right).\left(2x+4\right)\)
\(=6x-2x^2-5.\left(2x+4\right)+x.\left(2x+4\right)\)
\(=6x-2x^2-10x-20+2x^2+4x\)
\(=\left(-2x^2+2x^2\right)+\left(6x-10x+4x\right)-20\)
\(=-20\)
Vậy biểu thức trên không phụ thuộc vào biến \(x\)
giúp em với mn ơi,e đag cần gấp, mn giúp em với ạ
Mn giúp em bài 3 câu bc với em tính số xấu quá, mong mn giúp ạ:(
giúp em với ạ, em đang vội mong mn giúp đỡ
