a) ĐKXĐ: \(x>0;x\ne\pm1.\)
\(A=\left(\dfrac{\sqrt{x}+1}{\sqrt{x}-1}+\dfrac{\sqrt{x}}{\sqrt{x}+1}+\dfrac{\sqrt{x}}{1-x}\right):\left(\dfrac{\sqrt{x}+1}{\sqrt{x}-1}+\dfrac{1-\sqrt{x}}{\sqrt{x}+1}\right).\\ A=\dfrac{x+2\sqrt{x}+1+x-\sqrt{x}-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}:\dfrac{x+2\sqrt{x}+1-x+2\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}.\)
\(A=\dfrac{2x+1}{4\sqrt{x}}.\)
b) \(A=\dfrac{3}{4}.\Rightarrow\dfrac{2x+1}{4\sqrt{x}}=\dfrac{3}{4}.\Rightarrow12\sqrt{x}-8x+4=0.\\ \Leftrightarrow8x-12\sqrt{x}-4=0.\Leftrightarrow\left[{}\begin{matrix}\sqrt{x}=\dfrac{3+\sqrt{17}}{4}.\\\sqrt{x}=\dfrac{3-\sqrt{17}}{4}.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{13+3\sqrt{17}}{8}.\\x=\dfrac{13-3\sqrt{17}}{8}.\end{matrix}\right.\) (TM).


















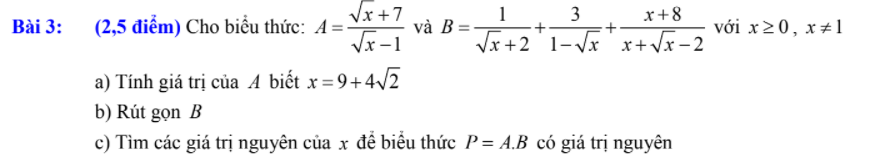 được không ạ, mai em phải nộp rồi, cảm ơn mn nhiều.
được không ạ, mai em phải nộp rồi, cảm ơn mn nhiều. 