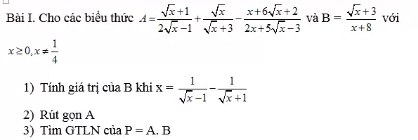Câu này tương đối lằng nhằng khi lấy biểu thức A nhân với biểu thức B
Vì rút gọn A thì biểu thức của A siêu lằng nhằng
Để làm được câu c thì phải qua câu b trước đã
Bài 1: 2)\(A=\dfrac{\sqrt{x}+1}{2\sqrt{x}-1}+\dfrac{\sqrt{x}}{\sqrt{x}+3}-\dfrac{x+6\sqrt{x}+2}{2x+5\sqrt{x}-3}\)
\(=\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}+3\right)+\left(2\sqrt{x}-1\right)\sqrt{x}}{\left(2\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}-\dfrac{\sqrt{x}^2+6\sqrt{x}+2}{2x-\sqrt{x}+6\sqrt{x}-3}\\ =\dfrac{\sqrt{x}^2+3\sqrt{x}+\sqrt{x}+3+2\sqrt{x}^2-\sqrt{x}-\sqrt{x}^2-6\sqrt{x}-2}{\left(2\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\\ =\dfrac{2\sqrt{x}^2-3\sqrt{x}+1}{\left(2\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\\ =\dfrac{2\sqrt{x}^2-2\sqrt{x}-\sqrt{x}+1}{\left(2\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\\ =\dfrac{2\sqrt{x}\left(\sqrt{x}-1\right)-\left(\sqrt{x}-1\right)}{\left(2\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\\ =\dfrac{\left(2\sqrt{x}-1\right)\left(\sqrt{x}-1\right)}{\left(2\sqrt{x}-1\right)\left(\sqrt{x}+3\right)}\\ =\dfrac{\sqrt{x}-1}{\sqrt{x}+3}\)
⇒\(P=A\cdot B=\dfrac{\sqrt{x}-1}{\sqrt{x}+3}\cdot\dfrac{\sqrt{x}+3}{x+8}=\dfrac{\sqrt{x}-1}{x+8}\)