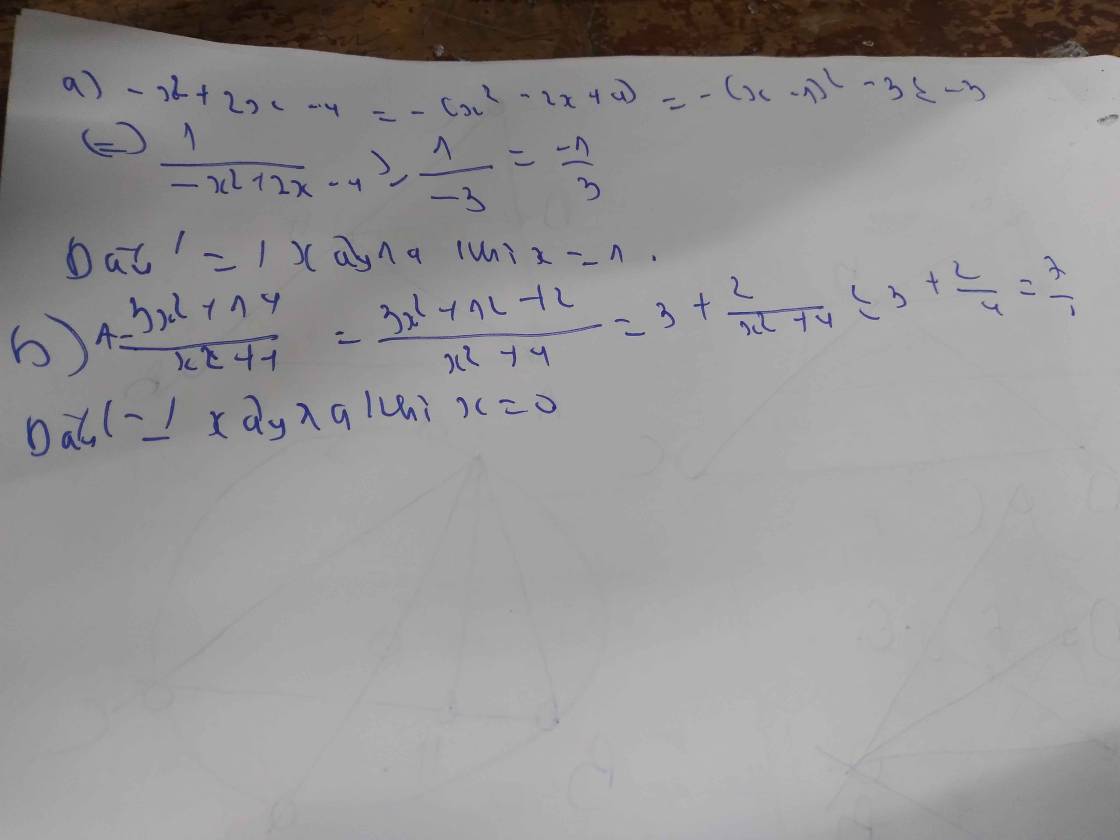Bài 1: Nhân đơn thức với đa thức
Các câu hỏi tương tự
1/ Thực hiện phép nhân :
a) x2 ( 5x3 - x - \(\dfrac{1}{2}\))
b) ( 3xy - x2 + y ) \(\dfrac{2}{3}\)x2y
c) x2 ( 4x3 - 5xy + 2x ) ( -\(\dfrac{1}{2}\) xy )
2/ Tìm x, biết
a) 3x( 12x - 4 ) - 9x (4x - 3 ) = 30
b ) x( 5 - 2x ) + 2x ( x - 1 )= 15
Rút gọn:
\(A=\left(\dfrac{1+2x}{4+2x}-\dfrac{x}{3x-6}+\dfrac{2x^2}{12-3x^2}\right)\cdot\dfrac{24-12x}{6+13x}\)
Bài 1: Tính giá trị của biểu thức
dfrac{3}{4} xy2(x2 + dfrac{2}{3} xy + dfrac{4}{3} y2) - dfrac{1}{2}xy (-dfrac{1}{2}x^2y + xy2 + y3) tại x dfrac{1}{2} , y 2
Bài 2: Chứng minh đẳng thức
a) 5x(x2 + 2x - 1) - 3x2 ( x - 2) x(2x2 - 1) + 4x(4x -1)
b) xy(2x3 - 3y3) - x2y2(5x + 4y) 2x2y(x2 - xy + y2) - 3xy2(x2 + 2xy + y2)
c) 2y(x3 + x2y - dfrac{1}{4} y3) - dfrac{1}{2}x(2x3 + 4xy2 - y3) dfrac{1}{2}y3(x - y) - x3(x - 2y)
Bài 3: Tìm x thỏa mãn điều kiện
a) 6x(x - 4) + 2x(2 - 3x) -25
b) 5x2(...
Đọc tiếp
Bài 1: Tính giá trị của biểu thức
\(\dfrac{3}{4}\) xy2(x2 + \(\dfrac{2}{3}\) xy + \(\dfrac{4}{3}\) y2) - \(\dfrac{1}{2}xy\) (-\(\dfrac{1}{2}x^2y\) + xy2 + y3) tại x = \(\dfrac{1}{2}\) , y = 2
Bài 2: Chứng minh đẳng thức
a) 5x(x2 + 2x - 1) - 3x2 ( x - 2) = x(2x2 - 1) + 4x(4x -1)
b) xy(2x3 - 3y3) - x2y2(5x + 4y) = 2x2y(x2 - xy + y2) - 3xy2(x2 + 2xy + y2)
c) 2y(x3 + x2y - \(\dfrac{1}{4}\) y3) - \(\dfrac{1}{2}\)x(2x3 + 4xy2 - y3) = \(\dfrac{1}{2}\)y3(x - y) - x3(x - 2y)
Bài 3: Tìm x thỏa mãn điều kiện
a) 6x(x - 4) + 2x(2 - 3x) = -25
b) 5x2(3x - 2) - 3x2(5x + 2) + 2x(3 + 8x) = 21
c) 5x(4x2 - 2x + 1) - 2x(10x2 - 5x - 2) = -36
Thực hiện phép tính:
a, (2x-y)(4x2+2x+y2)
b, (43y2-6x2y3+12x3y3):2x2y2
c, \(\dfrac{3x}{2x-2y}\):\(\dfrac{x^2}{x-y}\)
d, \(\dfrac{x}{x-1}\)+\(\dfrac{2}{1-x}\)\(\dfrac{x+1}{x^2-1}\)
-2,5ab.(-2a2+3b2) =...............................
-2x3(3x+0,5x2-7x3-2)=.........................
(x3-2x2+3x-5)(-xy)=..............................
(\(\dfrac{-1}{2}\)x2y)(3x3-\(\dfrac{2}{7}\)x2-\(\dfrac{4}{5}\)x+8)=.......................
Thực hiện phép tính , rút gọn bt
\(\dfrac{2x+y}{2x^2-xy}+\dfrac{16x}{y^2-4x^2}+\dfrac{2x-y}{2x^2+xy}\)
\(\dfrac{x+y}{2\left(x-y\right)}+\dfrac{2}{x^2+3}+\dfrac{1}{x+1}\)
Tìm x, biết
a) 4(x+2) - 7(2x-1) + 9(3x-4) = 30
b)2(5x-8) - 3(4x-5) = 4(3x-4) + 11
c) 4x2 + 3(2x2+1) = 2x(5x-7)
d) x(x2-7) = 2x(\(\dfrac{1}{2}\)x2+6) + 8
\(\left(5x^3-2x^2+\dfrac{1}{3}x-2\right).\left(\dfrac{-3}{2}x^3+\dfrac{1}{2}x^4\right)\)
Rút gọn các biểu thức sau :
a) \(x\left(2x^2-3\right)-x^2\left(5x+1\right)+x^2\)
b) \(3x\left(c-2\right)-5x\left(1-x\right)-8\left(x^2-3\right)\)
c) \(\dfrac{1}{2}x^2\left(6x-3\right)-x\left(x^2+\dfrac{1}{2}\right)+\dfrac{1}{2}\left(x+4\right)\)