Giải phương trình sau: tan3x.tanx = 1

Những câu hỏi liên quan
Dùng cung liên kết giải các phương trình sau:
a) Cos6x +3Cos2x = \(\sqrt{2}\)
b) Sin2x - Cos23x = 0
c) Tan3x.tanx = 1
d) Sin3x.Cos5x = Sinx.Cos3x
a.
\(\Leftrightarrow4cos^32x-3cos2x+3cos2x=\sqrt{2}\)
\(\Leftrightarrow cos^32x=\dfrac{\sqrt{2}}{4}\)
\(\Leftrightarrow cos2x=\dfrac{\sqrt{2}}{2}\)
\(\Leftrightarrow2x=\pm\dfrac{\pi}{4}+k2\pi\)
\(\Leftrightarrow x=\pm\dfrac{\pi}{8}+k\pi\) (\(k\in Z\))
Đúng 1
Bình luận (0)
c.
ĐKXĐ: \(\left\{{}\begin{matrix}cos3x\ne0\\cosx\ne0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x\ne\dfrac{\pi}{6}+\dfrac{k\pi}{3}\\x\ne\dfrac{\pi}{2}+k\pi\end{matrix}\right.\)
\(tan3x.tanx=1\)
\(\Rightarrow tan3x=\dfrac{1}{tanx}\)
\(\Rightarrow tan3x=cotx\)
\(\Rightarrow tan3x=tan\left(\dfrac{\pi}{2}-x\right)\)
\(\Rightarrow3x=\dfrac{\pi}{2}-x+k\pi\)
\(\Rightarrow x=\dfrac{\pi}{8}+\dfrac{k\pi}{4}\)
Đúng 1
Bình luận (0)
b.
\(\Leftrightarrow\dfrac{1}{2}-\dfrac{1}{2}cos2x-\left(\dfrac{1}{2}+\dfrac{1}{2}cos6x\right)=0\)
\(\Leftrightarrow cos6x=-cos2x\)
\(\Leftrightarrow cos6x=cos\left(\pi-2x\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}6x=\pi-2x+k2\pi\\6x=2x-\pi+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}8x=\pi+k2\pi\\4x=-\pi+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{8}+\dfrac{k\pi}{4}\\x=-\dfrac{\pi}{4}+\dfrac{k\pi}{2}\end{matrix}\right.\)
(\(k\in Z\))
Đúng 0
Bình luận (0)
Xem thêm câu trả lời
giải phương trình tan3x.tanx = 1
ĐKXĐ: \(\left\{{}\begin{matrix}cos3x\ne0\\cosx\ne0\end{matrix}\right.\)
\(\Leftrightarrow\frac{sin3x.sinx}{cos3x.cosx}=1\)
\(\Leftrightarrow sin3x.sinx-cos3x.cosx=0\)
\(\Leftrightarrow sin2x=0\)
\(\Leftrightarrow2sinx.cosx=0\)
\(\Leftrightarrow sinx=0\Leftrightarrow x=k\pi\)
Giải các phương trình
a) \(\dfrac{\cos2x}{\sin2x-1}=0\)
b) \(\cos\left(\sin x\right)=1\)
c) \(2\sin^2x-1+\cos3x=0\)
d) \(tan3x.tanx=1\)
e) \(\cos3x=-\cos7x\)
a: ĐKXĐ: sin 2x<>1
=>2x<>pi/2+k2pi
=>x<>pi/4+kpi
\(\dfrac{cos2x}{sin2x-1}=0\)
=>cos2x=0
=>2x=pi/2+kpi
=>x=pi/4+kpi/2
Kết hợp ĐKXĐ, ta được:
x=3/4pi+k2pi hoặc x=7/4pi+k2pi
b: cos(sinx)=1
=>sin x=kpi
=>sin x=0
=>x=kpi
c: \(2\cdot sin^2x-1+cos3x=0\)
=>cos3x+cos2x=0
=>cos3x=-cos2x=-sin(pi/2-2x)=sin(2x-pi/2)
=>cos3x=cos(pi/2-2x+pi/2)=cos(pi-2x)
=>3x=pi-2x+k2pi hoặc 3x=-pi+2x+k2pi
=>x=-pi+k2pi hoặc x=pi/5+k2pi/5
e: cos3x=-cos7x
=>cos3x=cos(pi-7x)
=>3x=pi-7x+k2pi hoặc 3x=-pi+7x+k2pi
=>x=pi/10+kpi/5 hoặc x=pi/4-kpi/2
Đúng 2
Bình luận (0)
Tìm nghiệm của phương trình: \(tan3x.tanx=1\) trên đoạn \(\left[0;2\pi\right]\)
Nhận thấy tanx=0 không phải nghiệm
\(\Leftrightarrow tan3x=\frac{1}{tanx}\)
\(\Leftrightarrow tan3x=cotx=tan\left(\frac{\pi}{2}-x\right)\)
\(\Rightarrow3x=\frac{\pi}{2}-x+k\pi\)
\(\Rightarrow x=\frac{\pi}{8}+\frac{k\pi}{4}\)
\(\Rightarrow x=\frac{\pi}{8}+\frac{k\pi}{4}\) với \(k=\left\{0;1;2...;7\right\}\)
Đúng 0
Bình luận (0)
Bài 1: Giải các bất phương trình và phương trình sau :
a) 2(3-4x) = 10-(2x – 5)
Giải các bất phương trình và phương trình sau :
a) 3(2-4x) = 11-(3x – 1)
Bài 1:
a) Ta có: \(2\left(3-4x\right)=10-\left(2x-5\right)\)
\(\Leftrightarrow6-8x-10+2x-5=0\)
\(\Leftrightarrow-6x+11=0\)
\(\Leftrightarrow-6x=-11\)
hay \(x=\dfrac{11}{6}\)
b) Ta có: \(3\left(2-4x\right)=11-\left(3x-1\right)\)
\(\Leftrightarrow6-12x-11+3x-1=0\)
\(\Leftrightarrow-9x-6=0\)
\(\Leftrightarrow-9x=6\)
hay \(x=-\dfrac{2}{3}\)
Đúng 2
Bình luận (0)
Bài 1: Giải phương trình và hệ phương trình sau:

cho phương trình sau: x² - 2(m+1) -m - 1=0 a, Giải phương trình trên khi m=2 b, không giải phương trình tính giá trị biểu thức c,tìm giá trị nhỏ nhất của phương trình tại m=4
a: Khi m=2 thì pt sẽ là x^2-6x-3=0
=>\(x=3\pm2\sqrt{3}\)
Đúng 0
Bình luận (0)
1.Giải các phương trình sau : a,7x+35=0 b, 8-x/x-7 -8 =1/x-7 2.giải bất phương trình sau : 18-3x(1-x)_< 3x^2-3x
a: 7x+35=0
=>7x=-35
=>x=-5
b: \(\dfrac{8-x}{x-7}-8=\dfrac{1}{x-7}\)
=>8-x-8(x-7)=1
=>8-x-8x+56=1
=>-9x+64=1
=>-9x=-63
hay x=7(loại)
Đúng 1
Bình luận (0)
a, \(7x=-35\Leftrightarrow x=-5\)
b, đk : x khác 7
\(8-x-8x+56=1\Leftrightarrow-9x=-63\Leftrightarrow x=7\left(ktm\right)\)
vậy pt vô nghiệm
2, thiếu đề
Đúng 0
Bình luận (0)
1.
\(a,7x+35=0\\ \Rightarrow7x=-35\\ \Rightarrow x=-5\\ b,ĐKXĐ:x\ne7\\ \dfrac{8-x}{x-7}-8=\dfrac{1}{x-7}\\ \Leftrightarrow\dfrac{8-x}{x-7}-\dfrac{8\left(x-7\right)}{x-7}-\dfrac{1}{x-7}=0\\ \Leftrightarrow\dfrac{8-x-8x+56-1}{x-7}=0\\ \Rightarrow-9x+63=0\\ \Leftrightarrow-9x=-63\\ \Leftrightarrow x=7\left(ktm\right)\)
2.đề thiếu
Đúng 0
Bình luận (0)
Giải phương trình sau bằng cách đưa về phương trình tích: 4x2 – 1 = (2x + 1)(3x – 5)
4x2 – 1 = (2x + 1)(3x – 5)
⇔ 4x2 – 1 – (2x + 1)(3x – 5) = 0
⇔ (2x – 1)(2x + 1) – (2x + 1)(3x – 5) = 0
⇔ (2x + 1)[(2x – 1) – (3x – 5)] = 0
⇔ (2x + 1)(2x – 1 – 3x + 5) = 0
⇔ (2x + 1)(4 – x) = 0
⇔ 2x + 1= 0 hoặc 4 – x = 0
+ 2x + 1 = 0 ⇔ 2x = -1 ⇔ x = -1/2.
+ 4 – x = 0 ⇔ x = 4.
Vậy phương trình có tập nghiệm 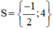
Đúng 0
Bình luận (0)





















