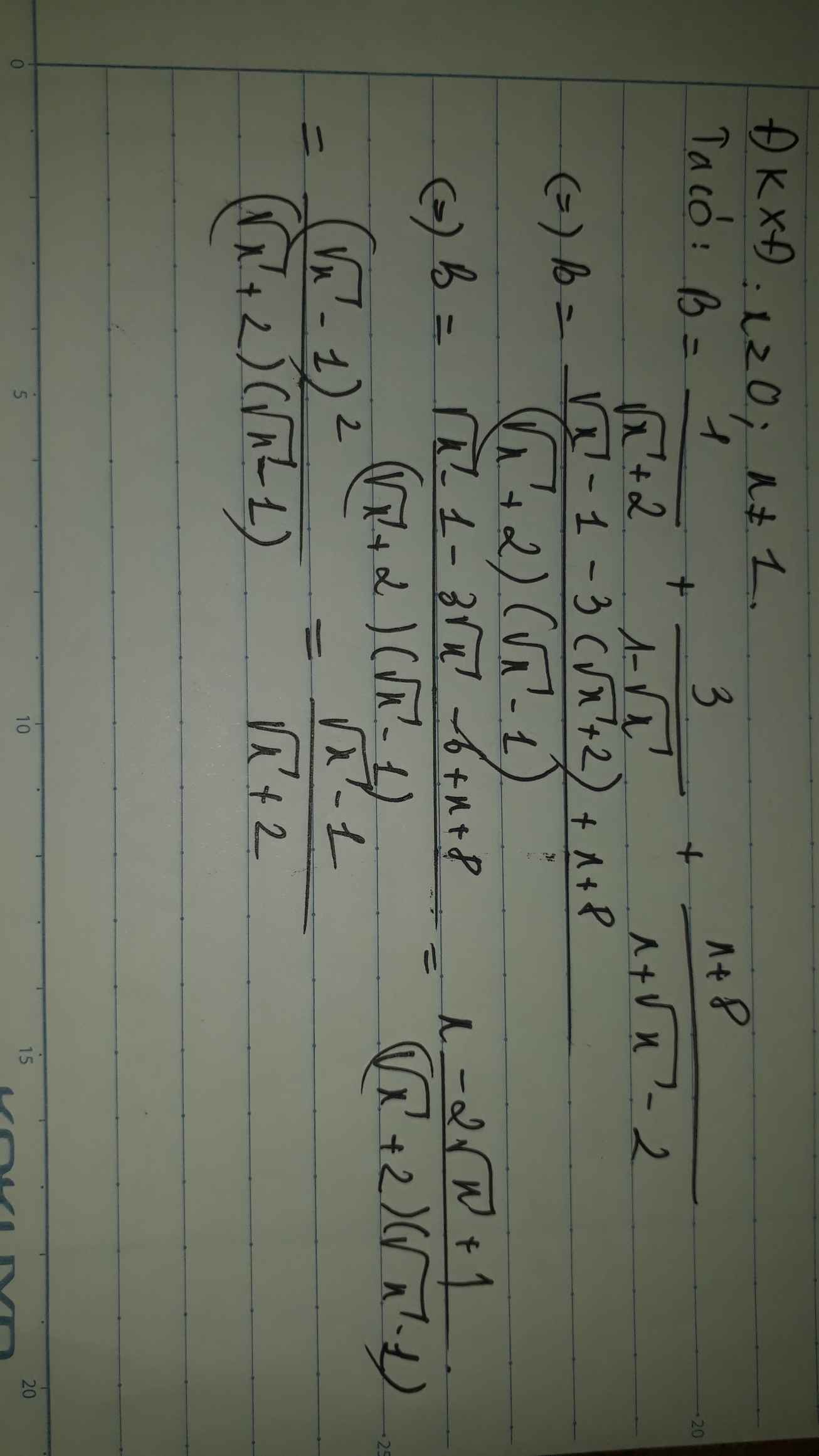ĐK: \(x\ge0;x\ne1\)
\(B=\dfrac{1}{\sqrt{x}+2}+\dfrac{3}{1-\sqrt{x}}+\dfrac{x+8}{x+\sqrt{x}-2}\)
\(=\dfrac{\sqrt{x}-1}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}-\dfrac{3\sqrt{x}+6}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}+\dfrac{x+8}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{x-2\sqrt{x}+1}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}=\dfrac{\sqrt{x}-1}{\sqrt{x}+2}\)
Ta có: \(B=\dfrac{1}{\sqrt{x}+2}+\dfrac{3}{1-\sqrt{x}}+\dfrac{x+8}{x+\sqrt{x}-2}\)
\(=\dfrac{\sqrt{x}-1-3\sqrt{x}-6+x+8}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{x-2\sqrt{x}+1}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}\)
\(=\dfrac{\sqrt{x}-1}{\sqrt{x}+2}\)