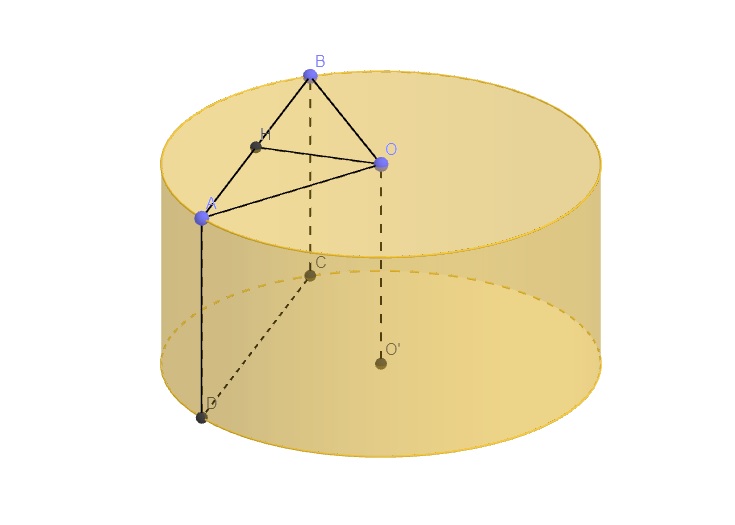
Gọi H là trung điểm AB \(\Rightarrow OH\perp AB\Rightarrow OH\perp\left(ABCD\right)\)
\(\Rightarrow V_{O.ABCD}=\dfrac{1}{3}OH.S_{ABCD}\)
Đặt \(OH=x\Rightarrow BH=\sqrt{R^2-OH^2}=\sqrt{9a^2-x^2}\)
\(\Rightarrow AB=2BH=2\sqrt{9a^2-x^2}\)
\(\Rightarrow V=\dfrac{1}{3}x.3a.2\sqrt{9a^2-x^2}=a.2x.\sqrt{9a^2-x^2}\le a\left(x^2+9a^2-x^2\right)=9a^3\)
\(\Rightarrow V_{max}=9a^3\)