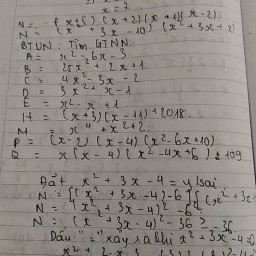Question

a,\(|x\left(x-4\right)|=x\)

b,\(|x+2|-6x=1\)

c, \(|x^2-2x|=x\)

Answer 1

\(a,\left|x\left(x-4\right)\right|=x\)

ĐKXĐ : \(x>0\)

=> \(\left|x^2-4x\right|=x\)

=> \(\left[{}\begin{matrix}x^2-4x=x\\x^2-4x=-x\end{matrix}\right.\)

=> \(\left[{}\begin{matrix}x^2-4x-x=0\\x^2-4x+x=0\end{matrix}\right.\)

=> \(\left[{}\begin{matrix}x^2-5x=0\\x^2-3x=0\end{matrix}\right.\)

=> \(\left[{}\begin{matrix}x.\left(x-5\right)=0\\x\left(x-3\right)=0\end{matrix}\right.\)

=> \(\left\{{}\begin{matrix}\left[{}\begin{matrix}x=0\\x-5=0=>x=5\end{matrix}\right.\\\left[{}\begin{matrix}x=0\\x-3=0=>x=3\end{matrix}\right.\end{matrix}\right.\)

Vậy \(x\in\left\{0;5;3\right\}\)

\(b,\left|x+2\right|-6x=1\)

=> \(\left|x+2\right|=1+6x\)

=> \(\left[{}\begin{matrix}x+2=1+6x\\x+2=-\left(1+6x\right)=-1-6x\end{matrix}\right.\)

=> \(\left[{}\begin{matrix}6x-x=2-1\\6x+x=-1-2\end{matrix}\right.\)

=> \(\left[{}\begin{matrix}5x=1\\7x=-3\end{matrix}\right.\)

=> \(\left[{}\begin{matrix}x=1:5=\frac{1}{5}\\x=-3:7=-\frac{3}{7}\end{matrix}\right.\)

Vậy \(x\in\left\{-\frac{3}{7};\frac{1}{5}\right\}\)

\(c,\left|x^2-2x\right|=x\)

=> \(\left[{}\begin{matrix}x^2-2x=x\\x^2-2x=-x\end{matrix}\right.\)

=> \(\left[{}\begin{matrix}x^2-2x-x=0\\x^2-2x+x=0\end{matrix}\right.\)

=> \(\left[{}\begin{matrix}x^2-3x=0\\x^2-x=0\end{matrix}\right.\)

=> \(\left[{}\begin{matrix}x\left(x-3\right)=0\\x\left(x-1\right)=0\end{matrix}\right.\)

=> \(\left\{{}\begin{matrix}\left[{}\begin{matrix}x=0\\x-3=0=>x=3\end{matrix}\right.\\\left[{}\begin{matrix}x=0\\x-1=0=>x=1\end{matrix}\right.\end{matrix}\right.\)

vậy \(x\in\left\{0;1;3\right\}\)

Answer 2

sửa sai tí T^T

\(b,\)ĐKXĐ : \(x>0\)

nên trường hợp \(x=-\frac{3}{7}\left(loại\right)\)

câu c thêm , ĐKXĐ : x>0

VỘI QUÁ NÊN => ........... ( là đó) Y^Y