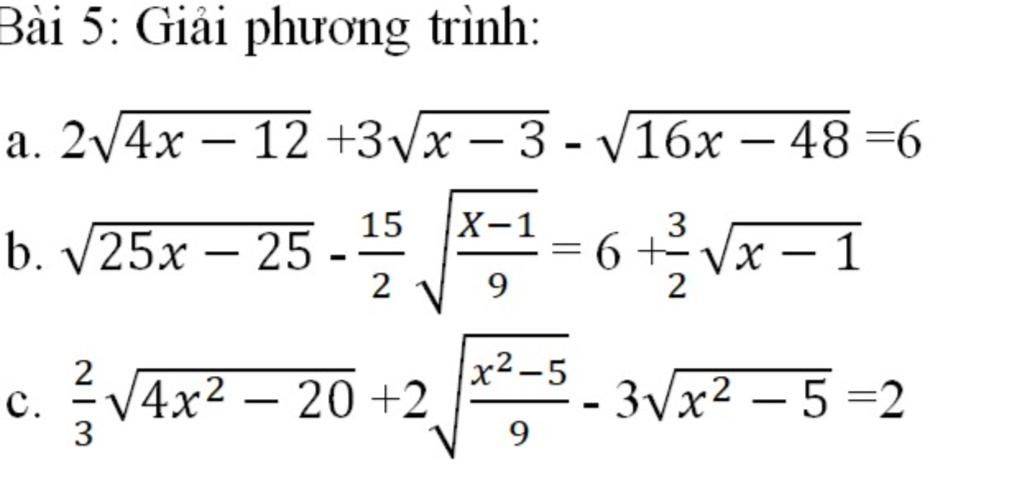
a: \(\Leftrightarrow2\cdot2\sqrt{x-3}+3\sqrt{x-3}-4\sqrt{x-3}=6\)
=>\(3\sqrt{x-3}=6\)
=>\(\sqrt{x-3}=2\)
=>x-3=4
=>x=7
b; \(\Leftrightarrow5\sqrt{x-1}-\dfrac{15}{2}\cdot\dfrac{1}{3}\sqrt{x-1}-\dfrac{3}{2}\sqrt{x-1}=6\)
=>\(\sqrt{x-1}=6\)
=>x-1=36
=>x=37
c: \(\Leftrightarrow2\sqrt{x^2-5}\cdot\dfrac{2}{3}+\dfrac{2}{3}\sqrt{x^2-5}-3\sqrt{x^2-5}=2\)
=>\(-\sqrt{x^2-5}=2\)(vô lý)
Vậy: \(x\in\varnothing\)
a.
ĐK: \(x\ge3\)
PT trở thành:
\(2\sqrt{4\left(x-3\right)}+3\sqrt{x-3}-\sqrt{16\left(x-3\right)}=6\\ \Leftrightarrow4\sqrt{x-3}+3\sqrt{x-3}-4\sqrt{x-3}=6\\ \Leftrightarrow\left(4+3-4\right)\sqrt{x-3}=6\\ \Leftrightarrow\sqrt{x-3}=2\\ \Leftrightarrow x-3=2^2=4\\ \Leftrightarrow x=7\left(tm\right)\)