a: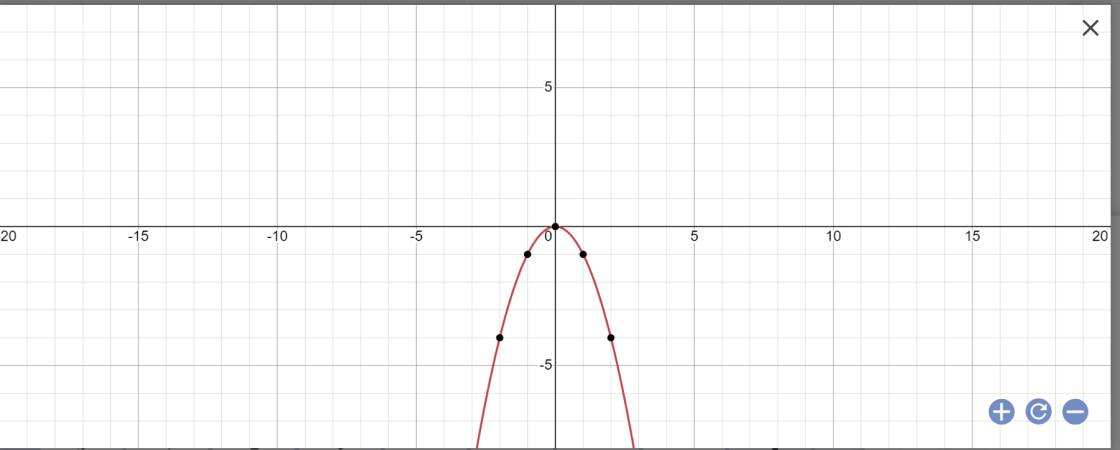 

b: PTHĐGĐ là:

-x^2=1/2x-3

=>-2x^2=x-6

=>-2x^2-x+6=0

=>2x^2+x-6=0

=>2x^2+4x-3x-6=0

=>(x+2)(2x-3)=0

=>x=3/2 hoặc x=-2

Khi x=-2 thì y=-(-2)^2=4

Khi x=3/2 thì y=-(3/2)^2=-9/4

c: Thay y=-x vào (P), ta được:

-x^2=-x

=>x^2=x

=>x(x-1)=0

=>x=0 hoặc x=1

Khi x=0 thì y=0

Khi x=1 thì y=-1

Vậy: Điểm cần tìm là M(1;-1) hoặc O(0;0)

\(\left(x-3\right)^3=x^3-9x^2+27x-27\)

\(\left(2x+\dfrac{1}{2}\right)^3=8x^3+6x^2+\dfrac{3}{2}x+\dfrac{1}{8}\)

c: Thay y=-x vào (P), ta được:

-x^2=-x

=>x^2=x

=>x(x-1)=0

=>x=0 hoặc x=1

Khi x=0 thì y=0

Khi x=1 thì y=-1

Vậy: Điểm cần tìm là M(1;-1) hoặc O(0;0)

\(\left(3x^2+\dfrac{2}{3}y\right)^2\)

\(=\left(3x^2\right)^2+2.3x.\dfrac{2}{3}y+\left(\dfrac{2}{3}y\right)^2\)

\(=9x^4+4xy+\dfrac{4}{9}y^2\)

(3x²+\(\dfrac{2}{3}\)y)²

=9\(x^4\)+\(\dfrac{4}{9}\)\(y^2\)

a) \(\left(2x+3y\right)^2=\left(2x\right)^2+2\cdot2x\cdot3y+\left(3y\right)^2=4x^2+12xy+9y^2\)

b) \(\left(x+\dfrac{1}{4}\right)^2=x^2+2\cdot x\cdot\dfrac{1}{4}+\left(\dfrac{1}{4}\right)^2=x^2+\dfrac{1}{2}x+\dfrac{1}{16}\)

c) \(\left(x^2+\dfrac{2}{5}y\right)\left(x^2-\dfrac{2}{5}y\right)=\left(x^2\right)^2-\left(\dfrac{2}{5}y\right)^2=x^4-\dfrac{4}{25}y^2\)

d) \(\left(2x+y^2\right)^3=\left(2x\right)^3+3\cdot\left(2x\right)^2\cdot y^2+3\cdot2x\cdot\left(y^2\right)^2+\left(y^2\right)^3=8x^3+12x^2y^2+6xy^4+y^6\)

e) \(\left(3x^2-2y\right)^2=\left(3x^2\right)^2-2\cdot3x^2\cdot2y+\left(2y\right)^2=9x^4-12x^2y+4y^2\)

f) \(\left(x+4\right)\left(x^2-4x+16\right)=x^3+4^3=x^3+64\)

g) \(\left(x^2-\dfrac{1}{3}\right)\cdot\left(x^4+\dfrac{1}{3}x^2+\dfrac{1}{9}\right)=\left(x^2\right)^3-\left(\dfrac{1}{3}\right)^3=x^6-\dfrac{1}{27}\)

\(A=\left(-\dfrac{2}{3}x^3y^4\right)^2.\left(-3x^5y^2\right)^3\)

\(A=\left(\dfrac{4}{9}x^6y^8\right).\left(-27x^{15}y^6\right)\)

\(A=\left(\dfrac{4}{9}.-27\right)\left(x^6.x^{15}\right)\left(y^8.y^{16}\right)\)

\(A=-12x^{21}y^{24}\)

\(\text{Hệ số:-12}\)

\(\text{Bậc:45}\)

\(B=\left(3x^2y\right).\left(-\dfrac{1}{3}x^3y\right).\left(-\dfrac{1}{4}x^3y^4\right)\)

\(B=\left(3.-\dfrac{1}{3}.-\dfrac{1}{4}\right).\left(x^2.x^3.x^3\right).\left(y.y.y^4\right)\)

\(B=\dfrac{1}{4}x^8y^6\)

\(\text{Hệ số:}\dfrac{1}{4}\)

\(\text{Bậc:14}\)

a) \(N=-1-x-x^2=-\left(x^2+x+\dfrac{1}{4}\right)-\dfrac{3}{4}=-\left(x+\dfrac{1}{2}\right)^2-\dfrac{3}{4}\le-\dfrac{3}{4}\)

\(maxN=-\dfrac{3}{4}\Leftrightarrow x=-\dfrac{1}{2}\)

b) \(B=3x^2+4x-13=3\left(x^2+\dfrac{4}{3}x+\dfrac{4}{9}\right)-\dfrac{35}{3}=3\left(x+\dfrac{2}{3}\right)^2-\dfrac{35}{3}\ge-\dfrac{35}{3}\)

\(minB=-\dfrac{35}{3}\Leftrightarrow x=-\dfrac{2}{3}\)

a: Ta có: \(N=-x^2-x-1\)

\(=-\left(x^2+x+1\right)\)

\(=-\left(x^2+2\cdot x\cdot\dfrac{1}{2}+\dfrac{1}{4}+\dfrac{3}{4}\right)\)

\(=-\left(x+\dfrac{1}{2}\right)^2-\dfrac{3}{4}\le-\dfrac{3}{4}\forall x\)

Dấu '=' xảy ra khi \(x=-\dfrac{1}{2}\)

b: ta có: \(B=3x^2+4x-13\)

\(=3\left(x^2+\dfrac{4}{3}x-\dfrac{13}{3}\right)\)

\(=3\left(x^2+2\cdot x\cdot\dfrac{2}{3}+\dfrac{4}{9}-\dfrac{43}{9}\right)\)

\(=3\left(x+\dfrac{2}{3}\right)^2-\dfrac{43}{3}\ge-\dfrac{43}{3}\forall x\)

Dấu '=' xảy ra khi \(x=-\dfrac{2}{3}\)

`P(x)=\(4x^2+x^3-2x+3-x-x^3+3x-2x^2\)

`= (x^3-x^3)+(4x^2-2x^2)+(-2x-x+3x)+3`

`= 2x^2+3`

`Q(x)=`\(3x^2-3x+2-x^3+2x-x^2\)

`= -x^3+(3x^2-x^2)+(-3x+2x)+2`

`= -x^3+2x^2-x+2`

`P(x)-Q(x)-R(x)=0`

`-> P(X)-Q(x)=R(x)`

`-> R(x)=P(x)-Q(x)`

`-> R(x)=(2x^2+3)-(-x^3+2x^2-x+2)`

`-> R(x)=2x^2+3+x^3-2x^2+x-2`

`= x^3+(2x^2-2x^2)+x+(3-2)`

`= x^3+x+1`

`@`\(\text{dn inactive.}\)

a: P(x)-Q(x)-R(x)=0

=>R(x)=P(x)-Q(x)

=2x^2+3+x^3-2x^2+x-2

=x^3+x+1