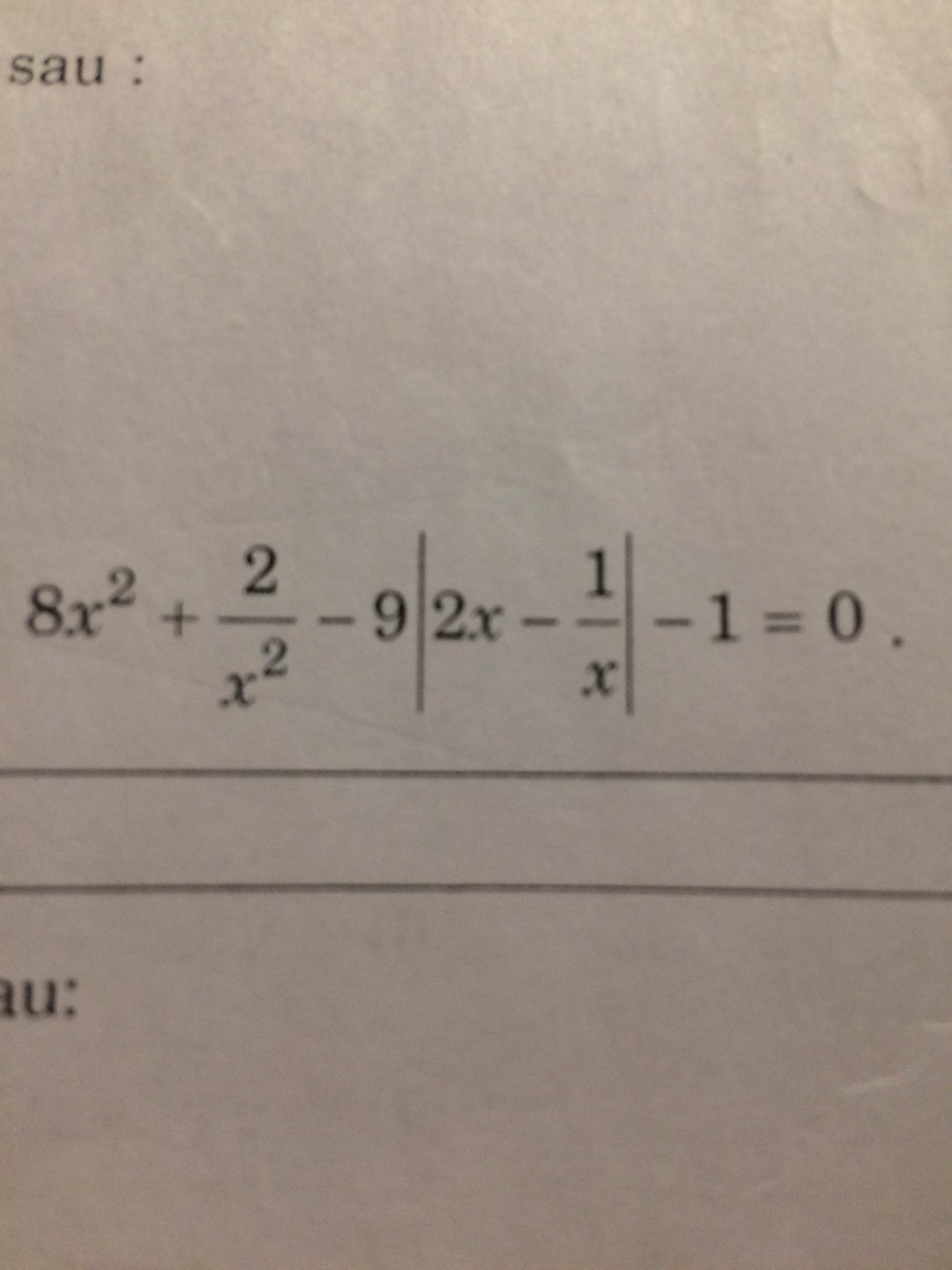Question

Help!!

Answer 1

\(J=\dfrac{1}{\sqrt{1-x}+\sqrt{1+x}}\)

\(\Rightarrow J^2=\dfrac{1}{\left(1-x\right)+2\sqrt{\left(1-x\right)\left(1+x\right)}+\left(1+x\right)}=\dfrac{1}{2+2\sqrt{\left(1-x\right)\left(1+x\right)}}\)

Ta có: \(-1\le x\le1\Rightarrow\left(1-x\right)\left(1+x\right)\ge0\)

\(\Rightarrow2+2\sqrt{\left(1-x\right)\left(1+x\right)}\ge2\)

\(\Rightarrow0< \dfrac{1}{2+2\sqrt{\left(1-x\right)\left(1+x\right)}}\le\dfrac{1}{2}\) hay \(J^2\le\dfrac{1}{2}\)

\(\Rightarrow0< J\le\dfrac{\sqrt{2}}{2}\)

\(MaxJ=\dfrac{\sqrt{2}}{2}\Leftrightarrow\left(1+x\right)\left(1-x\right)=0\Leftrightarrow x=\pm1\)

Vậy \(a=\dfrac{\sqrt{2}}{2}\)