Từ S kẻ \(SH\perp AC\) (1)
Ta có: \(\left\{{}\begin{matrix}SB\perp SA\\SB\perp SC\end{matrix}\right.\) \(\Rightarrow SB\perp\left(SAC\right)\Rightarrow SB\perp AC\) (2)
(1);(2) \(\Rightarrow AC\perp\left(SBH\right)\)
Trong mp (SBH), từ S kẻ \(SK\perp BH\Rightarrow SK\perp\left(ABC\right)\)
\(\Rightarrow SK=d\left(S;\left(ABC\right)\right)\)
\(\dfrac{1}{SH^2}=\dfrac{1}{SA^2}+\dfrac{1}{SC^2}\Rightarrow SH=\dfrac{SA.AC}{\sqrt{SA^2+SC^2}}=\dfrac{a\sqrt{3}}{2}\)
\(\dfrac{1}{SK^2}=\dfrac{1}{SB^2}+\dfrac{1}{SH^2}\Rightarrow SK=\dfrac{SB.SH}{\sqrt{SB^2+SH^2}}=\dfrac{a\sqrt{66}}{11}\)

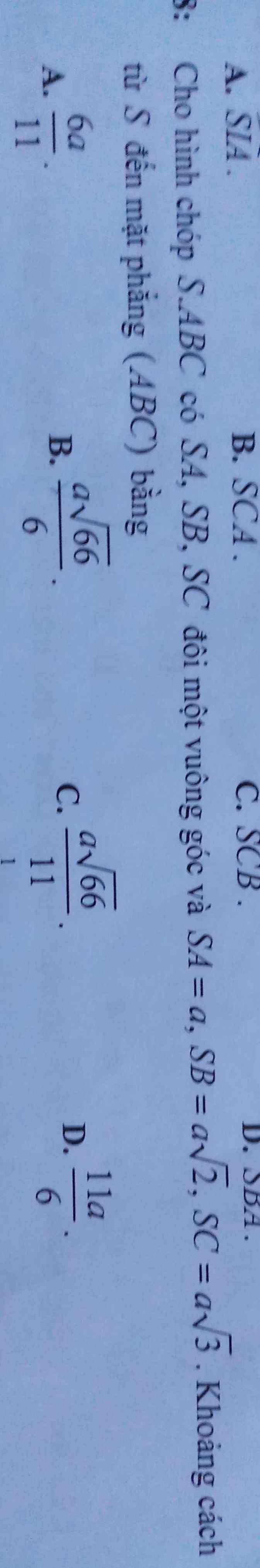



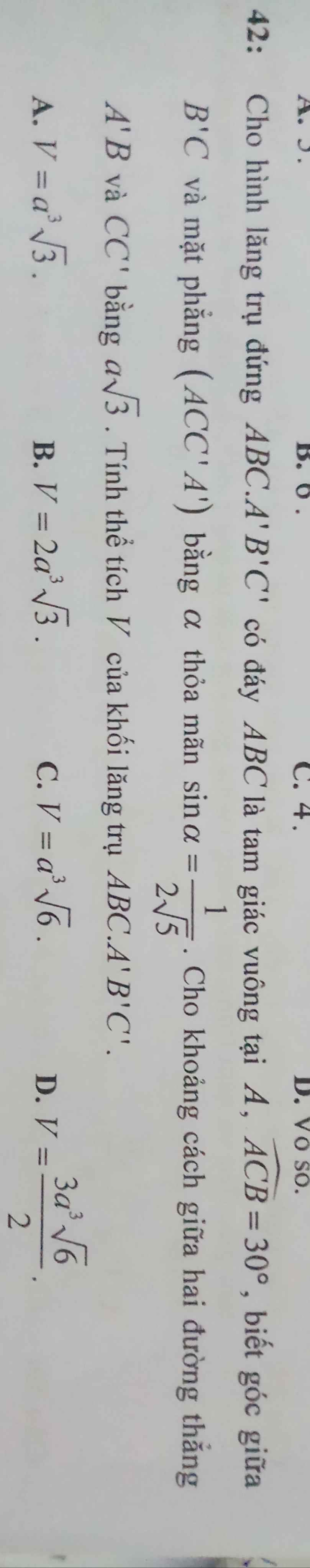












 Giải giúp em câu hai với câu 3 nha
Giải giúp em câu hai với câu 3 nha