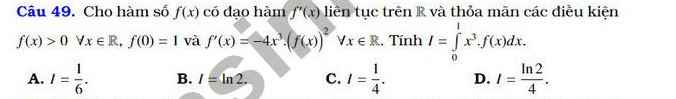Cho hàm số f(x) có đạo hàm cấp hai liên tục trên \(\left(0;+\infty\right)\) và thoả mãn \(f\left(\frac{1}{4}\right)=2\), \(f'\left(\frac{1}{4}\right)=-4,\) \(f\left(x\right)>0\), \(\left(x.f'\left(x\right)\right)^2+f\left(x\right).x^2.f''\left(x\right)=\frac{1}{x}\) với mọi \(x\in\left(0;+\infty\right)\) . Đặt \(m=\int_1^{16}f'\left(\frac{x}{4}\right)dx\), khẳng định nào sau đây đúng
\(A.m=-6\)
\(B.m=\frac{1}{2}\)
\(C.m=-4\)
\(D.m=\frac{5}{2}\)
\(\Leftrightarrow\left[f'\left(x\right)\right]^2+f\left(x\right).f''\left(x\right)=\frac{1}{x^3}\)
\(\Leftrightarrow\left[f'\left(x\right).f\left(x\right)\right]'=\frac{1}{x^3}\)
Lấy nguyên hàm 2 vế:
\(f'\left(x\right).f\left(x\right)=\int\frac{1}{x^3}dx=-\frac{1}{2x^2}+C\)
Thay \(x=\frac{1}{4}\Rightarrow-8=-8+C\Rightarrow C=0\)
\(\Rightarrow f'\left(x\right)f\left(x\right)=-\frac{1}{2x^2}\)
Tiếp tục lấy nguyên hàm 2 vế:
\(\int f\left(x\right).f'\left(x\right)dx=-\frac{1}{2}\int\frac{1}{x^2}dx\)
\(\Leftrightarrow\frac{1}{2}f^2\left(x\right)=\frac{1}{2x}+C\)
Thay \(x=\frac{1}{4}\Rightarrow2=2+C\Rightarrow C=0\Rightarrow f^2\left(x\right)=\frac{1}{x}\)
\(\Rightarrow f\left(x\right)=\frac{1}{\sqrt{x}}\Rightarrow f'\left(x\right)=-\frac{1}{2x\sqrt{x}}\)
\(\Rightarrow m=\int\limits^{16}_1-\frac{1}{\frac{2x}{4}\sqrt{\frac{x}{4}}}dx=-6\)