\(\begin{array}{l}A + B = (6{x^4} - 4{x^3} + x - \dfrac{1}{3}) + ( - 3{x^4} - 2{x^3} - 5{x^2} + x + \dfrac{2}{3})\\ = 6{x^4} - 4{x^3} + x - \dfrac{1}{3} - 3{x^4} - 2{x^3} - 5{x^2} + x + \dfrac{2}{3}\\ = (6{x^4} - 3{x^4}) + ( - 4{x^3} - 2{x^3}) - 5{x^2} + (x + x) + ( - \dfrac{1}{3} + \dfrac{2}{3})\\ = 3{x^4} - 6{x^3} - 5{x^2} + 2x + \dfrac{1}{3}\\A - B = (6{x^4} - 4{x^3} + x - \dfrac{1}{3}) - ( - 3{x^4} - 2{x^3} - 5{x^2} + x + \dfrac{2}{3})\\ = 6{x^4} - 4{x^3} + x - \dfrac{1}{3} + 3{x^4} + 2{x^3} + 5{x^2} - x - \dfrac{2}{3}\\ = (6{x^4} + 3{x^4}) + ( - 4{x^3} + 2{x^3}) + 5{x^2} + (x - x) + ( - \dfrac{1}{3} - \dfrac{2}{3})\\ = 9{x^4} - 2{x^3} + 5{x^2} - 1\end{array}\)\(\begin{array}{l}A + B = (6{x^4} - 4{x^3} + x - \dfrac{1}{3}) + ( - 3{x^4} - 2{x^3} - 5{x^2} + x + \dfrac{2}{3})\\ = 6{x^4} - 4{x^3} + x - \dfrac{1}{3} - 3{x^4} - 2{x^3} - 5{x^2} + x + \dfrac{2}{3}\\ = (6{x^4} - 3{x^4}) + ( - 4{x^3} - 2{x^3}) - 5{x^2} + (x + x) + ( - \dfrac{1}{3} + \dfrac{2}{3})\\ = 3{x^4} - 6{x^3} - 5{x^2} + 2x + \dfrac{1}{3}\\A - B = (6{x^4} - 4{x^3} + x - \dfrac{1}{3}) - ( - 3{x^4} - 2{x^3} - 5{x^2} + x + \dfrac{2}{3})\\ = 6{x^4} - 4{x^3} + x - \dfrac{1}{3} + 3{x^4} + 2{x^3} + 5{x^2} - x - \dfrac{2}{3}\\ = (6{x^4} + 3{x^4}) + ( - 4{x^3} + 2{x^3}) + 5{x^2} + (x - x) + ( - \dfrac{1}{3} - \dfrac{2}{3})\\ = 9{x^4} - 2{x^3} + 5{x^2} - 1\end{array}\)
Bài 26. Phép cộng và phép trừ đa thức một biến
Đúng 1
Bình luận (0)
Các câu hỏi tương tự
Cho các đa thức:
\(A = 3{x^4} - 2{x^3} - x + 1;B = - 2{x^3} + 4{x^2} + 5x;C = - 3{x^4} + 2{x^2} + 5\)
Tính A + B + C; A – B + C và A – B – C
Tìm tổng của hai đa thức sau bằng cách nhóm các hạng tử cùng bậc:
x2 – 3x + 2 và 4x3 – x2 + x - 1
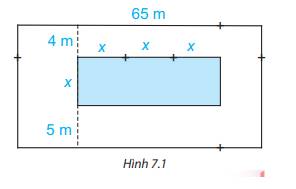
Trên một mảnh đất hình chữ nhật có chiều dài 65 m, người ta định làm một bể bơi có chiều rộng là x mét, chiều dài gấp 3 lần chiều rộng. Sơ đồ và kích thước cụ thể (tính bằng mét) đươc cho trong Hình 7.1. Tìm đa thức (biến x):a) Biểu thị diện tích bể bơib) Biểu thị diện tích mảnh đấtc) Biểu thị diện tích phần đất xung quanh bể bơi.
Đọc tiếp
Trên một mảnh đất hình chữ nhật có chiều dài 65 m, người ta định làm một bể bơi có chiều rộng là x mét, chiều dài gấp 3 lần chiều rộng. Sơ đồ và kích thước cụ thể (tính bằng mét) đươc cho trong Hình 7.1. Tìm đa thức (biến x):
a) Biểu thị diện tích bể bơi
b) Biểu thị diện tích mảnh đất
c) Biểu thị diện tích phần đất xung quanh bể bơi.
Đặt tính cộng để tìm tổng của ba đa thức sau:
A = 2x3 – 5x2 + x – 7
B = x2 – 2x + 6
C = -x3 + 4x2 - 1
Tìm tổng của hai đa thức: x3 – 5x + 2 và x3 – x2 +6x – 4.
Cho đa thức A = x4 – 3x2 – 2x + 1. Tìm các đa thức B và C sao cho:
A + B = 2x5 + 5x3 – 2
A – C = x3
Cho hai đa thức M = 0,5x4 – 4x3 + 2x – 2,5 và N = 2x3 + x2 + 1,5
Hãy tính tổng M + N (trình bày theo 2 cách)
Bạn Nam được phân công mua một số sách làm quà tặng trong buổi tổng kết cuối năm học của lớp. Nam dự định mau ba loại sách với giá bán như bảng sau. Giả sử Nam cần mua x cuốn sách khoa học, x+8 cuốn sách tham khảo và x + 5 cuốn truyện tranh.a) Viết các đa thức biểu thị số tiền Nam phải trả cho từng loại sách.b) Tìm đa thức biểu thị tổng số tiền Nam phải trả để mua số sách đó.
Đọc tiếp
Bạn Nam được phân công mua một số sách làm quà tặng trong buổi tổng kết cuối năm học của lớp. Nam dự định mau ba loại sách với giá bán như bảng sau. Giả sử Nam cần mua x cuốn sách khoa học, x+8 cuốn sách tham khảo và x + 5 cuốn truyện tranh.
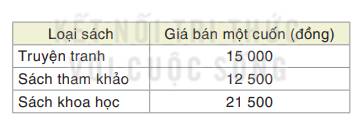
a) Viết các đa thức biểu thị số tiền Nam phải trả cho từng loại sách.
b) Tìm đa thức biểu thị tổng số tiền Nam phải trả để mua số sách đó.
Cho hai đa thức P = x4 + 3x3 – 5x2 + 7x và Q = -x3 + 4x2 – 2x +1
Tìm hiệu P – Q bằng cách đặt tính trừ: đặt đa thức Q dưới đa thức P sao cho các hạng tử cùng bậc thẳng cột với nhau rồi trừ theo từng cột.


