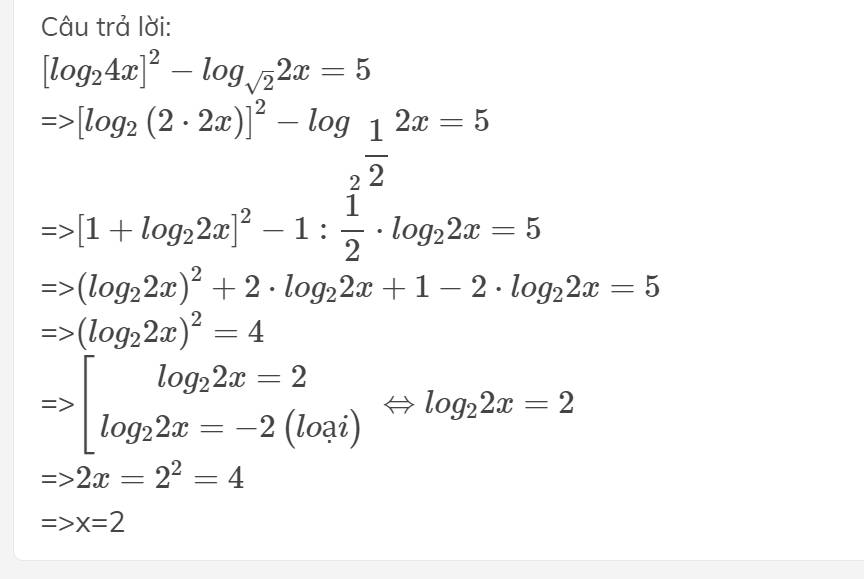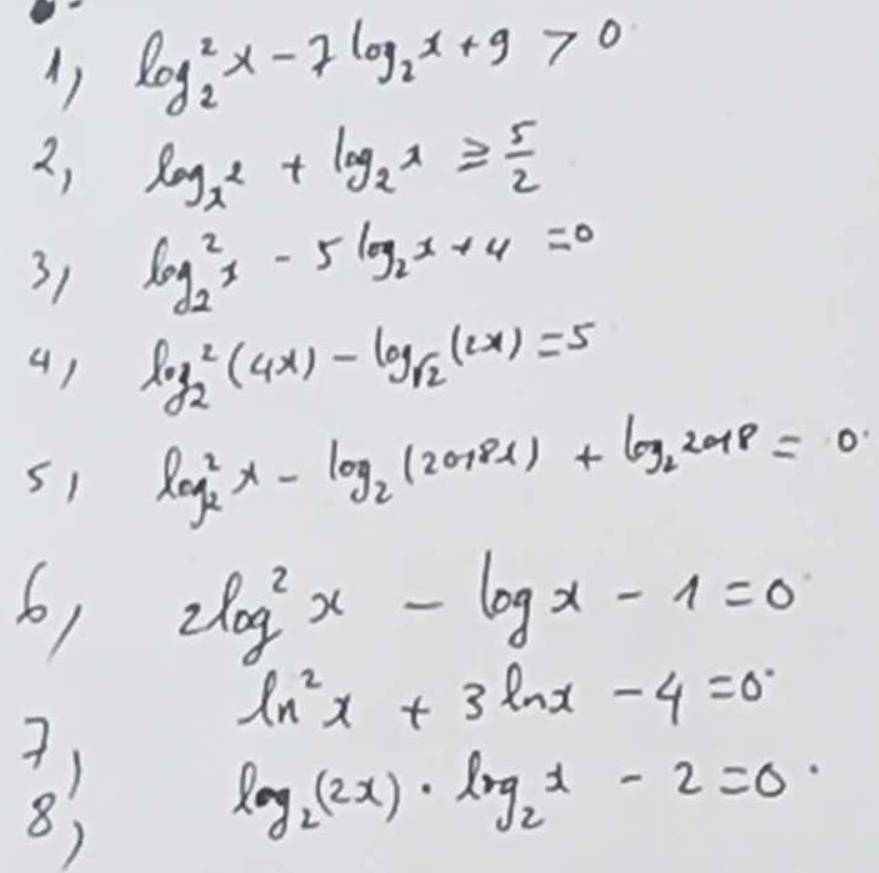3: \(log_2^2x-5\cdot log_2x=4=0\)
=>\(\left(log_2x-1\right)\left(log_2x-4\right)=0\)
=>\(\left[{}\begin{matrix}log_2x-1=0\\log_2x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}log_2x=1\\log_2x=4\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=0\\x=4\end{matrix}\right.\)
6: \(2\cdot log^2x-logx-1=0\)
=>\(2\cdot log^2x-2logx+logx-1=0\)
=>\(\left(logx-1\right)\left(2logx+1\right)=0\)
=>\(\left[{}\begin{matrix}logx=1\left(nhận\right)\\logx=-\dfrac{1}{2}\left(loại\right)\end{matrix}\right.\Leftrightarrow logx=1\)
=>\(x=10\)
7: \(ln^2x+3lnx-4=0\)
=>\(\left(lnx+4\right)\left(lnx-1\right)=0\)
=>\(\left[{}\begin{matrix}lnx+4=0\\lnx-1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}lnx=-4\\lnx=1\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=e^{-4}\\x=e^1=e\end{matrix}\right.\)
2:
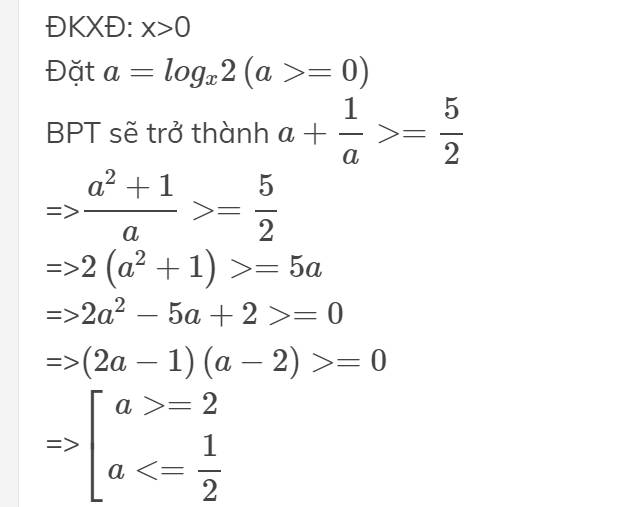
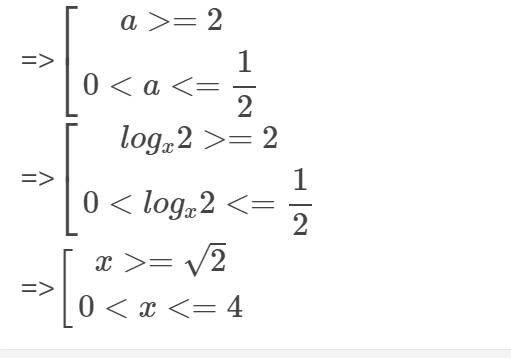
4: