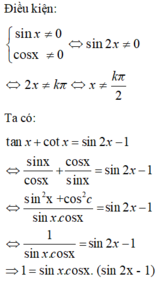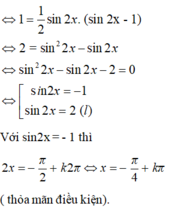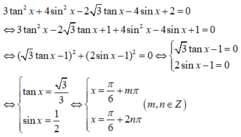ĐKXĐ: \(sin2x\ne0\)

\(\Leftrightarrow\frac{cos^2x-sin^2x}{sinx.cosx}+4sin2x=\frac{2}{sin2x}\)

\(\Leftrightarrow\frac{2cos2x}{sin2x}+4sin2x=\frac{2}{sin2x}\)

\(\Leftrightarrow cos2x+2sin^22x=1\)

\(\Leftrightarrow cos2x-2\left(1-cos^22x\right)-1=0\)

\(\Leftrightarrow2cos^22x+cos2x-3=0\)

\(\Leftrightarrow\left[{}\begin{matrix}cos2x=1\left(ktm\right)\\cos2x=-\frac{3}{2}>1\left(l\right)\end{matrix}\right.\)

Phương trình vô nghiệm

Điều kiện của phương trình: sinx ≠ 0, cos ≠ 0, tan ≠ -1.

Biến đổi tương đương đã cho, ta được

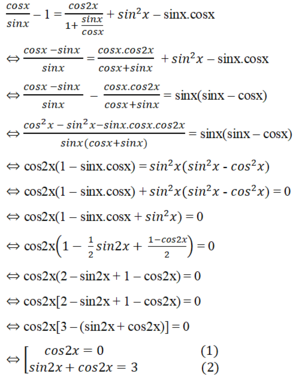
Phương trình (2) vô nghiệm vì |sin2x + cos2x| ≥ √2.

Phương trình (1) có nghiệm 2x = π/2+kπ,k ∈ Z

⇒ x = π/4+ k π/2,k ∈ Z.

Giá trị x = π/4+ k π/2, k = 2n + 1,

với n ∈ Z bị loại do điều kiện tanx ≠ -1.

Pt vô nghiệm

Chọn  B

Đáp án D

ĐKXĐ: \(x\ne k\dfrac{\pi}{2}\)

\(tanx+\dfrac{1}{tanx}=2\)

\(\Rightarrow tan^2x+1=2tanx\)

\(\Leftrightarrow\left(tanx-1\right)^2=0\)

\(\Leftrightarrow tanx=1\)

\(\Rightarrow x=\dfrac{\pi}{4}+k\pi\) (thỏa mãn)

ĐKXĐ: ...

a/ \(\frac{sin2x}{cos2x}+\frac{cosx}{sinx}=8cos^2x\)

\(\Leftrightarrow sin2x.sinx+cos2x.cosx=8cos^2x.sinx.cos2x\)

\(\Leftrightarrow cosx=4sin2x.cos2x.cosx\)

\(\Leftrightarrow cosx=2sin4x.cosx\)

\(\Leftrightarrow cosx\left(2sin4x-1\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\sin4x=\frac{1}{2}\end{matrix}\right.\) \(\Leftrightarrow...\)

b/ \(\frac{cosx}{sinx}-\frac{sinx}{cosx}+4sin2x=\frac{1}{sinx.cosx}\)

\(\Leftrightarrow cos^2x-sin^2x+4sin2x.sinx.cosx=1\)

\(\Leftrightarrow cos2x+2sin^22x=1\)

\(\Leftrightarrow cos2x+2\left(1-cos^22x\right)=1\)

\(\Leftrightarrow-2cos^22x+cos2x+1=0\)

\(\Leftrightarrow\left[{}\begin{matrix}cos2x=1\\cos2x=-\frac{1}{2}\end{matrix}\right.\) \(\Leftrightarrow...\)

1c/

\(5sinx-2=3\left(1-sinx\right)\frac{sin^2x}{1-sin^2x}\)

\(\Leftrightarrow5sinx-2=\frac{3sin^2x}{1+sinx}\)

\(\Leftrightarrow\left(5sinx-2\right)\left(1+sinx\right)=3sin^2x\)

\(\Leftrightarrow5sin^2x+3sinx-2=3sin^2x\)

\(\Leftrightarrow2sin^2x+3sinx-2=0\)

\(\Leftrightarrow\left[{}\begin{matrix}sinx=\frac{1}{2}\\sinx=-2\left(l\right)\end{matrix}\right.\) \(\Rightarrow x=...\)

Bài 2:

a/ \(\Leftrightarrow\frac{\left(m+1\right)\left(1-cos2x\right)}{2}-sin2x+cos2x=0\)

\(\Leftrightarrow2sin2x+\left(m-1\right)cos2x=m+1\)

Theo điều kiện có nghiệm của pt lượng giác bậc nhất:

\(4+\left(m-1\right)^2\ge\left(m+1\right)^2\)

\(\Leftrightarrow4m\le4\Rightarrow m\le1\)

Bài 2:

b/ \(\Leftrightarrow1-cos2x+msin2x=2m\)

\(\Leftrightarrow msin2x-cos2x=2m-1\)

Theo điều kiện có nghiệm của pt lượng giác bậc nhất:

\(m^2+1\ge\left(2m-1\right)^2\)

\(\Leftrightarrow3m^2-4m\le0\)

\(\Rightarrow0\le m\le\frac{4}{3}\)

c/ Với \(cosx=0\) không phải là nghiệm

Với \(cosx\ne0\), chia 2 vế cho \(cos^2x\) ta được:

\(tan^2x-4tanx+m-2=0\)

Đặt \(tanx=t\Rightarrow t\in\left[0;1\right]\)

Phương trình trở thành: \(t^2-4t+m-2=0\)

\(\Leftrightarrow f\left(t\right)=t^2-4t-2=-m\)

Dựa vào đồ thị hàm \(f\left(t\right)=t^2-4t-2\), để \(y=-m\) cắt \(y=f\left(t\right)\) với \(t\in\left[0;1\right]\) \(\Rightarrow-5\le-m\le-2\)

\(\Rightarrow2\le m\le5\)

Điều kiện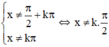 

      tanx – 2.cotx + 1 = 0

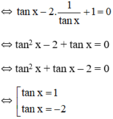
 (Thỏa mãn điều kiện).

Vậy phương trình có tập nghiệm

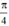{ + kπ; arctan(-2) + kπ} (k ∈ Z)

câu 1

⇒ \(\dfrac{cosx}{sinx}\) - \(\dfrac{sinx}{cosx}\) -\(\dfrac{2cos4x}{2sinxcosx}\) =0

⇔ \(\dfrac{cos^2x-sin^2x}{sinx.cosx}\) -\(\dfrac{cos4x}{sinx.cosx}\)= 0

⇔ \(\dfrac{cos2x-cos4x}{sinx.cosx}\) = 0

\(\left[{}\begin{matrix}cos2x=cos4x\\sin2x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=4x+k2\pi\\2x=-4x+k2\pi\\2x=k\pi\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-k\pi\\x=\dfrac{k\pi}{3}\\x=\dfrac{k\pi}{2}\end{matrix}\right.\) (k∈ Z)

câu 2 dùng công thức biến đổi tích thành tổng thành cos 4x + cos 2x sau đó phương trình trở thành sin x - cos 4x=0