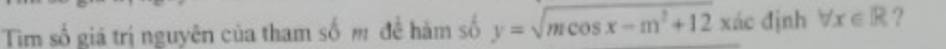Có \(sin^23x\le1;sin^2x\le1;sin3x\ge-1\)
\(\Rightarrow\left\{{}\begin{matrix}4sin^23x.sin^2x\le4\\6+2sin3x\ge6+2.-1=4\end{matrix}\right.\)
Dấu "=" xảy ra khi \(\left\{{}\begin{matrix}sin^23x=1\\sin^2x=1\\sin3x=-1\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}sin3x=1\\cos^2x=0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{\pi}{6}+\dfrac{k2\pi}{3}\\x=\dfrac{\pi}{2}+k2\pi\end{matrix}\right.\)\(\Rightarrow x=\dfrac{\pi}{2}+k2\pi\)
Vậy...





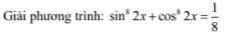
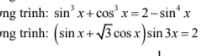
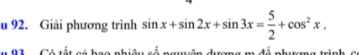


 mọi người giải giúp em theo phương pháp tự luận với ạ
mọi người giải giúp em theo phương pháp tự luận với ạ