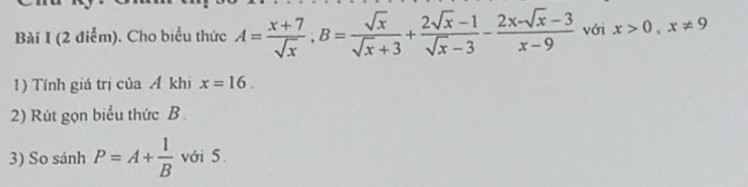a. Em tự tính
b.
\(B=\dfrac{\sqrt{x}-3}{\sqrt{x}-2}-\dfrac{2\sqrt{x}-9}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}-\dfrac{2\sqrt{x}-9}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{x-9-2\sqrt{x}+9}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}=\dfrac{\sqrt{x}\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{\sqrt{x}}{\sqrt{x}+3}\)
c.
\(P=\dfrac{B}{A}=\dfrac{\sqrt{x}}{\sqrt{x}-3}\)
\(\left|P\right|>P\Leftrightarrow P< 0\)
\(\Rightarrow\dfrac{\sqrt{x}}{\sqrt{x}-3}< 0\) \(\Rightarrow\left\{{}\begin{matrix}x\ne0\\\sqrt{x}-3< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ne0\\x< 9\end{matrix}\right.\)
Kết hợp điều kiện đề bài \(\Rightarrow\left\{{}\begin{matrix}0< x< 9\\x\ne4\end{matrix}\right.\)
a: Khi x=4 thì \(A=\dfrac{2-3}{2+3}=\dfrac{-1}{5}\)
b: \(=\dfrac{x-9-2\sqrt{x}+9}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-2\right)}=\dfrac{x-2\sqrt{x}}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-2\right)}=\dfrac{\sqrt{x}}{\sqrt{x}+3}\)
c: P=B:A
\(=\dfrac{\sqrt{x}}{\sqrt{x}+3}\cdot\dfrac{\sqrt{x}+3}{\sqrt{x}-3}=\dfrac{\sqrt{x}}{\sqrt{x}-3}\)
Để |P|>P thì P<=0
=>căn x-3<0
=>0<x<9

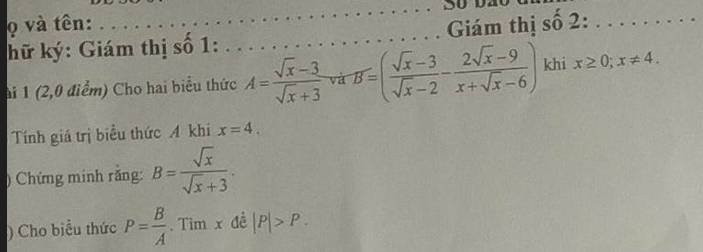




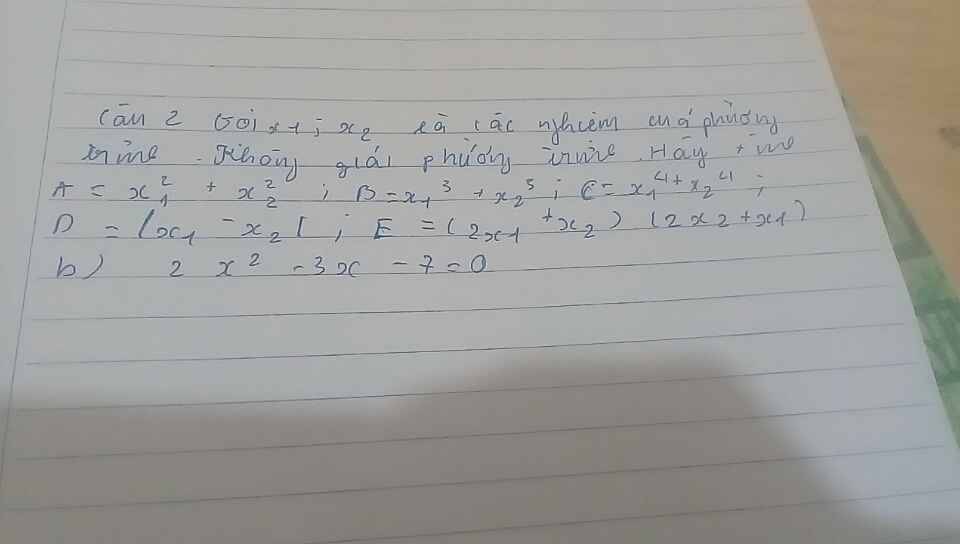

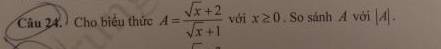

 giup minh cau nay voi
giup minh cau nay voi giup minh cau nay voi
giup minh cau nay voi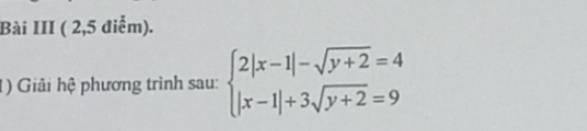
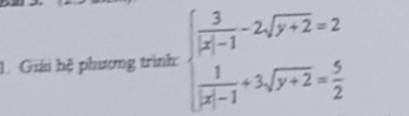 giup minh cau nay voi
giup minh cau nay voi