Cho \(-5\le m\le-1\).Tìm GTNN,GTLN của \(T=\left|3m-2\right|\)

Những câu hỏi liên quan
Cho 3 số a,b,c thỏa mãn \(0\le a\le b\le c\le1\) Tìm GTLN và GTNN của biểu thức \(B=\left(a+b+c+3\right)\left(\dfrac{1}{a+1}+\dfrac{1}{b+1}+\dfrac{1}{c+1}\right)\)
Tìm GTNN của biểu thức sau:
\(N=\dfrac{3x}{2}+\dfrac{1}{x+1}\) với \(x>-1\)
Tìm GTLN của biểu thức:
\(Q=\left(6x+3\right)\left(5-2x\right)\) với\(\dfrac{-1}{2}\le x\le\dfrac{5}{2}\)
tìm GTLN GTNN của
a, f(x)= \(3\sqrt{x-1}+4\sqrt{5-x}\) \(\left(1\le x\le5\right)\)
b, f(x)= 3x +\(4\sqrt{3-x^2}\) \(\left(-\sqrt{3}\le x\le\sqrt{3}\right)\)
Áp dụng BĐT Bunhiacopxki ta có :
\(\left(3\sqrt{x-1}+4\sqrt{5-x}\right)^2\le\left(3^2+4^2\right)\left(x-1+5-x\right)\)
\(\Leftrightarrow\left(3\sqrt{x-1}+4\sqrt{5-x}\right)^2\le100\)
\(\Leftrightarrow f\left(x\right)\le10\)
Dấu "=" xảy ra :
\(\Leftrightarrow\frac{\sqrt{x-1}}{3}=\frac{\sqrt{5-x}}{4}\)
Vậy...
TÌM GTNN CỦA HÀM SỐ SAU:
a) y=\(\dfrac{x^2+x+2}{\sqrt{x^2+x+1}}\)
TÌM GTLN CỦA HÀM SỐ SAU:
b)y= \(x^2\sqrt{9-x^2}với-3\le x\le3\)
c)y=\(\left(1-x\right)^3\left(1+3x\right)với\dfrac{-1}{3}\le x\le1\)
\(a,\dfrac{x^2+x+2}{\sqrt{x^2+x+1}}=\dfrac{x^2+x+1+1}{\sqrt{x^2+x+1}}=\sqrt{x^2+x+1}+\dfrac{1}{\sqrt{x^2+x+1}}\left(1\right)\)
Áp dụng BĐT cosi: \(\left(1\right)\ge2\sqrt{\sqrt{x^2+x+1}\cdot\dfrac{1}{\sqrt{x^2+x+1}}}=2\)
Dấu \("="\Leftrightarrow x^2+x+1=1\Leftrightarrow x^2+x=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-1\end{matrix}\right.\)
Đúng 3
Bình luận (0)
\(\dfrac{2x-1}{4}-1\le\dfrac{5-3\left(x+1\right)}{6}\)
Tìm GTLN và GTNN của
B = \(\dfrac{2m+1}{m^2+2}\)
b) Gọi a là một giá trị của B
\(\Rightarrow B=\dfrac{2m+1}{m^2+2}=a\)
<=> am2 + 2a = 2m + 1
<=> am2 + 2a - 2m - 1 = 0
<=> a2m2 + 2a2 - 2am - a = 0 (cùng nhân cả 2 vế với a)
<=> (a2m2 - 2am + 1) + (2a2 - a - 1) = 0
<=> (am - 1)2 + (2a2 - a - 1) = 0
Vì (am - 1)2 \(\ge\) 0 với mọi x
=> 2a2 - a - 1 \(\le\) 0
<=> (a - 1)(a + 0,5) \(\le\) 0
<=> -0,5 \(\le\) a \(\le\) 1
Vậy max B là 1; min B là -0,5
Đúng 0
Bình luận (0)
a) \(\dfrac{2x-1}{4}-1\le\dfrac{5-3\left(x+1\right)}{6}\)
\(\Leftrightarrow\dfrac{2x-1-4}{4}\le\dfrac{5-3x+3}{6}\)
\(\Leftrightarrow\dfrac{2x-5}{4}\le\dfrac{8-3x}{6}\)
\(\Leftrightarrow\dfrac{3\left(2x-5\right)}{12}\le\dfrac{2\left(8-3x\right)}{12}\)
\(\Rightarrow6x-15\le16-6x\)
\(\Leftrightarrow6x+6x\le15+16\)
\(\Leftrightarrow12x\le31\)
\(\Leftrightarrow x\le\dfrac{31}{12}\)
Đúng 0
Bình luận (0)
cho \(0\le a\le b\le c\le1\) . Tìm GTLN của
\(Q=a^2\left(b-c\right)+b^2\left(c-b\right)+c^2\left(1-c\right)\)
Cho \(P=x\sqrt{5-x}+\left(3-x\right)\sqrt{2+x}\). Tìm GTLN và GTNN của P khi \(0\le x\le3\)
a,Cho x,y,z tm \(\left\{{}\begin{matrix}x^2+y^2+z^2=8\\x+y+z=4\end{matrix}\right.\). CM: \(-\dfrac{8}{3}\le x\le\dfrac{8}{3}\)
b, cho \(x^2+3y^2=1\). Tìm GTLN, GTNN của\(P=x-y\)
c, Cho \(P=\dfrac{x^2-\left(x-4y\right)^2}{x^2+4y^2}\left(x^2+y^2>0\right)\)
Tìm GTLN của P
\(c,P=\dfrac{x^2-x^2+8xy-16y^2}{x^2+4y^2}=\dfrac{8\left(\dfrac{x}{y}\right)-16}{\left(\dfrac{x}{y}\right)^2+4}\)
Đặt \(\dfrac{x}{y}=t\)
\(\Leftrightarrow P=\dfrac{8t-16}{t^2+4}\Leftrightarrow Pt^2+4P=8t-16\\ \Leftrightarrow Pt^2-8t+4P+16=0\)
Với \(P=0\Leftrightarrow t=2\)
Với \(P\ne0\Leftrightarrow\Delta'=16-P\left(4P+16\right)\ge0\)
\(\Leftrightarrow-P^2-4P+4\ge0\Leftrightarrow-2-2\sqrt{2}\le P\le-2+2\sqrt{2}\)
Vậy \(P_{max}=-2+2\sqrt{2}\Leftrightarrow t=\dfrac{4}{P}=\dfrac{4}{-2+2\sqrt{2}}=2+\sqrt{2}\)
\(\Leftrightarrow\dfrac{x}{y}=2+2\sqrt{2}\)
Đúng 3
Bình luận (2)
Bài a hình như sai đề rồi bạn.
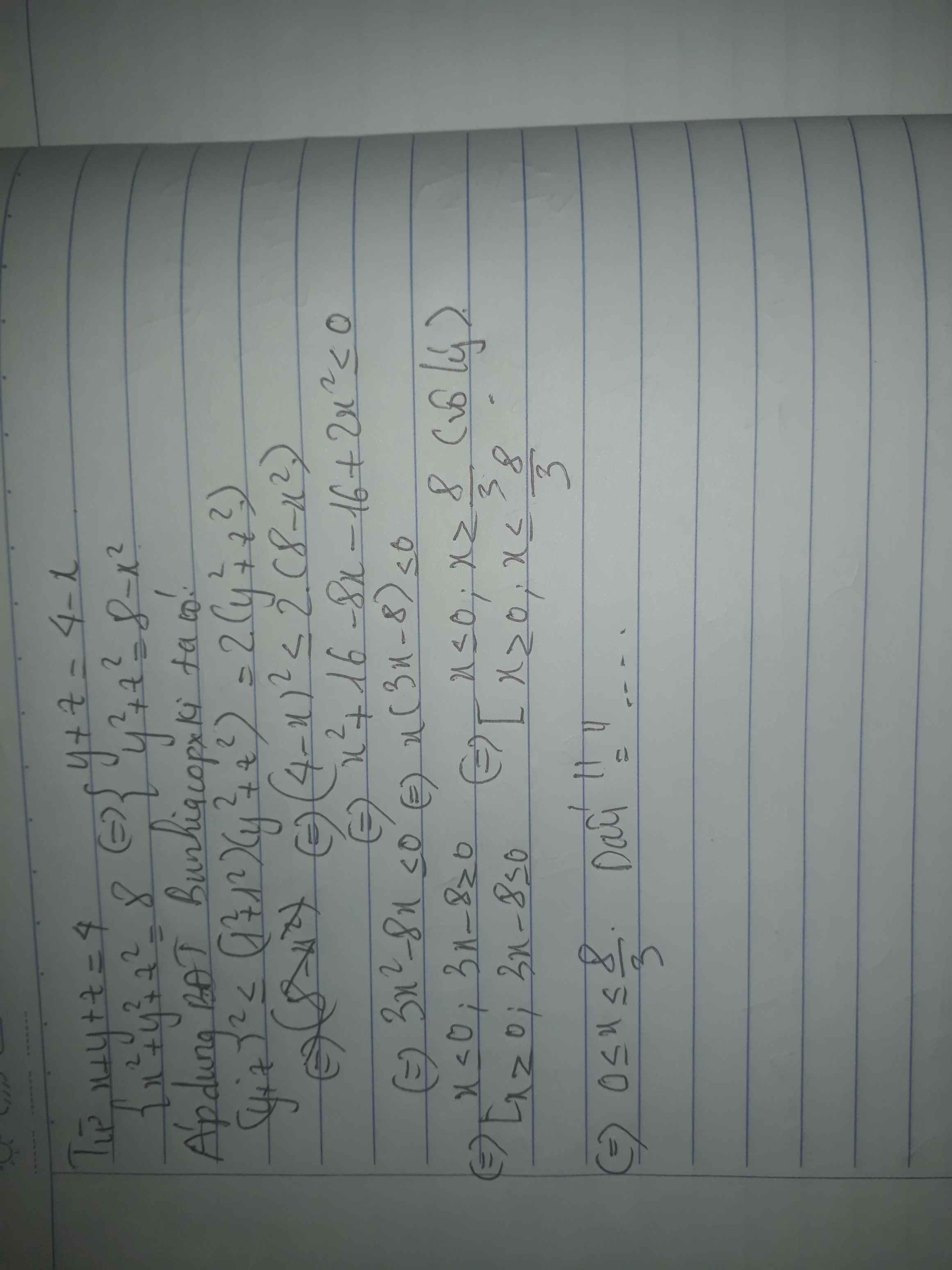
Đúng 3
Bình luận (2)
\(a,\text{Đặt }\left\{{}\begin{matrix}S=y+z\\P=yz\end{matrix}\right.\\ HPT\Leftrightarrow\left\{{}\begin{matrix}\left(y+z\right)^2-2yz+x^2=8\\x\left(y+z\right)+yz=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}S^2-2P+x^2=8\\Sx+P=4\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}S^2-2\left(4-Sx\right)+x^2=8\\P=4-Sx\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}S^2+2Sx+x^2-16=0\left(1\right)\\P=4-Sx\end{matrix}\right.\\ \left(1\right)\Leftrightarrow\left(S+x-4\right)\left(S+x+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}S=-x+4\Rightarrow P=\left(x-2\right)^2\\S=-x-4\Rightarrow P=\left(x+2\right)^2\end{matrix}\right.\)
Mà y,z là nghiệm của hệ nên \(S^2-4P\ge0\Leftrightarrow\left[{}\begin{matrix}\left(4-x\right)^2\ge4\left(x-2\right)^2\\\left(-4-x\right)^2\ge4\left(x+2\right)^2\end{matrix}\right.\Leftrightarrow-\dfrac{8}{3}\le x\le\dfrac{8}{3}\)
Đúng 2
Bình luận (0)
Xem thêm câu trả lời
Cho a, b, c không âm thoả mãn \(0\le a\le b\le c\le1\)
tìm GTLN của \(Q=a^2\left(b-c\right)+b^2\left(c-b\right)+c^2\left(1-c\right)\)



















