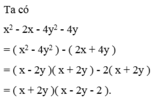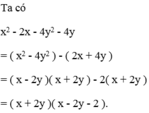Phân tích đa thức thành nhân tử : x2-4y2+9-6x

Những câu hỏi liên quan
Phân tích đa thức thành nhân tử x2-2x-4y2-4y
\(=\left(x-2y\right)\left(x+2y\right)-2\left(x+2y\right)=\left(x+2y\right)\left(x-2y-2\right)\)
Đúng 1
Bình luận (0)
= ( x2 - 4y2 ) - ( 2x + 4y )
= ( x - 2y ) ( x + 2y ) - 2 ( x - 2y )
= ( x - 2y ) ( x + 2y - 2 )
Đúng 1
Bình luận (0)
Phân tích đa thức thành nhân tử bằng phương pháp đặt nhân tử chung:
-x2-4xy-4y2
= \(-\left(x^2+4xy+4y^2\right)\)
= \(-\left(x+2y\right)^2\)
Đúng 1
Bình luận (0)
Phân tích đa thức thành nhân tử:
a)4x3y2-8x2y+12xy2
b)3x2-6xy-5x+10y
c)x2-49+4y2-4xy
d)x2-6x-16
a) \(4x^3y^2-8x^2y+12xy^2=4xy\left(x^2y-2x+3y\right)\)
b) \(3x^2-6xy-5x+10y=3x\left(x-2y\right)-5\left(x-2y\right)=\left(x-2y\right)\left(3x-5\right)\)
c) \(x^2-49+4y^2-4xy=\left(x-2y\right)^2-49=\left(x-2y-7\right)\left(x-2y+7\right)\)
d) \(x^2-6x-16=\left(x^2-6x+9\right)-25=\left(x-3\right)^2-25=\left(x-3-5\right)\left(x-3+5\right)=\left(x-8\right)\left(x+2\right)\)
Đúng 1
Bình luận (0)
a) 4x3y2−8x2y+12xy2=4xy(x2y−2x+3y)4x3y2−8x2y+12xy2=4xy(x2y−2x+3y)
b) 3x2−6xy−5x+10y=3x(x−2y)−5(x−2y)=(x−2y)(3x−5)3x2−6xy−5x+10y=3x(x−2y)−5(x−2y)=(x−2y)(3x−5)
c) x2−49+4y2−4xy=(x−2y)2−49=(x−2y−7)(x−2y+7)x2−49+4y2−4xy=(x−2y)2−49=(x−2y−7)(x−2y+7)
d) x2−6x−16=(x2−6x+9)−25=(x−3)2−25=(x−3−5)(x−3+5)=(x−8)(x+2)
Đúng 1
Bình luận (0)
Phân tích đa thức thành nhân tử:
a)4x3y2-8x2y+12xy2
b)3x2-6xy-5x+10y
c)x2-49+4y2-4xy
d)x2-6x-16
a) \(4x^3y^2-8x^2y+12xy^2=4xy.x^2y-4xy.2x+4xy.3y=4xy\left(x^2y-2x+3y\right)\)
b) \(3x^2-6xy-5x+10y=\left(3x^2-6xy\right)-\left(5x-10y\right)=3x\left(x-2y\right)-5\left(x-2y\right)=\left(x-2y\right)\left(3x-5\right)\)
c) \(x^2-49+4y^2-4xy=\left(x^2-4xy+4y^2\right)-49=\left(x-2y\right)^2-7^2=\left(x-2y-7\right)\left(x-2y+7\right)\)
d) \(x^2-6x-16=\left(x^2-8x\right)+\left(2x-16\right)=x\left(x-8\right)+2\left(x-8\right)=\left(x-8\right)\left(x+2\right)\)
Đúng 1
Bình luận (0)
Phân tích các đa thức sau thành nhân tử:
a, x2 – 2x – 4y2 + 4y
b, x⁴ + 6x²y + 9y² – 1
\(a,=\left(x-2y\right)\left(x+2y\right)-2\left(x-2y\right)=\left(x-2y\right)\left(x+2y-2\right)\\ b,=\left(x^2+3y\right)^2-1=\left(x^2+3y-1\right)\left(x^2+3y+1\right)\)
Đúng 4
Bình luận (0)
\(b)x^4+6x^2y+9y^2-1\\=(x^4+6x^2y+9y^2)-1\\=(x^2+3y)^2-1\\=(x^2+3y-1)(x^2+3y+1)\\a) x^2-2x+4y^2+4y\\=(x^2-4y^2)-(2x-4y)\\=(x-2y)(x+2y)-2(x-2y)\\=(x-2y)(x+2y-2)\)
Đúng 2
Bình luận (0)
\(x^2-2x-4y^2+4y=x^2-2x+1-4y^2+4y-1=\left(x-1\right)^2-\left(2y-1\right)^2=\left(x-1-2y+1\right)\left(x-1+2y-1\right)=\left(x-2y\right)\left(x+2y-2\right)\)
\(x^4+6x^2y+9y^2-1=\left(x^2+3y\right)^2-1=\left(x^2+3y-1\right)\left(x^2+3y+1\right)\)
Đúng 2
Bình luận (0)
Phân tích các đa thức sau thành nhân tử x 2 - 2 x - 4 y 2 - 4 y
Phân tích các đa thức sau thành nhân tử x 2 - 2 x - 4 y 2 - 4 y
x2–4xy +4y2–z2+ 2zt –t2 = ??? (Phân tích đa thức thành nhân tử)
x2 - 4xy + 4y2 - z2 + 2zt - t2
= (x2 - 4xy + 4y2) - (z2 - 2zt + t2)
= (x - 2y)2 - (z - t)2
= (x - 2y + z - t)(x - 2y - z + t)
Đúng 1
Bình luận (0)
Câu 13: Đa thức x2 – 6x –4y2 + 9 được phân tích thành nhân tử có kết quả là
A. (x + 2y + 3)(x + 2y - 3)
B. (x – 3 – 2y)(x – 3 + 2y)
C. (x – 2y - 3)(x - 2y + 3)
D. (x – 3 – 4y)(x – 3 + 4y)
phân tích đa thức thành nhân tử
(x2 + 4y2 - 20)2 - 16(xy - 4)2
\(\left(x^2+4y^2-20\right)^2-16\left(xy-4\right)^2=\left(x^2+4y^2-20\right)^2-\left(4xy-16\right)^2=\left(x^2+4y^2-20-4xy+16\right)\left(x^2+4y^2-20+4xy-16\right)=\left[\left(x-2y\right)^2-4\right]\left[\left(x+2y\right)^2-36\right]=\left(x-2y-2\right)\left(x-2y+2\right)\left(x+2y-6\right)\left(x+2y+6\right)\)
Đúng 6
Bình luận (1)
\(\left(x^2+4y^2-20\right)^2-\left(4xy-16\right)^2\)
\(=\left(x^2+4y^2-20-4xy+16\right)\left(x^2+4y^2-20+4xy-16\right)\)
\(=\left[\left(x-2y\right)^2-4\right]\left[\left(x+2y\right)^2-36\right]\)
\(=\left(x-2y-2\right)\left(x-2y+2\right)\left(x+2y-6\right)\left(x+2y+6\right)\)
Đúng 1
Bình luận (0)