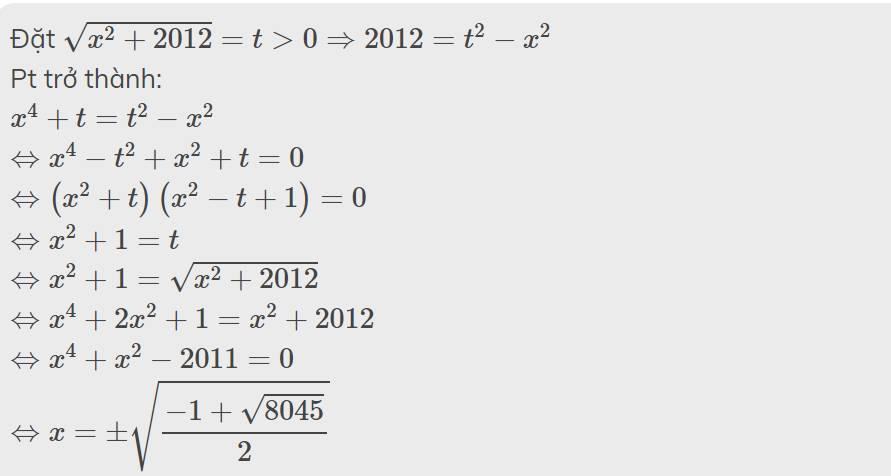Bài 1: Căn bậc hai
Các câu hỏi tương tự
Giải phương trình :a,\(\sqrt{1-x}=\sqrt{6-x}-\sqrt{-5-2x}\)
b,\(\sqrt{x^2 +1-2x}+\sqrt{x^2+4-4x}=\sqrt{1+2012^2+\frac{2012^2}{2013^2}}+\frac{2012}{2013}\)
c,\(x^2-x-1=\sqrt{8x+1}\)
tìm nghiệm nguyên của phương trình :
\(\dfrac{1}{2}\)+\(\dfrac{1}{6}+\dfrac{1}{12}\)+...+ \(\dfrac{1}{x\left(x+1\right)}\)=\(\dfrac{\sqrt{2012-x}+2012}{\sqrt{2012-x}+2013}\)
giải hpt sau:
\(\sqrt{x-2}+\sqrt{y+2012}+\sqrt{z-2013}=\dfrac{1}{2}\left(x+y+z\right)\)
Tìm giá trị của biểu thức: x\(^{2012}+2x^{2013}+3x^{2014}\)
Với x = \(\dfrac{\sqrt{\sqrt{5}+2}+\sqrt{\sqrt{5}-2}}{\sqrt{\sqrt{5}+1}}-\sqrt{3-2\sqrt{2}}\)
1.Chứng minh: \(\frac{1}{2\cdot\sqrt{1}}+\frac{1}{3\cdot\sqrt{2}}+\frac{1}{4\cdot\sqrt{3}}+...+\frac{1}{2012\cdot\sqrt{2011}}+\frac{1}{2013\cdot\sqrt{2012}}\)\(< 2\)
2.Chứng minh: A= \(\frac{1}{3\cdot\left(\sqrt{1}+\sqrt{2}\right)}+\frac{1}{5\cdot\left(\sqrt{2}+\sqrt{3}\right)}+...+\frac{1}{97\cdot\left(\sqrt{48}+\sqrt{49}\right)}\)\(< \frac{1}{2}\)
Câu 1: Cho biểu thức: P dfrac{sqrt{a}+2}{sqrt{a}+3}- dfrac{5}{a+sqrt{a}-6} + dfrac{1}{2-sqrt{a}} với a lớn hơn hoặc bằng 0, a # 4
a) Rút gọn P
b) Tìm a sao cho P 1
c) Tìm a để P sqrt{2012}
Câu 2: Cho biểu thức P dfrac{15sqrt{x}-11}{x+2sqrt{x}-3} + dfrac{3sqrt{x}-2}{1-sqrt{x}}- dfrac{2sqrt{x}+3}{sqrt{x}+3} với x lớn hơn hoặc bằng 0, x # 1
a) Rút gọn P
b) Tìm x để P dfrac{1}{2}
c) CMR: P nhỏ hơn hoặc bằng dfrac{2}{3}
Đọc tiếp
Câu 1: Cho biểu thức: P = \(\dfrac{\sqrt{a}+2}{\sqrt{a}+3}\)- \(\dfrac{5}{a+\sqrt{a}-6}\) + \(\dfrac{1}{2-\sqrt{a}}\) với a lớn hơn hoặc bằng 0, a # 4
a) Rút gọn P
b) Tìm a sao cho P < 1
c) Tìm a để P = \(\sqrt{2012}\)
Câu 2: Cho biểu thức P = \(\dfrac{15\sqrt{x}-11}{x+2\sqrt{x}-3}\) + \(\dfrac{3\sqrt{x}-2}{1-\sqrt{x}}\)- \(\dfrac{2\sqrt{x}+3}{\sqrt{x}+3}\) với x lớn hơn hoặc bằng 0, x # 1
a) Rút gọn P
b) Tìm x để P = \(\dfrac{1}{2}\)
c) CMR: P nhỏ hơn hoặc bằng \(\dfrac{2}{3}\)
Tính giá trị biểu thức sau:
M = \(\dfrac{1}{1+\sqrt{2}}+\dfrac{1}{\sqrt{2}+\sqrt{3}}+\dfrac{1}{\sqrt{3}+\sqrt{4}}+...+\dfrac{1}{\sqrt{2012}+\sqrt{2013}}\)
Tìm x biết:
a) \(\sqrt{x}\) < 3
b) \(\sqrt{4-x}\) ≤ 2
c) \(\sqrt{x+2}\) = \(\sqrt{4-x}\)
d) \(\sqrt{x^{2^{ }}-1}\) = x - 1
\(\sqrt{\dfrac{x+2}{4}}+\sqrt{25x+50}-2\sqrt{x+2}=14\) ; \(\sqrt{2x+3}=x\) ; \(\sqrt{25x^2+20x+4}=1\) ; \(\sqrt{\dfrac{x+1}{2x-1}}=2\) ; \(\dfrac{\sqrt{x-2}}{\sqrt{3x+1}}=6\)
Tìm x