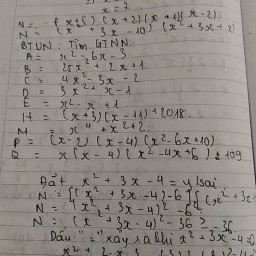ban đặt x2 -1 = a ta đc phương trình \(\sqrt{a}-a=0\) rồi giải tìm a, xong thay vào tìm x
\(\sqrt{x^2-1}-x^2+1=0\)
=>\(\sqrt{x^2-1}-x^2=0-1=-1\)
=>\(\sqrt{x^2-1}=-1+x^2=x^2-1\)
=>\(x^2-1=\left(x^2-1\right)^2\)
dùng bằng phương pháp thế , ta có
\(y=y^2\)
=>\(\left[{}\begin{matrix}y=0\\y=1\end{matrix}\right.\)
Thay vào biểu thức trên ta có :
\(\left[{}\begin{matrix}x^2-1=0\\x^2-1=1\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x^2=0+1=1\\x^2=1+1=2\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x=\sqrt{1}=1\\x=\sqrt{2}\end{matrix}\right.\)
Vậy x∈{1;\(\sqrt{2}\)}
( HS 6->7 làm bài , ko chắc chắn :))
ĐK: \(\left\{{}\begin{matrix}x\le-1\\x\ge1\end{matrix}\right.\)
Đặt \(x^2-1=a\left(a\ge0\right)\)
=> \(\sqrt{a}-a=0\)
=> \(\sqrt{a}\left(1-\sqrt{a}\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}\sqrt{a}=0\\1-\sqrt{a}=0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}a=0\\a=1\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x^2-1=0\\x^2-1=1\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=1\left(TMDK\right)\\x=\sqrt{2}\left(TMDK\right)\end{matrix}\right.\)
Vậy x=1 và x=căn2