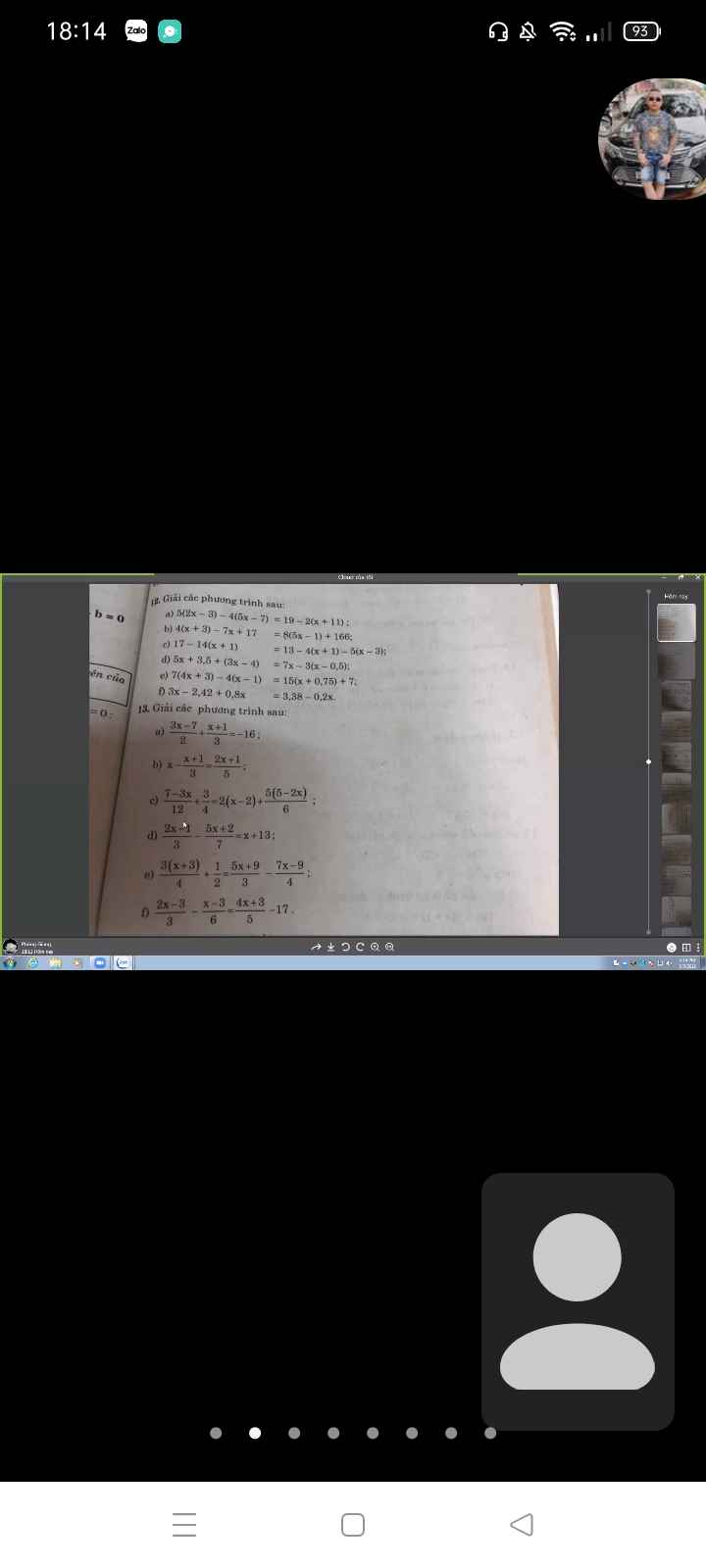
Bài 1:
a) Ta có: \(2x-3=4x+6\)
\(\Leftrightarrow2x-4x=6+3\)
\(\Leftrightarrow-2x=9\)
\(\Leftrightarrow x=-\dfrac{9}{2}\)
Vậy: \(S=\left\{-\dfrac{9}{2}\right\}\)
Bài 1:
b) Ta có: \(\dfrac{x+2}{4}-x+3-\dfrac{1-x}{8}=0\)
\(\Leftrightarrow\dfrac{2\left(x+2\right)}{8}+\dfrac{8\left(-x+3\right)}{8}+\dfrac{x-1}{8}=0\)
Suy ra: \(2x+4-8x-24+x-1=0\)
\(\Leftrightarrow-5x-21=0\)
\(\Leftrightarrow-5x=21\)
hay \(x=-\dfrac{21}{5}\)
Vậy: \(S=\left\{-\dfrac{21}{5}\right\}\)
Bài 1: bạn tự kết luận nghiệm nhé, vì đây là những phương trình cơ bản nên mình giải tắt chút
a, \(2x-3=4x+6\Leftrightarrow-2x=9\Leftrightarrow x=-\dfrac{9}{2}\)
b, \(\dfrac{x+2}{4}+3-\dfrac{1-x}{8}=0\)
\(\Leftrightarrow\dfrac{2x+4+24-1+x}{8}=0\Rightarrow3x+27=0\Leftrightarrow x=-3\)
c, \(x\left(x-1\right)+x\left(x+3\right)=0\Leftrightarrow x^2-x+x^2+3x=0\)
\(\Leftrightarrow2x^2+2x=0\Leftrightarrow2x\left(x+1\right)=0\Leftrightarrow x=0;x=-1\)
d, \(\dfrac{x}{2x-6}-\dfrac{x}{2x+2}=\dfrac{2x}{\left(x+1\right)\left(x-3\right)}\)ĐK : \(x\ne-1;3\)
\(\Leftrightarrow\dfrac{x}{2\left(x-3\right)}-\dfrac{x}{2\left(x+1\right)}=\dfrac{2x}{\left(x+1\right)\left(x-3\right)}\)
\(\Leftrightarrow\dfrac{x^2+x-x^2+3x}{2\left(x+1\right)\left(x-3\right)}=\dfrac{4x}{2\left(x+1\right)\left(x-3\right)}\Rightarrow0=0\)
Vậy phương trình có vô số nghiệm
Bài 2 :
a, \(2x-3>3\left(x-2\right)\Leftrightarrow2x-3>3x-6\)
\(\Leftrightarrow-x>-3\Leftrightarrow x< 3\)
Vậy tập nghiệm của bất phương trình là S = { x | x < 3 }
b, \(\dfrac{12x+1}{12}\le\dfrac{9x+1}{3}-\dfrac{8x+1}{4}\)
\(\Leftrightarrow\dfrac{12x+1}{12}\le\dfrac{36x+4}{12}-\dfrac{24x+3}{12}\Rightarrow12x+1\le12x+1\Leftrightarrow0=0\)
Vậy bất phương trình có vô số nghiệm