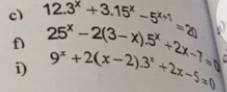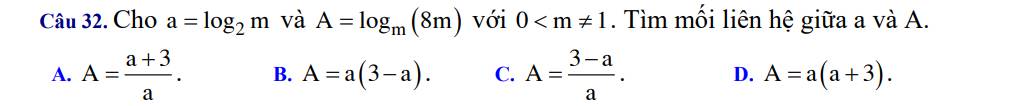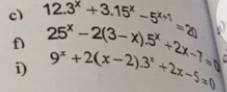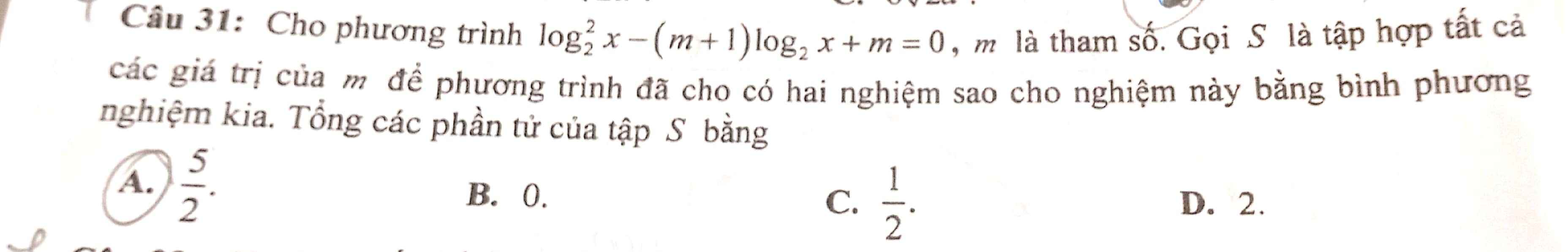Câu 64:
Ta có:
\(6^x+(3-m)2^x-m=0\)
\(\Leftrightarrow 6^x+3.2^x=m(1+2^x)\)
\(\Leftrightarrow \frac{6^x+3.2^x}{2^x+1}-m=0\)
Xét \(f(x)=\frac{6^x+3.2^x}{2^x+1}-m\) là một hàm liên tục. Để pt \(f(x)=0\) có nghiệm trong khoảng \((0;1)\Rightarrow f(0).f(1)< 0\)
\(\Leftrightarrow (2-m)(4-m)< 0\)
\(\Leftrightarrow 2< m< 4\Leftrightarrow m\in (2;4)\)
Đáp án C
Câu 65:
Ta có:
\(P=\log^2_{\frac{a}{b}}a^2+3\log_b\left(\frac{a}{b}\right)\)
\(\Leftrightarrow P=[2\log_{\frac{a}{b}}a]^2+3\log_b\left(\frac{a}{b}\right)\)
\(\Leftrightarrow P=4\log^2_{\frac{a}{b}}a+3(\log_ba-\log_bb)\)
\(\Leftrightarrow P=4\log^2_{\frac{a}{b}}a+3(\log_ba-1)\)
Biến đổi: \(\log_{\frac{a}{b}}a.\log_a\left(\frac{a}{b}\right)=1\)
\(\Rightarrow \log_{\frac{a}{b}}a=\frac{1}{\log_a\left(\frac{a}{b}\right)}=\frac{1}{\log_aa-\log_ab}=\frac{1}{1-\log_ab}\)
Do đó, \(P=\frac{4}{(1-\log_ab)^2}+3(\log_ba-1)\)
Đặt \(\log_ba=x\Rightarrow \log_ab=\frac{1}{x}\)
\(P=\frac{4x^2}{(x-1)^2}+3(x-1)\). Vì \(a>b>1\Rightarrow x>1\)
\(P'=\frac{3x^3-9x^2+x-3}{(x-1)^3}=0\)
\(\Leftrightarrow 3x^3-9x^2+x-3=0\Leftrightarrow x=3\)
Lập bảng biến thiên ta suy ra \(P_{\min}=P(3)=15\)
Đáp án D
Câu 66:
\(\log(mx)=2\log(x+1)\)
\(\Leftrightarrow \log(mx)=\log(x+1)^2\Leftrightarrow mx=(x+1)^2\)
\(\Leftrightarrow m=\frac{(x+1)^2}{x}\)
Để pt có nghiệm duy nhất thì đồ thị hàm số \(y=\frac{(x+1)^2}{x}\) cắt đường thẳng \(y=m\) tại một điểm duy nhất.
Xét \(y=\frac{(x+1)^2}{x}\Rightarrow y'=1-\frac{1}{x^2}=0\Leftrightarrow x=\pm 1\)
\(\Rightarrow x=1\) vì \(x\in (-1;+\infty)\)
Lập bảng biến thiên:

Từ bảng biến thiên suy ra để 2 đths cắt nhau tại duy nhất 1 điểm thì \(m<0\) hoặc \(m=4\)
\(\Rightarrow m=4\) hoặc \(m\in [-2017;-1]\)
Do đó có 2018 giá trị m thỏa mãn
Đáp án C
Bài 67:
Xem tại đây https://hoc24.vn/hoi-dap/question/483277.html