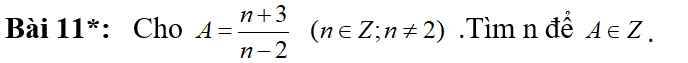\(\Rightarrow3x+2⋮x-3\Rightarrow\left(3x-9\right)+11⋮x-3\Rightarrow3\left(x-3\right)+11⋮x-3\Rightarrow11⋮x-3\)
\(\Rightarrow x-3\in\left\{-1;1;11;-11\right\}\)
\(\Rightarrow x\in\left\{2;4;14;-8\right\}\)
Vậy \(x\in\left\{2;4;14;-8\right\}\)
3x + 2 là bội của x - 3
⇒ \(\left[{}\begin{matrix}\text{3x + 2 ⋮ x - 3}\\\text{x - 3 ⋮ x - 3}\end{matrix}\right.\)
⇒ \(\left[{}\begin{matrix}\text{3x + 2 ⋮ x - 3}\\\text{3(x - 3) ⋮ x - 3}\end{matrix}\right.\)
3x + 2 ⋮ 3(x - 3)
Do đó ta có 3x + 2 = 3(x - 3) + 11
Vậy x - 3 ∈ Ư(11) = {-1; 1; -11; 11}
Ta có bảng sau :
| x - 3 | -1 | 1 | -11 | 11 |
| x | 2 | 4 | -8 | 14 |
➤ Vậy x ∈ {2; 4; -8; 14}