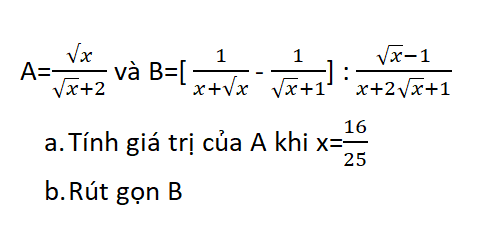a: Ta có: \(A=\dfrac{5\sqrt{x}}{\sqrt{x}-2}+\dfrac{3-\sqrt{x}}{\sqrt{x}+2}+\dfrac{6x}{4-x}\)
\(=\dfrac{5\sqrt{x}\left(\sqrt{x}+2\right)+\left(3-\sqrt{x}\right)\left(\sqrt{x}-2\right)-6x}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{5x+10\sqrt{x}+3\sqrt{x}-6-x+2\sqrt{x}-6x}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{15\sqrt{x}-6}{x-4}\)
b: Ta có: \(B=\left(\dfrac{15-\sqrt{x}}{x-25}+\dfrac{2}{\sqrt{x}+5}\right):\dfrac{\sqrt{x}+1}{\sqrt{x}-5}\)
\(=\dfrac{15-\sqrt{x}+2\sqrt{x}-10}{\left(\sqrt{x}+5\right)\left(\sqrt{x}-5\right)}\cdot\dfrac{\sqrt{x}-5}{\sqrt{x}+1}\)
\(=\dfrac{1}{\sqrt{x}+1}\)
c: Ta có: \(C=\dfrac{\sqrt{x}+2}{x-5\sqrt{x}+6}+\dfrac{\sqrt{x}+3}{\sqrt{x}-2}+\dfrac{\sqrt{x}+2}{3-\sqrt{x}}\)
\(=\dfrac{\sqrt{x}+2+x-9-x+4}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{\sqrt{x}-3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}-2\right)}=\dfrac{1}{\sqrt{x}-2}\)