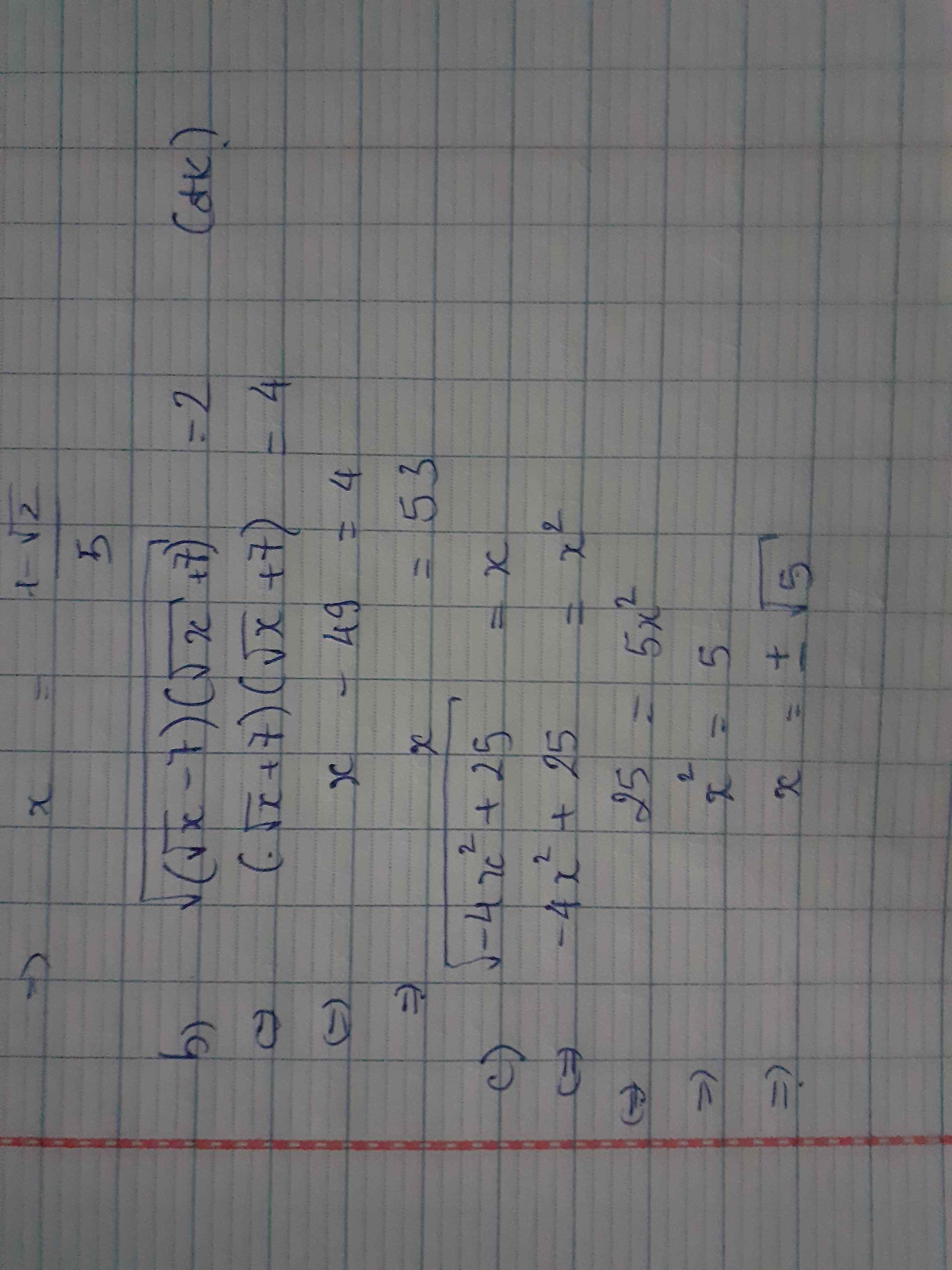1. ĐKXĐ: $x\geq \frac{-3}{5}$
PT $\Leftrightarrow 5x+3=3-\sqrt{2}$
$\Leftrightarrow x=\frac{-\sqrt{2}}{5}$
2. ĐKXĐ: $x\geq \sqrt{7}$
PT $\Leftrightarrow (\sqrt{x}-7)(\sqrt{x}+7)=4$
$\Leftrightarrow x-49=4$
$\Leftrightarrow x=53$ (thỏa mãn)
3. ĐKXĐ: $\frac{5}{2}\geq x\geq \frac{-5}{2}$
PT \(\Rightarrow \left\{\begin{matrix} x\geq 0\\ -4x^2+25=x^2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq 0\\ x^2=5\end{matrix}\right.\)
\(\Rightarrow x=\sqrt{5}\) (thỏa mãn)
1)
ĐKXĐ: \(x\ge-\dfrac{3}{5}\)
Ta có: \(\sqrt{5x+3}=\sqrt{3-\sqrt{2}}\)
\(\Leftrightarrow5x+3=3-\sqrt{2}\)
\(\Leftrightarrow5x=-\sqrt{2}\)
hay \(x=\dfrac{-\sqrt{2}}{5}\)(nhận)
Vậy: \(S=\left\{-\dfrac{\sqrt{2}}{5}\right\}\)
2)
ĐKXĐ: \(x\ge0\)
Ta có: \(\sqrt{\left(\sqrt{x}-7\right)\left(\sqrt{x}+7\right)}=2\)
\(\Leftrightarrow\sqrt{x-49}=2\)
\(\Leftrightarrow x-49=4\)
hay x=53(thỏa ĐK)
3) Ta có: \(\sqrt{-4x^2+25}=x\)
\(\Leftrightarrow-4x^2+25=x^2\)
\(\Leftrightarrow-4x^2-x^2=-25\)
\(\Leftrightarrow x^2=5\)
hay \(x\in\left\{\sqrt{5};-\sqrt{5}\right\}\)
Vậy: \(S=\left\{\sqrt{5};-\sqrt{5}\right\}\)