Question

Answer 1

ĐKXĐ: x>=0

Answer 2

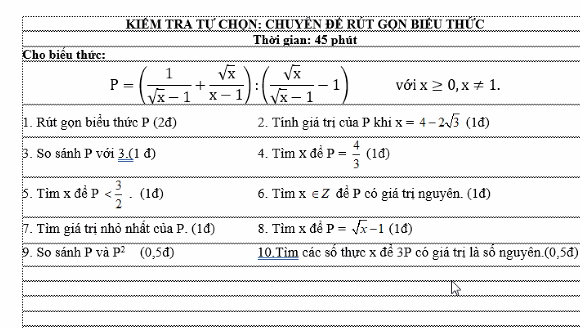
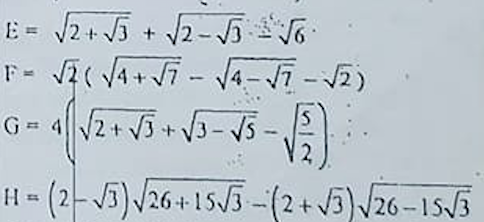ĐK: \(x\ge0\)

\(D=\left(\dfrac{1}{\sqrt{x}+1}-\dfrac{1}{x+\sqrt{x}}\right):\dfrac{x-\sqrt{x}+1}{x\sqrt{x}+1}\\ =\dfrac{\sqrt{x}-1}{\sqrt{x}\left(\sqrt{x}+1\right)}:\dfrac{x-\sqrt{x}+1}{\left(\sqrt{x}+1\right)\left(x-\sqrt{x}+1\right)}\\ =\dfrac{\sqrt{x}-1}{\sqrt{x}\left(\sqrt{x}+1\right)}:\dfrac{1}{\sqrt{x}+1}\\ =\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+1\right)}=\dfrac{\sqrt{x}-1}{\sqrt{x}}\)