Question

Answer 1

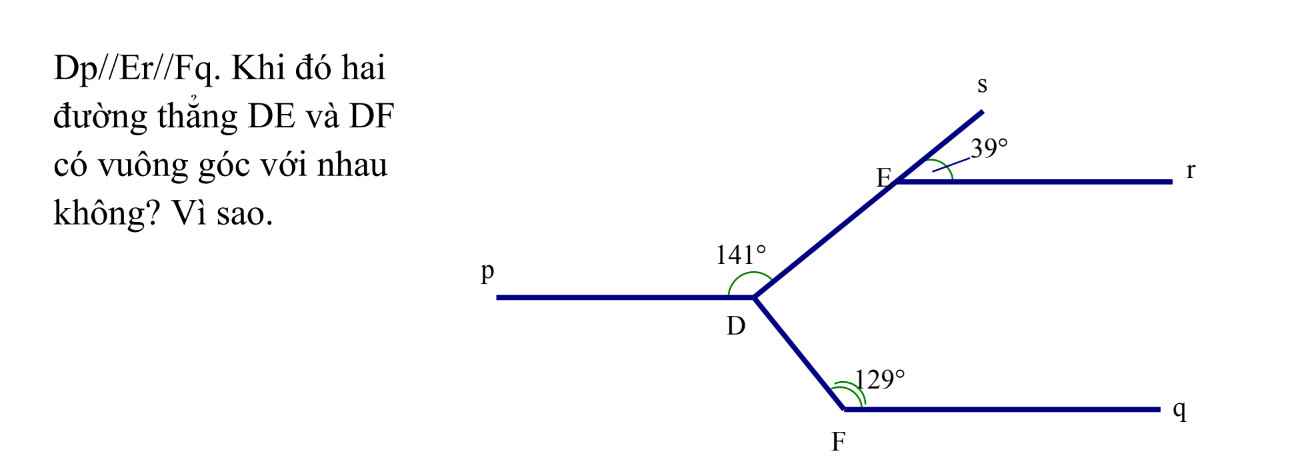
Lời giải:
Lấy tia $Dp'$ là tia đối của $Dp$. Theo đề thì $Dp'\parallel Er\parallel Fq$

$\widehat{EDp'}=\widehat{sEr}=39^0$ (2 góc đồng vị)

$\widehat{FDp'}=180^0-\widehat{DFq}=180^0-129^0=51^0$ (hai góc trong cùng phía)

$\Rightarrow \widehat{EDF}=\widehat{EDp'}+\widehat{FDp'}=39^0+51^0=90^0$ 

$\Rightarrow DE\perp DF$

Answer 2

Hình vẽ: