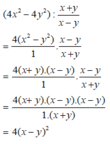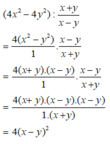4x2-8xy+4y2

Những câu hỏi liên quan
Bài 1: Phân tích đa thức thành nhân tử: a) 4y3 + 16y2 + 16y b) 8x2-48x+6xy-36y c) 8x2-48x-6xy+36y d) a2 –2ab+b2 –4 e) 4–x2 –4xy–4y2 f) 8a2 –16a+8ax–16x g) 16–4x2 +8xy–4y2 h) –4x2 –16xy–16y2 Bài 2: Tìm x, biết: a) x3 – 6x2 + 9x = 0 b) 5x(x–6)+3x–18=0 c) 5x(x – 6) – 18 + 3x = 0 d) 5x(x – 6) – 3x + 18 = 0 e) (2x – 3)2 = (5 – x)2 f) (2x + 1)2 = (3x – 2)2 g) 16(2x–3)=-25x2 (3–2x)
b: \(8x^2-48x+6xy-36y\)
\(=8x\left(x-6\right)+6y\left(x-6\right)\)
\(=2\left(x-6\right)\left(4x+3y\right)\)
d: \(a^2-2ab+b^2-4\)
\(=\left(a-b\right)^2-4\)
\(=\left(a-b-2\right)\left(a-b+2\right)\)
Đúng 0
Bình luận (0)
Viết biểu thức sau dưới dạng tổng của hai bình phương:
a. x2-2x+2+4y2+4y
b. 4x2+y2+12x+4y+13
c. x2+17+4y2+8x+4y
d. 4x2-12x+y2-4y+13
`a)x^2-2x+2+4y^2+4y`
`=x^2-2x+1+4y^2+4y+1`
`=(x-1)^2+(2y+1)^2`
`b)4x^2+y^2+12x+4y+13`
`=4x^2+12x+9+y^2+4y+4`
`=(2x+3)^2+(y+2)^2`
`c)x^2+17+4y^2+8x+4y`
`=x^2+8x+16+4y^2+4y+1`
`=(x+4)^2+(2y+1)^2`
`d)4x^2-12xy+y^2-4y+13`
`=4x^2-12x+9+y^2-4y+4`
`=(2x-3)^2+(y-2)^2`
Đúng 4
Bình luận (0)
a) \(x^2-2x+2+4y^2+4y=\left(x-1\right)^2+\left(2y+1\right)^2\)
b) \(4x^2+y^2+12x+4y+13=\left(2x+3\right)^2+\left(y+2\right)^2\)
c) \(x^2+17+4y^2+8x+4y=\left(x+4\right)^2+\left(2y+1\right)^2\)
d) \(4x^2-12x+y^2-4y+13=\left(2x-3\right)^2+\left(y-2\right)^2\)
Đúng 0
Bình luận (0)
a: \(x^2-2x+2+4y^2+4y\)
\(=x^2-2x+1+4y^2+4y+1\)
\(=\left(x-1\right)^2+\left(2y+1\right)^2\)
b: \(4x^2+12x+y^2+4y+13\)
\(=4x^2+12x+9+y^2+4y+4\)
\(=\left(2x+3\right)^2+\left(y+2\right)^2\)
c: \(x^2+8x+4y^2+4y+17\)
\(=x^2+8x+16+4y^2+4y+1\)
\(=\left(x+4\right)^2+\left(2y+1\right)^2\)
d: \(4x^2-12x+y^2-4y+13\)
\(=4x^2-12x+9+y^2-4y+4\)
\(=\left(2x-3\right)^2+\left(y-2\right)^2\)
Đúng 0
Bình luận (0)
4x2 + 8x + 4 - 4y2
\(4x^2+8x+4-4y^2\)
\(=\left(4x^2+8x+4\right)-4y^2\)
\(=\left(2x+2\right)^2-\left(2y\right)^2\)
\(=\left(2x+2-2y\right)\left(2x+2+2y\right)\)
\(=2\cdot\left(x-y+1\right)\cdot2\cdot\left(x+y+1\right)\)
\(=4\left(x-y+1\right)\left(x+y+1\right)\)
Đúng 3
Bình luận (0)
Thực hiện phép tính:
4
x
2
-
4
y
2
:
x
+
y
x
-
y
.
A.
4
x
-
y
B.
4
x...
Đọc tiếp
Thực hiện phép tính: 4 x 2 - 4 y 2 : x + y x - y .
A. 4 x - y
B. 4 x x - y
C. 4 x + y 2
D. 4 x - y 2
Thực hiện phép tính:
4
x
2
-
4
y
2
:
x
+
y
x
-
y
.
A.
4
x
-
y
B.
4
x...
Đọc tiếp
Thực hiện phép tính: 4 x 2 - 4 y 2 : x + y x - y .
A. 4 x - y
B. 4 x x - y
C. 4 x + y 2
D. 4 x - y 2
tìm x;y
a) 4x2+13y+12xy−18y−4x+104x2+13y+12xy−18y−4x+10
b) 4x2+12xy+9y2+4y2−18y−4x+104x2+12xy+9y2+4y2−18y−4x+10
c) (2x+3y)2−2(2x+3y)+1+4y2−12y+9(2x+3y)2−2(2x+3y)+1+4y2−12y+9
d) (2x+3y−1)+(2y−3)2=0
Phân tích các đa thức sau thành nhân tử:
e/ x2−4y2−2x+4yx2−4y2−2x+4y
f/ x2−25−2xy+y2x2−25−2xy+y2
g/ x3−2x2+x−xy2x3−2x2+x−xy2
h/ x3−4x2−12x+27
h: \(=\left(x+3\right)\cdot\left(x^2-3x+9\right)-4x\left(x+3\right)\)
\(=\left(x+3\right)\left(x^2-7x+9\right)\)
Đúng 1
Bình luận (0)
16x2 - 8xy +y2 + 1
-4x2 + 2x -1
\(16x^2-8xy+y^2+1=\left(4x-y\right)^2+1\ge1\)
Dấu \("="\Leftrightarrow4x=y\)
\(-4x^2+2x-1=-\left(4x^2-2\cdot2\cdot\dfrac{1}{2}x+\dfrac{1}{16}\right)-\dfrac{15}{16}=-\left(2x-\dfrac{1}{4}\right)^2-\dfrac{15}{16}\le-\dfrac{15}{16}\)
Dấu \("="\Leftrightarrow2x=\dfrac{1}{4}\Leftrightarrow x=\dfrac{1}{8}\)
Đúng 1
Bình luận (0)
a) A = x2 - 4y2 + 2x + 4y
b) A = 4x2 - 9y2 - 4x - 6y
c) A = 3x2 - 3xy - 5x + 5y
a) A = x2 - 4y2 + 2x + 4y = (x-2y)(x+2y)+2(x+2y)=(x+2y)(x-2y+2)
b) A = 4x2 - 9y2 - 4x - 6y=(2x-3y)(2x+3y)-2(2x+3y)=(2x+3y)(2x-3y-2)
c) A = 3x2 - 3xy - 5x + 5y=3x(x-y)-5(x-y)=(x-y)(3x-5)
Đúng 0
Bình luận (0)
a) \(A=x^2-4y^2+2x+4y=\left(x-2y\right)\left(x+2y\right)+2\left(x+2y\right)=\left(x+2y\right)\left(x-2y+2\right)\)
b) \(A=4x^2-9y^2-4x-6y=\left(2x-3y\right)\left(2x+3y\right)-2\left(2x+3y\right)=\left(2x+3y\right)\left(2x-3y-2\right)\)
c) \(A=3x^2-3xy-5x+5y=3x\left(x-y\right)-5\left(x-y\right)=\left(x-y\right)\left(3x-5\right)\)
Đúng 1
Bình luận (0)