Số nghiệm của phương trình:
\(\sqrt{x-512}+\sqrt{1024-x}=16+4\sqrt[8]{\left(x-512\right)\left(1024-x\right)}\)
Phương trình x − 512 + 1024 − x = 16 + 4 x − 512 1024 − x 8 có bao nhiêu nghiệm?
A. 2 nghiệm
B. 8 nghiệm
C. 4 nghiệm
D. 3 nghiệm
Đáp án D
Lời giải:
Đặt t = x − 512 1024 − x 8 ≥ 0 , ta có
t 4 = x − 512 1024 − x ≤ x − 512 + 1024 − x 2 = 256 ⇒ 0 ≤ t ≤ 4
Với t = 4 thì ta tìm được 1 giá trị của x = 768
Với 0 ≤ t ≤ 4 thì ta tìm được 2 giá trị của x (Khi đó phương trình của Định lý Viét đảo có 2 nghiệm phân biệt)
Bình phương 2 vế phương trình đã cho, ta được
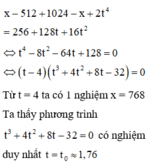
(sử dụng máy tính).
Từ đó ta có 2 nghiệm x thỏa mãn
Do đó phương trình đã cho có 3 nghiệm.
Phương trình x - 512 + 1024 - x = 16 + 4 x - 512 1024 - x 8 có bao nhiêu nghiệm?
A. 4.
B. 2.
C. 3.
D. 8.
Phương trình x - 512 + 1024 - x = 16 + 4 x - 512 1024 - x 8 có bao nhiêu nghiệm?
A. 4 nghiệm
B. 2 nghiệm
C. 3 nghiệm
D. 8 nghiệm
Đáp án C
x - 512 + 1024 - x = 16 + 4 x - 512 1024 - x 8 (*), 512 ≤ x ≤ 1024
t = x - 512 1024 - x 8 ⇒ t 4 = x - 512 1024 - x ≤ x - 512 + 1024 - x 2 = 256 ⇒ 0 ≤ t ≤ 4 t = 4 ⇒ x = 768 0 ≤ t ≤ 4
=> Bình phương hai vế (*):
( t - 4 ) t 3 + 4 t 2 + 8 t - 32 = 0 ⇔ [ x ≈ 512 , 18 x ≈ 1023 , 82
Phương trình x − 512 + 1024 − x = 16 + 4 x − 512 1024 − x 8 có bao nhiêu nghiệm?
A. 4 nghiệm
B. 2 nghiệm
C. 3 nghiệm
D. 8 nghiệm
Đáp án C
x − 512 + 1024 − x = 16 + 4 ( x − 512 ) ( 1024 − x ) 8 ( * ) , ( 512 ≤ x ≤ 1024 ) t = ( x − 512 ) ( 1024 − x ) 8 ⇒ t 4 = ( x − 512 ) ( 1024 − x ) ≤ x − 512 + 1024 − x 2 = 256 ⇒ 0 ≤ t ≤ 4 ⋅ t = 4 ⇒ x = 768
0 ≤ t < 4 ⇒ bình phương 2 vế (*):
⇒ ( t − 4 ) ( t 3 + 4 t 2 + 8 t − 32 ) = 0 ⇔ t ≈ 1 , 76 ⇔ x ≈ 512 , 18 x ≈ 1023 , 82
Phương trình \(\sqrt{2-f\left(x\right)}=f\left(x\right)\) có tập nghiệm A = {1;2;3}. Phương trình \(\sqrt{2.g\left(x\right)-1}+\sqrt[3]{3.g\left(x\right)-2}=2.g\left(x\right)\) có tập nghiệm là B = {0;3;4;5} . Hỏi tập nghiệm của phương trình \(\sqrt{f\left(x\right)-1}+\sqrt{g\left(x\right)-1}+f\left(x\right).g\left(x\right)+1=f\left(x\right)+g\left(x\right)\)
có bao nhiêu phần tử?
A.1
B.4 C.6 D.7
\(\sqrt{2-f\left(x\right)}=f\left(x\right)\Leftrightarrow\left\{{}\begin{matrix}f\left(x\right)\ge0\\f^2\left(x\right)+f\left(x\right)-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}f\left(x\right)=1\\f\left(x\right)=-2< 0\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow f\left(1\right)=f\left(2\right)=f\left(3\right)=1\)
\(\sqrt{2g\left(x\right)-1}+\sqrt[3]{3g\left(x\right)-2}=2.g\left(x\right)\)
\(VT=1.\sqrt{2g\left(x\right)-1}+1.1\sqrt[3]{3g\left(x\right)-2}\)
\(VT\le\dfrac{1}{2}\left(1+2g\left(x\right)-1\right)+\dfrac{1}{3}\left(1+1+3g\left(x\right)-2\right)\)
\(\Leftrightarrow VT\le2g\left(x\right)\)
Dấu "=" xảy ra khi và chỉ khi \(g\left(x\right)=1\)
\(\Rightarrow g\left(0\right)=g\left(3\right)=g\left(4\right)=g\left(5\right)=1\)
Để các căn thức xác định \(\Rightarrow\left\{{}\begin{matrix}f\left(x\right)-1\ge0\\g\left(x\right)-1\ge0\end{matrix}\right.\)
Ta có:
\(\sqrt{f\left(x\right)-1}+\sqrt{g\left(x\right)-1}+f\left(x\right).g\left(x\right)-f\left(x\right)-g\left(x\right)+1=0\)
\(\Leftrightarrow\sqrt{f\left(x\right)-1}+\sqrt{g\left(x\right)-1}+\left[f\left(x\right)-1\right]\left[g\left(x\right)-1\right]=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}f\left(x\right)=1\\g\left(x\right)=1\end{matrix}\right.\) \(\Leftrightarrow x=3\)
Vậy tập nghiệm của pt đã cho có đúng 1 phần tử
Cho phương trình -8(\(\sqrt{x+1}+\sqrt{8-x}\) ) + \(2\sqrt{\left(x+1\right)\left(8-x\right)}-2m=0\) tìm m để phương trình có nghiệm
Tính nhanh:
\(\frac{\left(1+2+4+8+...+512\right)x\left(101x102-101x101-50-51\right)}{2+4+8+16+...+1024+2048}\)
Bài làm:
Xét: \(101\times102-101\times101-50-51=101\times\left(102-101\right)-101=101\times1-101=0\)
\(\Rightarrow\left(1+2+4+8+...+512\right)\times\left(101\times102-101\times101-50-51\right)=0\)
\(\Rightarrow\frac{\left(1+2+4+8+...+512\right)\times\left(101\times102-101\times101-50-51\right)}{2+4+8+16+...+1024+2048}=0\)
Học tốt!!!!
tìm m để các phương trình sau có nghiệm
\(\sqrt{1+x}+\sqrt{8-x}+\sqrt{\left(1+x\right)\left(8-x\right)}\)
Để pt có nghiệm thì
\(1+x\ne0\) và \(8-x\ne0\)
\(\Rightarrow x\ne-1\) và \(x\ne8\)
\(\sqrt{1+x} +\sqrt{8-x}+\sqrt{\left(1+x\right)\left(8-x\right)}=m\)
( mk viết thiếu đề)
Để pt có nghiệm thì
1+x≠01+x≠0 và 8−x≠08−x≠0
⇒x≠−1⇒x≠−1 và x≠8
Cho xin một like đi các dân chơi à.
tìm m để phương trình sau có nghiệm
\(\sqrt{1+x}+\sqrt{8-x}+\sqrt{\left(1+x\right)\left(8-x\right)}=m\)
Vì $\sqrt{1+x}\ge 0,\sqrt{8-x}\ge 0,\sqrt{(1+x)(8-x)}\ge 0$
$\to \sqrt{1+x}+\sqrt{8-x}+\sqrt{(1+x)(8-x)}\ge 0$
mà $\sqrt{1+x}+\sqrt{8-x}+\sqrt{(1+x)(8-x)}=m$
=> m≥0
Đặt :
\(t=\sqrt{1+x}+\sqrt{8-x}\) \(\left(t\ge0\right)\)
DKXĐ : \(-1\le x\le8\)
\(\Leftrightarrow t^2=9+2\sqrt{\left(1+x\right)\left(8-x\right)}\) (1)
BBT của \(t^2\) :
| \(x\) | \(-1\) \(0\) \(8\) |
| \(t^2\) | \(9+2\sqrt{2}\) \(9\) \(9\) |
| \(t\) | \(1+2\sqrt{2}\) \(1\) \(2\sqrt{2}\) |
\(\Leftrightarrow t\in\left(1,2\sqrt{2}\right)\)
Thay \(\left(1\right)\) vào pt ta có :\(\Leftrightarrow\sqrt{\left(1+x\right)\left(8-x\right)}=\dfrac{t^2-9}{2}\) (1)
\(\Leftrightarrow f\left(t\right)=t^2+2t-9=2m\)
BBT của \(f\left(t\right)\) :
| \(t\) | \(1\) \(2\sqrt{2}\) |
| \(f\left(t\right)\) | \(4\sqrt{2}-1\) \(-6\) |
\(\Leftrightarrow2m\in\left[-6;4\sqrt{2}-1\right]\) thì pt có nghiệm
\(\Leftrightarrow m\in\left(-3;\dfrac{-1+4\sqrt{2}}{2}\right)\)
Vẽ dùm mình mấy cái mũi tên trên BBT nhé UwU
Giải phương trình: \(\left(\sqrt{4x^4-12x^3+9x^2+16}-2x^2+3x\right)\left(\sqrt{x+3}+\sqrt{x-1}\right)=8\)
ĐKXĐ: \(x\ge1\).
Phương trình đã cho tương đương:
\(\sqrt{x+3}+\sqrt{x-1}=\dfrac{8}{\sqrt{4x^4-12x^3+9x^2+16}-\left(2x^2-3x\right)}\)
\(\Leftrightarrow\sqrt{x+3}+\sqrt{x-1}=\dfrac{\sqrt{4x^4-12x^3+9x^2+16}+\left(2x^2-3x\right)}{2}\)
\(\Leftrightarrow\sqrt{4x^4-12x^3+9x^2+16}+\left(2x^2-3x\right)-2\sqrt{x+3}-2\sqrt{x-1}=0\)
\(\Leftrightarrow\left(\sqrt{4x^4-12x^3+9x^2+16}-2\sqrt{x+3}\right)+\left(2x^2-3x-2\sqrt{x-1}\right)=0\)
\(\Leftrightarrow\dfrac{4x^4-12x^3+9x^2-4x+4}{\sqrt{4x^4-12x^3+9x^2+16}+2\sqrt{x+3}}+\dfrac{4x^4-12x^3+9x^2-4x+4}{2x^2-3x+2\sqrt{x-1}}=0\)
\(\Leftrightarrow\left(x-2\right)\left(4x^3-4x^2+x-2\right)\left(\dfrac{1}{\sqrt{4x^4-12x^3+9x^2+16}+2\sqrt{x+3}}+\dfrac{1}{2x^2-3x+2\sqrt{x-1}}\right)=0\).
Do \(x\ge1\) nên ta có \(\dfrac{1}{\sqrt{4x^4-12x^3+9x^2+16}+2\sqrt{x+3}}+\dfrac{1}{2x^2-3x+2\sqrt{x-1}}>0\).
Do đó \(\left[{}\begin{matrix}x-2=0\Leftrightarrow x=2\left(TMĐK\right)\\4x^3-4x^2+x-2=0\left(1\right)\end{matrix}\right.\).
Giải phương trình bậc 3 ở (1) ta được \(x=\dfrac{\sqrt[3]{36\sqrt{13}+53\sqrt{6}}}{\sqrt[6]{279936}}+\dfrac{1}{\sqrt[6]{7776}\sqrt[3]{36\sqrt{13}+53\sqrt{6}}}+\dfrac{1}{3}\approx1,157298106\left(TMĐK\right)\).
Vậy...
Vì trong bài làm của mình có một số dòng khá dài nên bạn có thể vào trang cá nhân của mình để đọc tốt hơn!