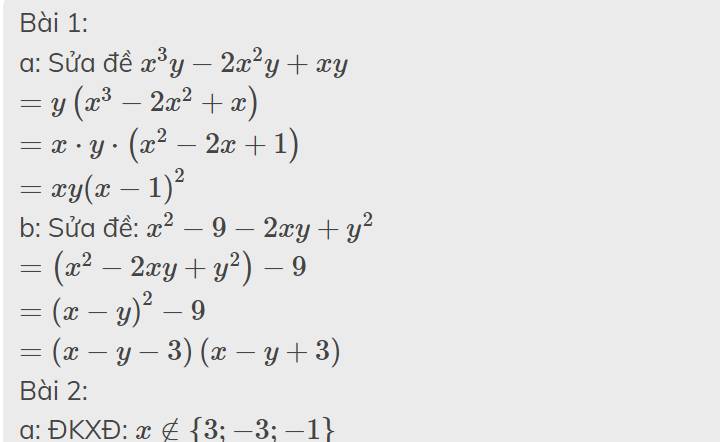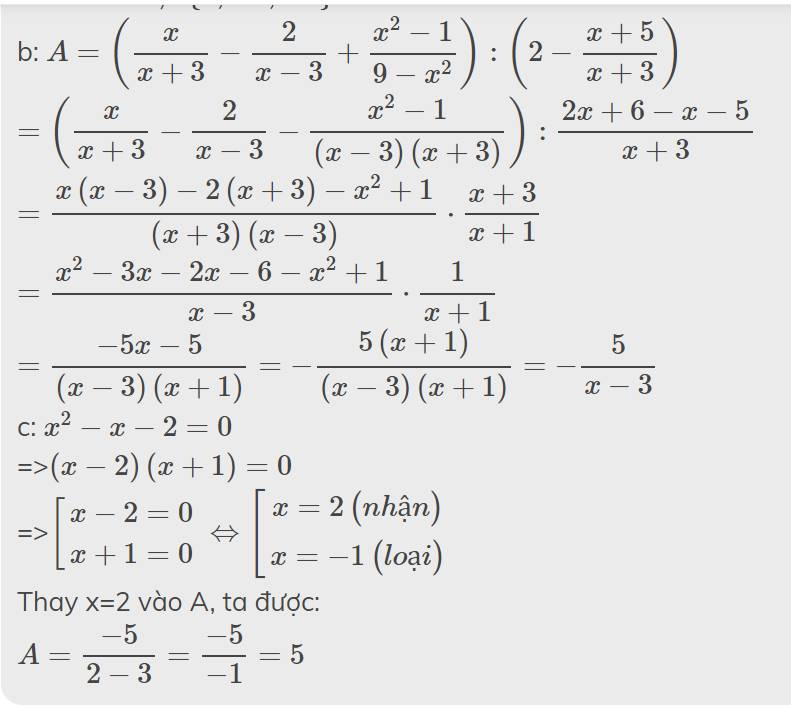Những câu hỏi liên quan
Cho biểu thức B = \(\left(\frac{x+3}{x-3}+\frac{2x^2-6}{9-x^2}+\frac{x}{x+3}\right):\frac{6x-12}{2x^2-18}\)
a. Tìm tập xác định và rút gọn B
b. Tìm giá trị của B với |x+1 | = 2
c. Tìm giá trị nguyên của x để B nhận giá trị nguyên.
a) tính gtrị của biểu thức A = \(\sqrt{3}+\sqrt{12}-\sqrt{27}-\sqrt{36}\)
b) cho bt B = \(\dfrac{2}{\sqrt{x-1}}-\dfrac{1}{\sqrt{x}}+\dfrac{3\sqrt{x-5}}{\sqrt{x\left(\sqrt{x-1}\right)}}\) với x > 0 và x \(\ne\) 1 . rút gọn bt và tìm x để B = 2
\(a,\) ta có :
\(\Leftrightarrow\left\{{}\begin{matrix}A=\sqrt{3}+\sqrt{2^2.3}-\sqrt{3^2.3}-\sqrt{6^2}\\A=\sqrt{3}+2\sqrt{3}-3\sqrt{3}-6\\A=\sqrt{3}.\left(1+2-3\right)-6\\A=-6\end{matrix}\right.\)
\(\Rightarrow A=-6\) . vậy \(A=9\sqrt{5}\)
__________________________________________________________
\(b,\) với \(x>0\) và \(x\ne1\) . ta có :
\(B=\dfrac{2}{\sqrt{x}-1}-\dfrac{1}{\sqrt{x}}+\dfrac{3\sqrt{x}-5}{\sqrt{x}\left(\sqrt{x}-1\right)}\)
\(\Leftrightarrow B=\dfrac{2\sqrt{x}-\left(\sqrt{x}-1\right)+3\sqrt{x}-5}{\sqrt{x}\left(\sqrt{x}-1\right)}\)
\(\Leftrightarrow B=\dfrac{2\sqrt{x}-\sqrt{x}+1+3\sqrt{x}-5}{\sqrt{x}\left(\sqrt{x}-1\right)}\)
\(\Leftrightarrow B=\dfrac{4\sqrt{x}-4}{\sqrt{x}\left(\sqrt{x}-1\right)}\)
\(\Leftrightarrow\) \(B=\dfrac{4\left(\sqrt{x}-1\right)}{\sqrt{x}\left(\sqrt{x}-1\right)}\)
\(\Leftrightarrow B=\dfrac{4}{\sqrt{x}}\)
vậy với \(x>0\) \(;\) \(x\ne1\) thì \(B=\dfrac{4}{\sqrt{x}}\)
để \(B=2\) thì \(\dfrac{4}{\sqrt{x}}=2\Leftrightarrow\sqrt{x}=2\Leftrightarrow x=4\left(tm\right)\)
vậy để \(B=2\) thì \(x=4\)
Đúng 4
Bình luận (1)
Cho biểu thức B = \(\left(\frac{x+3}{x-3}+\frac{2\chi^2-6}{9-\chi^2}+\frac{\chi}{\chi+3}\right):\frac{6\chi-12}{2\chi^2-18}\)
a) tìm tập xác định và rút gọn biểu thức
b) Tìm giá trị của B với x=1 hoặc x= -3
c) tìm giá trị nguyên của x để B nhận giá trị nguyên
a, B=[(x+3)/(x-3)+(2x^2-6)/(9-x^2)+x/(x+3)]:[(6x-12)/(2x^2-18)]
=[(x+3)/(x-3)+ -(2x^2-6)/(x^2-9)+x/(x+3)]:[(6x-12)/(2x^2-18)]
=[(x+3)/(x-3)+ -(2x^2-6)/(x-3)(x+3)+x/(x+3)]:[(6x-12)/2(x-3)(x+3)]
={[(x+3)^2-2x^2+6+x(x-3)]/(x-3)(x+3)}:[6(x-2)/2(x-3)(x+3)]
=(x^2+6x+9-2x^2+6+x^2-3x)/(x-3)(x+3): 6(x-2)/2(x-3)(x+3)
=3x+15/(x-3)(x+3): 6(x-2)/2(x-3)(x+3)
=3(x+5)/(x-3)(x+3): 6(x-2)/2(x-3)(x+3
=3(x+5)/(x-3)(x+3).2(x-3)(x+3)/6(x-2)
=3(x+5).6/(x-2)
=6(x+5)/6(x-2)
=x+5/x-2
b,Ta thay : x=1
=>x+5/x-2=1+5/1-2=-6
Ta thay : x=-3
=>x+5/x-2=-3+5/-3-2=-2/5
c, Ta co : x+5/x-2=0
x+5=(x-2).0
x+5=0
x=-5
Vậy : x=-5
Đúng 0
Bình luận (0)
Cho biểu thức P= ( 2x-1/x+3 - x/3-x - 3-10x/x^2-9) : x+2/x-3
A) rút gọn P và tìm điều kiện xác định của P
B) tính giá trị của P khi x^2-7x+12=0
C) tìm các giá trị nguyên của x để P có giá trị nguyên dương
Xem chi tiết
a) ĐKXĐ: \(x\notin\left\{3;-3;-2\right\}\)
Ta có: \(P=\left(\dfrac{2x-1}{x+3}-\dfrac{x}{3-x}-\dfrac{3-10x}{x^2-9}\right):\dfrac{x+2}{x-3}\)
\(=\left(\dfrac{\left(2x-1\right)\left(x-3\right)}{\left(x+3\right)\left(x-3\right)}+\dfrac{x\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}-\dfrac{3-10x}{\left(x-3\right)\left(x+3\right)}\right):\dfrac{x+2}{x-3}\)
\(=\dfrac{2x^2-6x-x+3+x^2+3x-3+10x}{\left(x-3\right)\left(x+3\right)}:\dfrac{x+2}{x-3}\)
\(=\dfrac{3x^2+6x}{\left(x-3\right)\left(x+3\right)}:\dfrac{x+2}{x-3}\)
\(=\dfrac{3x\left(x+2\right)}{\left(x-3\right)\left(x+3\right)}\cdot\dfrac{x-3}{x+2}\)
\(=\dfrac{3x}{x+3}\)
b) Ta có: \(x^2-7x+12=0\)
\(\Leftrightarrow x^2-3x-4x+12=0\)
\(\Leftrightarrow x\left(x-3\right)-4\left(x-3\right)=0\)
\(\Leftrightarrow\left(x-3\right)\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3=0\\x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\left(loại\right)\\x=4\left(nhận\right)\end{matrix}\right.\)
Thay x=4 vào biểu thức \(P=\dfrac{3x}{x+3}\), ta được:
\(P=\dfrac{3\cdot4}{4+3}=\dfrac{12}{7}\)
Vậy: Khi \(x^2-7x+12=0\) thì \(P=\dfrac{12}{7}\)
Đúng 6
Bình luận (0)
BÀI 1: pttnt
a) x^3y-2x^y+xy b) x^2-9-4xy+y^2
bài 2 cho biểu thức 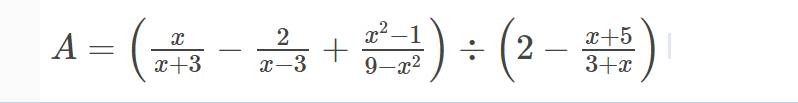
a) Tìm đk xác định của bt A
b) rút gọn bt A
c) tính giá trị của biểu thức A biết x^2-x-2=0
BÀI 1: pttnt
a) x^3y-2x^y+xy b) x^2-9-4xy+y^2
bài 2 cho biểu thức 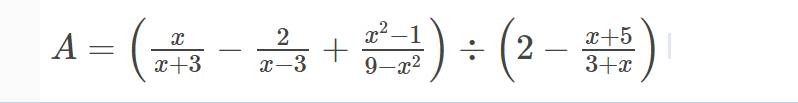
a) Tìm đk xác định của bt A
b) rút gọn bt A
c) tính giá trị của biểu thức A biết x^2-x-2=0
Bài 1:
a: Sửa đề \(x^3y-2x^2y+xy\)
\(=y\left(x^3-2x^2+x\right)\)
\(=x\cdot y\cdot\left(x^2-2x+1\right)\)
\(=xy\left(x-1\right)^2\)
b: Sửa đề: \(x^2-9-2xy+y^2\)
\(=\left(x^2-2xy+y^2\right)-9\)
\(=\left(x-y\right)^2-9\)
\(=\left(x-y-3\right)\left(x-y+3\right)\)
Bài 2:
a: ĐKXĐ: \(x\notin\left\{3;-3;-1\right\}\)
b: \(A=\left(\dfrac{x}{x+3}-\dfrac{2}{x-3}+\dfrac{x^2-1}{9-x^2}\right):\left(2-\dfrac{x+5}{x+3}\right)\)
\(=\left(\dfrac{x}{x+3}-\dfrac{2}{x-3}-\dfrac{x^2-1}{\left(x-3\right)\left(x+3\right)}\right):\dfrac{2x+6-x-5}{x+3}\)
\(=\dfrac{x\left(x-3\right)-2\left(x+3\right)-x^2+1}{\left(x+3\right)\left(x-3\right)}\cdot\dfrac{x+3}{x+1}\)
\(=\dfrac{x^2-3x-2x-6-x^2+1}{x-3}\cdot\dfrac{1}{x+1}\)
\(=\dfrac{-5x-5}{\left(x-3\right)\left(x+1\right)}=-\dfrac{5\left(x+1\right)}{\left(x-3\right)\left(x+1\right)}=-\dfrac{5}{x-3}\)
c: \(x^2-x-2=0\)
=>\(\left(x-2\right)\left(x+1\right)=0\)
=>\(\left[{}\begin{matrix}x-2=0\\x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\left(nhận\right)\\x=-1\left(loại\right)\end{matrix}\right.\)
Thay x=2 vào A, ta được:
\(A=\dfrac{-5}{2-3}=\dfrac{-5}{-1}=5\)
Đúng 3
Bình luận (1)
Tìm tập xác định của hàm số
y
x
+
2
1
2
A.
D
ℝ
B.
D
ℝ
−
2
C.
D
2
;
+
∞
D. ...
Đọc tiếp
Tìm tập xác định của hàm số y = x + 2 1 2
A. D = ℝ
B. D = ℝ \ − 2
C. D = 2 ; + ∞
D. D = − 2 ; + ∞
Đáp án D
Điều kiện x + 2 > 0 ⇔ x > − 2 ⇒ D = − 2 ; + ∞
Đúng 0
Bình luận (0)
ChoP=\(\left(\dfrac{3}{\sqrt{x}+1}-\dfrac{1}{x-1}\right):\dfrac{1}{\sqrt{x}+1}\)
a,Tìm tập xác định và rút gọn biểu thức P
b,Tìm để P=\(\dfrac{5}{4}\)
c,Tìm giá trị nhỏ nhất của M = \(\dfrac{x+12}{\sqrt{x}-1}\cdot\dfrac{1}{P}\)
a: TXĐ: D=[0;+\(\infty\))\{1}
Ta có: \(P=\left(\dfrac{3}{\sqrt{x}+1}-\dfrac{1}{x-1}\right):\dfrac{1}{\sqrt{x}+1}\)
\(=\dfrac{3\sqrt{x}-3-1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\cdot\dfrac{\sqrt{x}+1}{1}\)
\(=\dfrac{3\sqrt{x}-4}{\sqrt{x}-1}\)
Đúng 0
Bình luận (0)
Cho biểu thức P =(1/(x-cănx)+cănx/(x-1)):(xcănx-1)/(xcănx-cănx) (với x>0 và x1)
a)Rút gọn P.
b) Tìm x để P=1/2
a) \(P=\left(\dfrac{1}{x-\sqrt{x}}+\dfrac{\sqrt{x}}{x-1}\right):\left(\dfrac{x\sqrt{x}-1}{x\sqrt{x}-\sqrt{x}}\right)\)
\(P=\left(\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}+\dfrac{\sqrt{x}}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\right):\left(\dfrac{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right)\)
\(P=\left(\dfrac{\sqrt{x}+1+x}{\sqrt{x}\left(\sqrt{x}+1\right)\left(\sqrt{x}+1\right)}\right):\dfrac{x+\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}+1\right)}\)
\(P=\dfrac{x+\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\cdot\dfrac{\sqrt{x}\left(\sqrt{x}+1\right)}{x+\sqrt{x}+1}\)
\(P=\dfrac{1}{\sqrt{x}-1}\)
b) P = \(\dfrac{1}{2}\) khi:
\(\dfrac{1}{\sqrt{x}-1}=\dfrac{1}{2}\)
\(\Rightarrow2=\sqrt{x}-1\)
\(\Rightarrow\sqrt{x}=3\)
\(\Rightarrow x=9\left(tm\right)\)
Đúng 1
Bình luận (0)
a: \(P=\left(\dfrac{1}{x-\sqrt{x}}+\dfrac{\sqrt{x}}{x-1}\right):\dfrac{x\sqrt{x}-1}{x\sqrt{x}-\sqrt{x}}\)
\(=\dfrac{\sqrt{x}+1+x}{\sqrt{x}\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\cdot\dfrac{\sqrt{x}\left(x-1\right)}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\)
\(=\dfrac{1}{\sqrt{x}-1}\)
b: P=1/2
=>căn x-1=2
=>căn x=3
=>x=9
Đúng 0
Bình luận (0)
a) Để rút gọn biểu thức P, ta thực hiện các bước sau: P = [(1/(x-√x)) + (√x/(x-1))] : [(x√x-1)/(x√x-√x)] Đầu tiên, ta nhân tử và mẫu của phân số bên trái với (x-√x) để loại bỏ mẫu phân số trong dấu ngoặc: P = [(1/(x-√x)) * (x-√x) + (√x/(x-1)) * (x-√x)] : [(x√x-1)/(x√x-√x)] P = [1 + (√x * (x-√x))/(x-1)] : [(x√x-1)/(x√x-√x)] Tiếp theo, ta nhân tử và mẫu của phân số bên phải với (x√x+√x) để loại bỏ mẫu phân số trong dấu ngoặc: P = [1 + (√x * (x-√x))/(x-1)] * [(x√x+√x)/(x√x+√x)] : [(x√x-1)/(x√x-√x)] P = [(x√x+√x + √x * (x-√x))/(x-1)] * [(x√x+√x)/(x√x-1)] P = [(x√x+√x + √x * (x-√x)) * (x√x+√x)] / [(x-1) * (x√x-1)] P = [(x√x+√x + √x * (x-√x)) * (x√x+√x)] / [(x-1) * (x√x-1)] P = [(x^2 + 2√x + x - x) * (x√x+√x)] / [(x-1) * (x√x-1)] P = [(x^2 + 2√x) * (x√x+√x)] / [(x-1) * (x√x-1)] P = [(x^2 + 2√x) * (x√x+√x)] / [(x-1) * (x√x-1)] P = [(x^3 + 3x√x + 2x)] / [(x-1) * (x√x-1)] P = (x^3 + 3x√x + 2x) / (x^2√x - x√x - x + 1) Vậy biểu thức P sau khi rút gọn là (x^3 + 3x√x + 2x) / (x^2√x - x√x - x + 1). b) Để tìm x để P = 1/2, ta giải phương trình: (x^3 + 3x√x + 2x) / (x^2√x - x√x - x + 1) = 1/2 Nhân cả hai vế của phương trình với (x^2√x - x√x - x + 1) để loại bỏ mẫu phân số: 2(x^3 + 3x√x + 2x) = x^2√x - x√x - x + 1 2x^3 + 6x√x + 4x = x^2√x - x√x - x + 1 2x^3 + 6x√x + 4x - x^2√x + x√x + x - 1 = 0 2x^3 + 5x√x + 5x - x^2√x - 1 = 0 Đây là phương trình không thể giải bằng phép tính đơn giản. Ta có thể sử dụng phương pháp số học hoặc phương pháp đồ thị để tìm nghiệm của phương trình này.
Đúng 1
Bình luận (0)