Tìm điều kiện xác định của 5 - 3 x
A. x ≤ 5 3
B. x ≥ 5 3
C. x ≥ 3 5
D. x ≤ 3 5
Tìm điều kiện của x để biểu thức xác định
a) \(\sqrt{-2x^2+3}\)
b) \(\sqrt{6x^2-6}\)
c) \(\sqrt{\dfrac{3}{-x^2+5}}\)
d) \(\sqrt{-x^3-5}\)
a: ĐKXĐ: \(-\dfrac{\sqrt{6}}{2}\le x\le\dfrac{\sqrt{6}}{2}\)
b: ĐKXĐ: \(\left[{}\begin{matrix}x\ge1\\x\le-1\end{matrix}\right.\)
c: ĐKXĐ: \(-\sqrt{5}< x< \sqrt{5}\)
d: ĐKXĐ: \(x\le\sqrt[3]{-5}\)
Cho biểu thức
2
2 5 1
25 5 5
x
A
x x x
= − −
− − +
1) Tìm điều kiện xác định của biểu thức A.
2) Rút gọn biểu thức A.
3) Tính giá trị của biểu thức A khi
x =1.
đkxđ:\(x\ne5,x\ne-5\)
\(\dfrac{2x}{\left(x-5\right)\left(x+5\right)}-\dfrac{5}{x-5}-\dfrac{1}{x+5}\)
\(\dfrac{2x}{\left(x-5\right)\left(x+5\right)}-\dfrac{5x+25}{\left(x-5\right)\left(x+5\right)}-\dfrac{x-5}{\left(x-5\right)\left(x+5\right)}\)
\(\dfrac{2x-5x-25-x+5}{\left(x-5\right)\left(x+5\right)}=\dfrac{-4x-20}{\left(x-5\right)\left(x+5\right)}=\dfrac{-4\left(x+5\right)}{\left(x-5\right)\left(x+5\right)}=-\dfrac{4}{x-5}\)
thay x=1 vào bt A, ta được:
\(-\dfrac{4}{1-5}=1\)
\frac{3}{x-3}-\frac{6x}{9-x^2}+\frac{x}{x+3}
tìm điều kiện xác định
rút gọn
tính x=5
ĐK: `x \ne 3; x \ne -3`
`A=3/(x-3)-(6x)/(9-x^2)+x/(x+3)`
`=3/(x-3)+(6x)/(x^2-9)+x/(x+3)`
`=3/(x-3)+(6x)/((x-3)(x+3))+x/(x+3)`
`=(3(x+3)+6x+x(x-3))/((x-3)(x+3))`
`=(3x+9+6x+x^2-3x)/((x+3)(x-3))`
`=(x^2+6x+9)/((x-3)(x+3))`
`=((x+3)^2)/((x-3)(x+3))`
`=(x+3)/(x-3)`
`x=5 => A=(5+3)/(5-3)=4`
ĐKXĐ:\(\left\{{}\begin{matrix}x-3\ne0\\9-x^2\ne0\\x+3\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne3\\x^2\ne9\\x\ne-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne3\\x\ne-3\end{matrix}\right.\)
\(\dfrac{3}{x-3}-\dfrac{6x}{9-x^2}+\dfrac{x}{x+3}\\ =\dfrac{3\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}-\dfrac{6x}{\left(3-x\right)\left(3+x\right)}+\dfrac{x\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}\\ =\dfrac{3x+9}{\left(x-3\right)\left(x+3\right)}+\dfrac{6x}{\left(x-3\right)\left(x+3\right)}+\dfrac{x^2-3x}{\left(x-3\right)\left(x+3\right)}\\ =\dfrac{3x+9+6x+x^2-3x}{\left(x-3\right)\left(x+3\right)}\\ =\dfrac{x^2+6x+9}{\left(x-3\right)\left(x+3\right)}\\ =\dfrac{\left(x+3\right)^2}{\left(x-3\right)\left(x+3\right)}\\ =\dfrac{x+3}{x-3}\)
Thay x=5 vào \(\dfrac{x+3}{x-3}=\dfrac{5+3}{5-3}=\dfrac{8}{2}=4\)
Cho biết : M = 4/x+2+3/x-2-5x+2/x^2-4 a) tìm điều kiện xác định của M b) rút gọn M c) tìm x để M = 2/5
\(M=\dfrac{4}{x+2}+\dfrac{3}{x-2}-\dfrac{5x+2}{x^2-4}\left(dkxd:x\ne\pm2\right)\)
\(=\dfrac{4}{x+2}+\dfrac{3}{x-2}-\dfrac{5x+2}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{4\left(x-2\right)+3\left(x+2\right)-\left(5x+2\right)}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{4x-8+3x+6-5x-2}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{2x-4}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{2\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{2}{x+2}\)
Để \(M=\dfrac{2}{5}\) thì \(\dfrac{2}{x+2}=\dfrac{2}{5}\)
Suy ra :
\(2.5=2\left(x+2\right)\)
\(\Leftrightarrow2x+4=10\)
\(\Leftrightarrow x=3\)
Vậy \(M=\dfrac{2}{5}\) thì x = 3
Tìm điều kiện xác định của biểu thức : B = \(\sqrt{x^2-3x}\) + \(\sqrt{\dfrac{x-5}{x-1}}\)- \(\sqrt[3]{2x-1}\)
Tìm điều kiện của x để phân thức sau xác định:
1) \(\dfrac{5-x}{x^2-3x}\)
2) \(\dfrac{3x}{2x+3}\)
1) \(\dfrac{5-x}{x^2-3x}=\dfrac{5-x}{x\left(x-3\right)}\left(đk:x\ne0,x\ne3\right)\)
2) \(\dfrac{3x}{2x+3}\left(đk:x\ne-\dfrac{3}{2}\right)\)
cho biểu thức B=[ x+1/ 2x-2 +3/x2 -1 -x+3/2x+2] .2x2 -2/5
a, tìm điều kiện của biến để phân thức xác định
b, c/m rằng khi giá trị của biểu thức xác định thì nó không phụ thuộc vào biến x ?
bài 1: tìm điều kiện xác định với giá trị nào của x thì các biểu thức sau đây xác định
a, \(\sqrt{-2x+3}\)
b, \(\sqrt{3x+4}\)
c, \(\sqrt{1+x\overset{2}{ }}\)
d, \(\sqrt{^{-3}_{3x+5}}\)
e, \(\sqrt{\dfrac{2}{x}}\)
help me :((
a/ ĐKXĐ : \(-2x+3\ge0\)
\(\Leftrightarrow x\le\dfrac{3}{2}\)
b/ ĐKXĐ : \(3x+4\ge0\)
\(\Leftrightarrow x\ge-\dfrac{4}{3}\)
c/ Căn thức \(\sqrt{1+x^2}\) luôn được xác định với mọi x
d/ ĐKXĐ : \(-\dfrac{3}{3x+5}\ge0\)
\(\Leftrightarrow3x+5< 0\)
\(\Leftrightarrow x< -\dfrac{5}{3}\)
e/ ĐKXĐ : \(\dfrac{2}{x}\ge0\Leftrightarrow x>0\)
P.s : không chắc lắm á!
bài 1: Tìm điều kiện xác định của phân thức:
a) x2-4/x2-16
b)5/(x-1)(x2+3)
a: ĐKXĐ: \(x\notin\left\{4;-4\right\}\)
b: ĐKXĐ: \(x\ne1\)
Bài 1: Ph tích thành nhân tử
b) x^5-x^3-x^2+1
Bài 2: Cho biểu thức 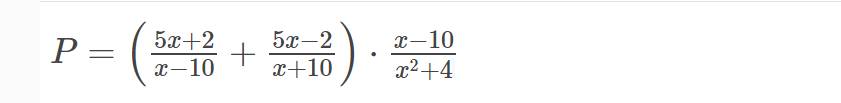
a) tìm điều kiện xác định của P
b) Rút gọn biểu thức P
c) tính giá trị của P khi x=2/5
Bài 2
a) ĐKXĐ: x - 10 0 và x + 10 0
*) x - 10 0
x 10
*) x + 10 0
x 10
Vậy ĐKXĐ: x -10; x 10
b) P = [(5x + 2)(x + 10) + (5x - 2)(x - 10)]/[(x - 10)(x + 10)] . (x - 10)/(x² + 4)
= (5x² + 50x + 2x + 20 + 5x² - 50x - 2x + 20)/[(x + 10)(x² + 4)]
= (10x² + 40)/[(x + 10)(x² + 4)]
= 10(x² + 4)/[(x + 10)(x² + 4)]
= 10/(x + 10)
c) Khi x = 2/5 ta có:
P = 10.(2/5 + 10)
= 4 + 100
= 104
Bài 1
b) x⁵ - x³ - x² + 1
= (x⁵ - x³) - (x² - 1)
= x³.(x² - 1) - (x² - 1)
= (x² - 1)(x³ - 1)
= (x - 1)(x + 1)(x - 1)(x² + x + 1)
= (x + 1)(x - 1)²(x² + x + 1)