sin (2x + η/3 )= 5
Sin (2x+ η/10)= -2
Sin (x- η/6)= √3/2
Sin ( x-η/4)= √2/2
Giải phương trình lượng giác sau
1) 2 cos 2x -\(\sqrt{3}\) = 0
2)\(\sqrt{3}\) tan x + 1 = 0
3) 2 cos2x = 1
4) 6 sin2 x- 13 sin x + 5 = 0
5) 5 cos 2x + 6 cos x + 1 = 0
6 ) 2 cos 2 2x - 3 cos 2x + 1 = 0
7) tan 2 x + ( 1 - \(\sqrt{3}\)) tan x - \(\sqrt{3}\) = 0
8) cos 6x + 2 sin 3x + 3 = 0
9) cos 2x - 4 cos x - 5 = 0
10 ) 3 cos 2 x = 2 sin 2 x + 4 sin x
11) cos 2x + sin2x + 2 cos x + 1 = 0
12) cos 4x + sin 4x + sin 2x = \(\dfrac{5}{2}\)
Giải các phương trình sau :
a) \(2\sin^2x+\sin x\cos x-3\cos^2x=0\)
b) \(3\sin^2-4\sin x\cos x+5\cos^2x=2\)
c) \(\sin^2x+\sin2x-2\cos^2+5\cos^2x=2\)
d) \(2\cos^2x-3\sqrt{3}\sin2x-4\sin^2x=-4\)
a) Dễ thấy cosx = 0 không thỏa mãn phương trình đã cho nên chiaw phương trình cho cos2x ta được phương trình tương đương 2tan2x + tanx - 3 = 0.
Đặt t = tanx thì phương trình này trở thành
2t2 + t - 3 = 0 ⇔ t ∈ {1 ; ![]() }.
}.
Vậy ![]()
b) Thay 2 = 2(sin2x + cos2x), phương trình đã cho trở thành
3sin2x - 4sinxcosx + 5cos2x = 2sin2x + 2cos2x
⇔ sin2x - 4sinxcosx + 3cos2x = 0
⇔ tan2x - 4tanx + 3 = 0
⇔ ![]()
⇔ x = ![]() + kπ ; x = arctan3 + kπ, k ∈ Z.
+ kπ ; x = arctan3 + kπ, k ∈ Z.
c) Thay sin2x = 2sinxcosx ; ![]() =
= ![]() (sin2x + cos2x) vào phương trình đã cho và rút gọn ta được phương trình tương đương
(sin2x + cos2x) vào phương trình đã cho và rút gọn ta được phương trình tương đương
![]() sin2x + 2sinxcosx -
sin2x + 2sinxcosx - ![]() cos2x = 0 ⇔ tan2x + 4tanx - 5 = 0 ⇔
cos2x = 0 ⇔ tan2x + 4tanx - 5 = 0 ⇔ ![]()
⇔ x = ![]() + kπ ; x = arctan(-5) + kπ, k ∈ Z.
+ kπ ; x = arctan(-5) + kπ, k ∈ Z.
d) 2cos2x - 3√3sin2x - 4sin2x = -4
⇔ 2cos2x - 3√3sin2x + 4 - 4sin2x = 0
⇔ 6cos2x - 6√3sinxcosx = 0 ⇔ cosx(cosx - √3sinx) = 0
⇔ 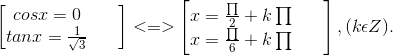
1,phương trình 3sin(2x-η/5)+1=m có nghiệm khi m∈(a,b).Giá trị b-a là
\(3sin\left(2x-\dfrac{\pi}{5}\right)+1=m\)
\(\Leftrightarrow sin\left(2x-\dfrac{\pi}{5}\right)=\dfrac{m-1}{3}\)
Phương trình có nghiệm khi:
\(-1\le\dfrac{m-1}{3}\le1\)
\(\Leftrightarrow-2\le m\le4\)
\(\Rightarrow m\in\left[-2;4\right]\)
\(\Rightarrow b-a=6\)
Giải các phương trình sau:
1) sin2x + sin23x - 3cos22x = 0
2) sin22x + sin24x = sin26x
3) cos4x - 5sin4x = 1
4) sin24x + sin23x = cos22x +cos2x với x∈(0;π)
5) 4sin3x - 1 = 3 - √3cos3x
6)sin2x = cos22x + cos23x
Giải các phương trình sau:
\(5\sin^22x-6\sin4x-2\cos^2x=0\)
\(2\sin^23x-10\sin6x-\cos^23x=-2\)
\(\sin^2x\left(\tan x+1\right)=3\sin x\left(\cos x-\sin x\right)+3\)
\(6\sin x-2\cos^3x=\frac{5\sin4x.\cos x}{2\cos2x}\)
Giải phương trình sau:
a) $\tan ^2x+4\cos ^2x+7=4\tan x+8\cot x$
b) $6\sin ^2x+2\cos ^2x-2\sqrt{3}\sin 2x=14\sin \left(x-\frac{\pi }{6}\right)$
\(\sin^22x-\sin^2x=\frac{1}{2}\)
\(2\sin^2x+4\sin x=3\cos^2x\)
\(\sin^3x+3\sin^2x+2\sin x=0\)
a.
\(1-cos^22x-\left(\frac{1-cos2x}{2}\right)=\frac{1}{2}\)
\(\Leftrightarrow2cos^22x-cos2x=0\)
\(\Leftrightarrow cos2x\left(2cos2x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=0\\cos2x=\frac{1}{2}\\\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2x=\frac{\pi}{2}+k\pi\\2x=\frac{\pi}{3}+k2\pi\\2x=-\frac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{4}+\frac{k\pi}{2}\\x=\frac{\pi}{6}+k\pi\\x=-\frac{\pi}{6}+k\pi\end{matrix}\right.\)
b.
\(\Leftrightarrow2sin^2x+4sinx=3\left(1-sin^2x\right)\)
\(\Leftrightarrow5sin^2x+4sinx-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=\frac{-2-\sqrt{19}}{5}\left(l\right)\\sinx=\frac{-2+\sqrt{19}}{5}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=arcsin\left(\frac{-2+\sqrt{19}}{5}\right)+k2\pi\\x=\pi-arcsin\left(\frac{-2+\sqrt{19}}{5}\right)+k2\pi\end{matrix}\right.\)
c.
\(\Leftrightarrow sinx\left(sin^2x+3sinx+2\right)=0\)
\(\Leftrightarrow sinx\left(sinx+1\right)\left(sinx+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=0\\sinx=-1\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k\pi\\x=-\frac{\pi}{2}+k2\pi\end{matrix}\right.\)
Tìm GTLN, GTNN:
a, \(y=4\sin^2x-4\sin x+3\).
b, \(y=\cos^2x+2\sin x+2\).
c, \(y=\sin^4x-2\cos^2x+1\).
a.
Tìm min:
$y=(4\sin ^2x-4\sin x+1)+2=(2\sin x-1)^2+2$
Vì $(2\sin x-1)^2\geq 0$ với mọi $x$ nên $y=(2\sin x-1)^2+2\geq 0+2=2$
Vậy $y_{\min}=2$
----------------
Mặt khác:
$y=4\sin x(\sin x+1)-8(\sin x+1)+11$
$=(\sin x+1)(4\sin x-8)+11$
$=4(\sin x+1)(\sin x-2)+11$
Vì $\sin x\in [-1;1]\Rightarrow \sin x+1\geq 0; \sin x-2<0$
$\Rightarrow 4(\sin x+1)(\sin x-2)\leq 0$
$\Rightarrow y=4(\sin x+1)(\sin x-2)+11\leq 11$
Vậy $y_{\max}=11$
b.
$y=\cos ^2x+2\sin x+2=1-\sin ^2x+2\sin x+2$
$=3-\sin ^2x+2\sin x$
$=4-(\sin ^2x-2\sin x+1)=4-(\sin x-1)^2\leq 4-0=4$
Vậy $y_{\max}=4$.
---------------------------
Mặt khác:
$y=3-\sin ^2x+2\sin x = (1-\sin ^2x)+(2+2\sin x)$
$=(1-\sin x)(1+\sin x)+2(1+\sin x)=(1+\sin x)(1-\sin x+2)$
$=(1+\sin x)(3-\sin x)$
Vì $\sin x\in [-1;1]$ nên $1+\sin x\geq 0; 3-\sin x>0$
$\Rightarrow y=(1+\sin x)(3-\sin x)\geq 0$
Vậy $y_{\min}=0$
c.
$y=\sin ^4x-2\cos ^2x+1=\sin ^4x-2(1-\sin ^2x)+1$
$=\sin ^4x+2\sin ^2x-1$
$=(\sin ^4x-1)+(2\sin ^2x-2)+2$
$=(\sin ^2x-1)(\sin ^2x+1)+2(\sin ^2x-1)+2$
$=(\sin ^2x-1)(\sin ^2x+3)+2$
Vì $\sin x\in [-1;1]$ nên $\sin ^2x\leq 1$
$\Rightarrow (\sin ^2x-1)(\sin ^2x+3)\leq 0$
$\Rightarrow y=(\sin ^2x-1)(\sin ^2x+3)+2\leq 2$
Vậy $y_{\max}=2$
------------------------------------------
$y=\sin ^4x+2\sin ^2x-1=\sin ^2x(\sin ^2x+2)-1$
Vì $\sin ^2x\geq 0$ nên $\sin ^2x(\sin ^2x+2)\geq 0$
$\Rightarrow y=\sin ^2x(\sin ^2x+2)-1\geq 0-1=-1$
Vậy $y_{\min}=-1$
Giải các phương trình sau :
a) \(\cos^2x+2\sin x\cos x+5\sin^2x=2\)
b) \(3\cos^2x-2\sin2x+\sin^2x=1\)
c) \(4\cos^2x-3\sin x\cos x+3\sin^2x=1\)