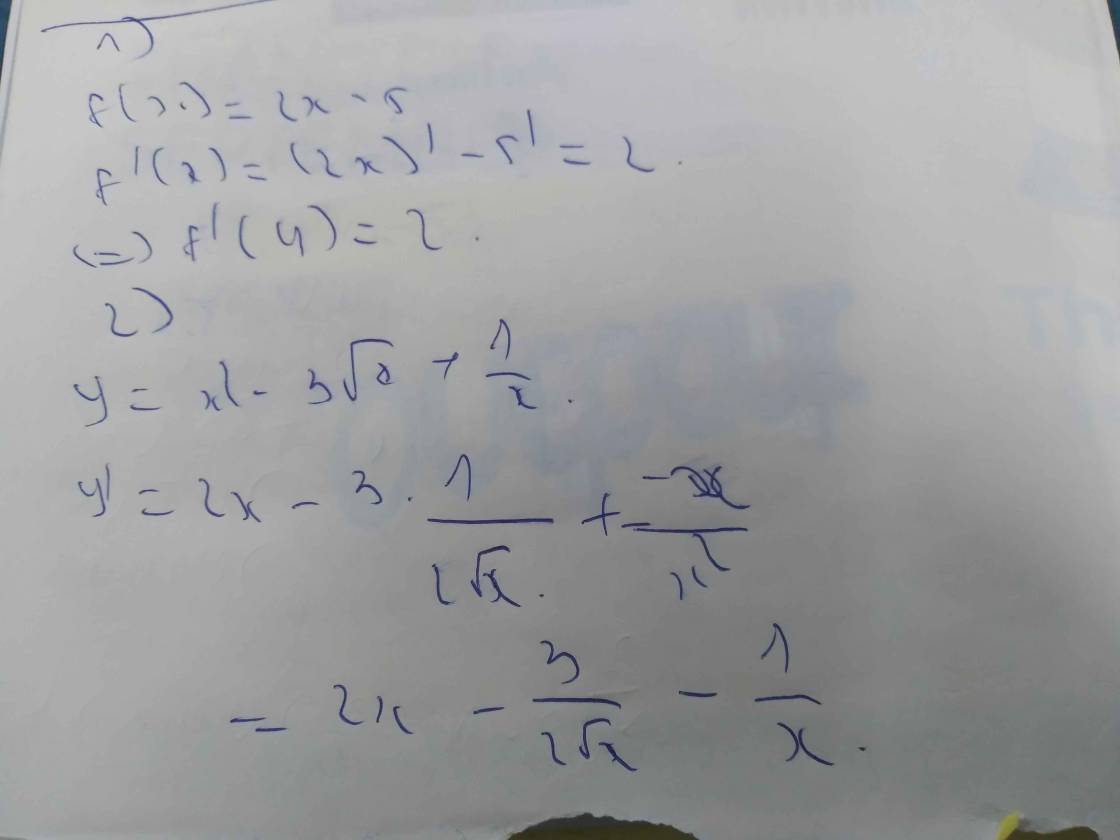Tính đạo hàm của hàm số \(\sqrt{x-1}+\frac{1}{\sqrt{x-1}}\)
Những câu hỏi liên quan
SGK Kết nối tri thức và cuộc sống
17 tháng 8 2023 lúc 10:36
Tính đạo hàm của các hàm số sau:
a) \(y = \frac{{\sqrt x }}{{x + 1}};\)
b) \(y = \left( {\sqrt x + 1} \right)\left( {{x^2} + 2} \right).\)
\(a,y'=\left(\dfrac{\sqrt{x}}{x+1}\right)'\\ =\dfrac{\left(\sqrt{x}\right)'\left(x+1\right)-\sqrt{x}\left(x+1\right)}{\left(x+1\right)^2}\\ =\dfrac{\dfrac{x+1}{2\sqrt{x}}-\sqrt{x}}{\left(x+1\right)^2}\\ =\dfrac{x+1-2x}{2\sqrt{x}\left(x+1\right)^2}\\ =\dfrac{-x+1}{2\sqrt{x}\left(x+1\right)^2}\)
\(b,y'=\left(\sqrt{x}+1\right)'\left(x^2+2\right)+\left(\sqrt{x}+1\right)\left(x^2+2\right)'\\ =\dfrac{x^2+2}{2\sqrt{x}}+\left(\sqrt{x}+1\right)\cdot2x\)
Đúng 1
Bình luận (0)
1. đạo hàm của hàm số f(x) = 2x - 5 tại \(x_0=4\)
2. đạo hàm của hàm số \(y=x^2-3\sqrt{x}+\dfrac{1}{x}\)
3. đạo hàm của hàm số \(f\left(x\right)=\dfrac{x+9}{x+3}+4\sqrt{x}\) tại điểm x = 1
1) \(f\left(x\right)=2x-5\)
\(f'\left(x\right)=2\)
\(\Rightarrow f'\left(4\right)=2\)
2) \(y=x^2-3\sqrt[]{x}+\dfrac{1}{x}\)
\(\Rightarrow y'=2x-\dfrac{3}{2\sqrt[]{x}}-\dfrac{1}{x^2}\)
3) \(f\left(x\right)=\dfrac{x+9}{x+3}+4\sqrt[]{x}\)
\(\Rightarrow f'\left(x\right)=\dfrac{1.\left(x+3\right)-1.\left(x+9\right)}{\left(x-3\right)^2}+\dfrac{4}{2\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=\dfrac{x+3-x-9}{\left(x-3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=\dfrac{12}{\left(x-3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=2\left[\dfrac{6}{\left(x-3\right)^2}+\dfrac{1}{\sqrt[]{x}}\right]\)
\(\Rightarrow f'\left(1\right)=2\left[\dfrac{6}{\left(1-3\right)^2}+\dfrac{1}{\sqrt[]{1}}\right]=2\left(\dfrac{3}{2}+1\right)=2.\dfrac{5}{2}=5\)
Đúng 3
Bình luận (0)
1) đạo hàm của hàm số \(y=x^2-3\sqrt{x}+\dfrac{1}{x}\)
2) đạo hàm của hàm số \(f\left(x\right)=\dfrac{x+9}{x+3}+4\sqrt{x}\) tại điểm x = 1
1) \(y=x^2-3\sqrt[]{x}+\dfrac{1}{x}\)
\(\Rightarrow y=2x-\dfrac{3}{2\sqrt[]{x}}-\dfrac{1}{x^2}\)
2) \(f\left(x\right)=\dfrac{x+9}{x+3}+4\sqrt[]{x}\)
\(\Rightarrow f'\left(x\right)=\dfrac{1.\left(x+3\right)-1\left(x+9\right)}{\left(x+3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=\dfrac{x+3-x-9}{\left(x+3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(x\right)=\dfrac{-6}{\left(x+3\right)^2}+\dfrac{2}{\sqrt[]{x}}\)
\(\Rightarrow f'\left(1\right)=\dfrac{-6}{\left(1+3\right)^2}+\dfrac{2}{\sqrt[]{1}}=-\dfrac{3}{8}+2=\dfrac{13}{8}\)
Đúng 1
Bình luận (0)
1. Đạo hàm của hàm số y= \(\left(x^3-5\right).\sqrt{x}\) bằng bao nhiêu?
2. Đạo hàm của hàm số y= \(\dfrac{1}{2}x^6-\dfrac{3}{x}+2\sqrt{x}\) là?
3. Hàm số y= \(2x+1+\dfrac{2}{x-2}\) có đạo hàm bằng?
1. \(y'=3x^2\sqrt{x}+\dfrac{x^3-5}{2\sqrt{x}}=\dfrac{7x^3-5}{2\sqrt{x}}\)
2. \(y'=3x^5+\dfrac{3}{x^2}+\dfrac{1}{\sqrt{x}}\)
3. \(y'=2-\dfrac{2}{\left(x-2\right)^2}\)
Đúng 1
Bình luận (0)
SGK Kết nối tri thức và cuộc sống
17 tháng 8 2023 lúc 21:13
Tính đạo hàm của các hàm số sau:
a) \(y = {\left( {\frac{{2x - 1}}{{x + 2}}} \right)^5}\)
b) \(y = \frac{{2x}}{{{x^2} + 1}}\);
c) \(y = {e^x}{\sin ^2}x\);
d) \(y = \log (x + \sqrt x )\).
tham khảo:
a)\(y'\left(x\right)=5\left(\dfrac{2x-1}{x+2}\right)^4.\dfrac{\left(x+2\right)\left(2\right)-\left(2x-1\right).1}{\left(x+2\right)^2}\)
\(=\dfrac{10\left(2x-1\right)\left(x+2\right)^3}{\left(x+2\right)^4}=\dfrac{20x-50}{\left(x+2\right)^4}\)
b)\(y'\left(x\right)=\dfrac{2\left(x^2+1\right)-2x\left(2x\right)}{\left(x^2+1\right)^2}\)\(=\dfrac{2\left(1-x^2\right)}{\left(x^2+1\right)^2}\)
c)\(y'\left(x\right)=e^x.2sinxcosx+e^xsin^2x.2cosx\)
\(=2e^xsinx\left(cosx+sinxcosx\right)\)
\(=2e^xsinxcos^2x\)
d)\(y'\left(x\right)=\dfrac{1}{x\sqrt{x}}.\left(+\dfrac{1}{2\sqrt{x}}\right)\)
\(=\dfrac{1}{\sqrt{x}\left(2\sqrt{x}+\sqrt{x}+2\right)}\)
\(=\dfrac{1}{\sqrt{x}\left(3\sqrt{x}+2\right)}\)
Đúng 1
Bình luận (0)
Cho hàm số \(y=\sqrt{x+\sqrt{x^2+1}}\). Tính đạo gàm của hàm số.
A. \(y'=\dfrac{x+\sqrt{x^2+1}}{2\sqrt{x^2+1}}\)
B. \(y'=\dfrac{\sqrt{x+\sqrt{x^2+1}}}{\sqrt{x^2+1}}\)
C. \(y'=\dfrac{\sqrt{x^2+1}}{2\sqrt{\sqrt{x+\sqrt{x^2+1}}}}\)
D. \(y'=\dfrac{\sqrt{x+\sqrt{x^2+1}}}{2\sqrt{x^2+1}}\)
\(y'=\dfrac{\left(x+\sqrt{x^2+1}\right)'}{2\sqrt{x+\sqrt{x^2+1}}}=\dfrac{1+\dfrac{x}{\sqrt{x^2+1}}}{2\sqrt{x+\sqrt{x^2+1}}}=\dfrac{x+\sqrt{x^2+1}}{2\sqrt{x^2+1}.\sqrt{x+\sqrt{x^2+1}}}\)
\(=\dfrac{\sqrt{x+\sqrt{x^2+1}}}{2\sqrt{x^2+1}}\)
Đúng 1
Bình luận (0)
27 tháng 4 2022 lúc 20:49
Tính đạo hàm của hàm số sau:
y=\(\dfrac{x^5}{5}+\sqrt{x}-\dfrac{1}{x}+\dfrac{1}{x^3}-2\)
\(y'=\dfrac{1}{5}\cdot5x^4+\dfrac{1}{2\sqrt{x}}+\dfrac{1}{x^2}+\dfrac{-\left(x^3\right)'}{x^6}\)
\(=x^4+\dfrac{1}{2\sqrt{x}}+\dfrac{1}{x^2}+\dfrac{-3x^2}{x^6}=x^4+\dfrac{1}{2\sqrt{x}}+\dfrac{1}{x^2}-\dfrac{3}{x^4}\)
Đúng 0
Bình luận (0)
Tính đạo hàm các hàm số:
1.y = ex sinx + x2 cosx
2.y = cot (\(\dfrac{1}{1+X^2}\) )
3.y = \(\dfrac{\sqrt{1+x^2}+\sqrt{1-x^2}}{\sqrt{1+x^2}-\sqrt{1-x^2}}\)
SGK Chân trời sáng tạo
20 tháng 8 2023 lúc 20:06
Tìm đạo hàm của các hàm số:
a) \(y = \sqrt[4]{x}\) tại \(x = 1\);
b) \(y = \frac{1}{x}\) tại \(x = - \frac{1}{4}\);
a) \(y' = {\left( {\sqrt[4]{x}} \right)^\prime } = {\left( {{x^{\frac{1}{4}}}} \right)^\prime } = \frac{1}{4}{x^{\frac{1}{4} - 1}} = \frac{1}{4}{x^{ - \frac{3}{4}}} = \frac{1}{{4\sqrt[4]{{{x^3}}}}}\)
\(y'\left( 1 \right) = \frac{1}{{4\sqrt[4]{{{1^3}}}}} = \frac{1}{4}\).
b) \(y' = {\left( {\frac{1}{x}} \right)^\prime } = - \frac{1}{{{x^2}}}\)
\(y'\left( { - \frac{1}{4}} \right) = - \frac{1}{{{{\left( { - \frac{1}{4}} \right)}^2}}} = - 16\).
Đúng 0
Bình luận (0)
Tính các đạo hàm của hàm số sau:
a) \(y=\sqrt{x}\left(x+3\right)\)
b) \(y=\sqrt{2x^2-6x-9}\)
c) \(y=\left(\sqrt{x^2+1}+x\right)^{10}\)