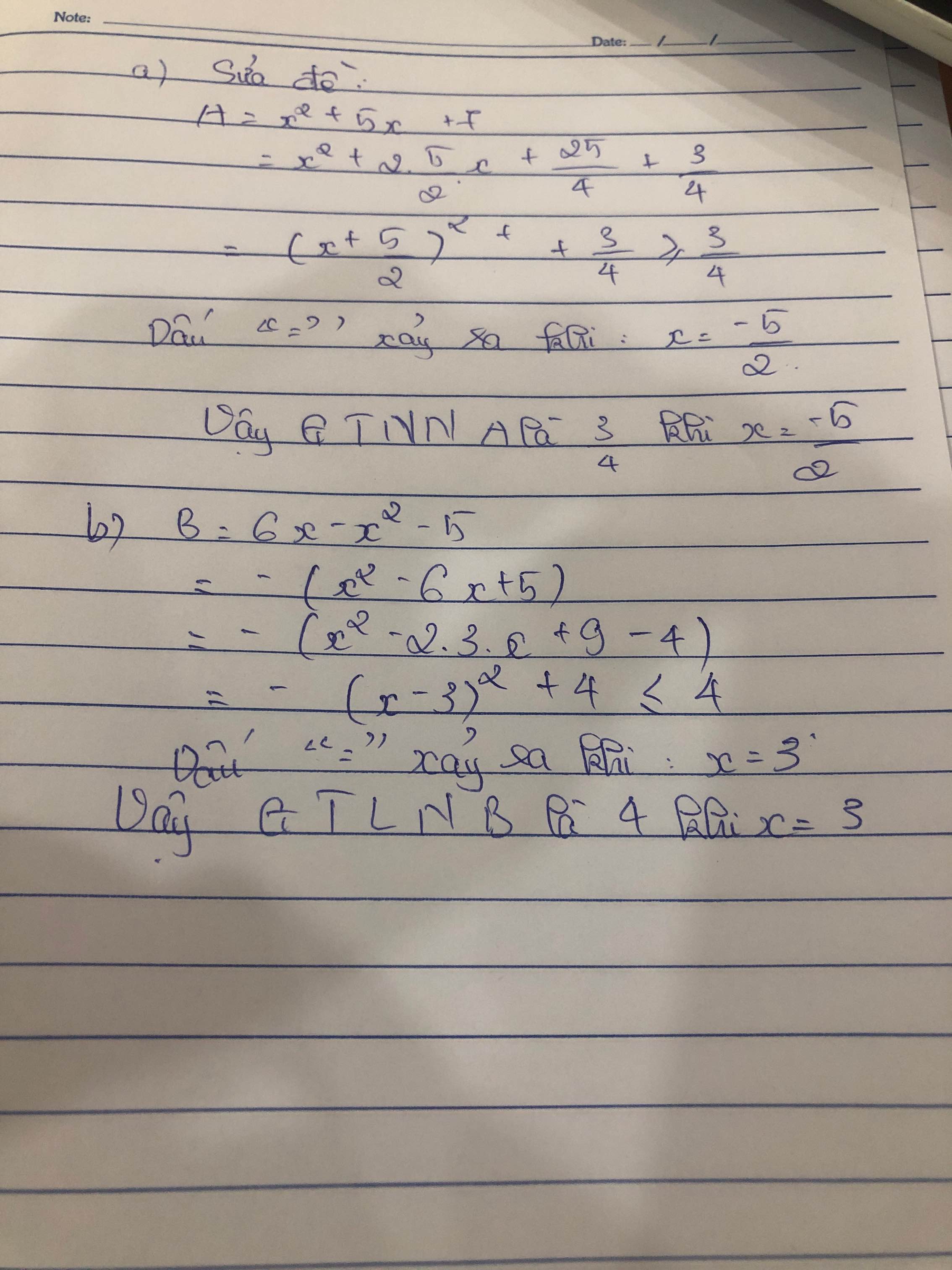`A=x^4-6x^3+18x^2-6xy+y^2+2012`
`=x^4-6x^3+9x^2+9x^2-6xy+y^2+2012`
`=(x^2-x)^2+(3x-y)^2+2012>=2012`
Dấu "=" xảy ra khi:
$\begin{cases}x=x^2\\y=3x\end{cases}$
`<=>` $\left[ \begin{array}{l}\begin{cases}x=0\\y=3x=0\\\end{cases}\\\begin{cases}x=1\\y=3x=3\\\end{cases}\end{array} \right.$
Vậy `min_A=2012<=>` $\left[ \begin{array}{l}x=y=0\\\begin{cases}x=1\\y=3\end{cases}\end{array} \right.$

mai nop cho co giao roi giup mk nha

ai giai dung mk h cho that nhieu

\(=\left(x^2+6x+9\right)-9+\left(y^2-y+\frac{1}{4}\right)-\frac{1}{4}+5\)

\(=\left(x+3\right)^2+\left(y-\frac{1}{2}\right)^2-\frac{17}{4}\)

Vì \(\left(x+3\right)^2\ge0\)

     \(\left(y-\frac{1}{2}\right)^2\ge0\)

nên \(A\ge-\frac{17}{4}\)

cau A thay = bằng cộng ạ

\(A=x^2+y^2+xy-6x-6y+2\)

\(\Rightarrow4A=4x^2+4y^2+4xy-24x-24y+8\)

           \(=\left(4x^2+4xy+y^2\right)+3y^2-24x-24y+8\)

            \(=\left[\left(2x+y\right)^2-12\left(2x+y\right)+36\right]+3y^2-12y-28\)

           \(=\left(2x+y-6\right)^2+3\left(y^2-4y+4\right)-40\)

            \(=\left(2x+y-6\right)^2+3\left(y-2\right)^2-40\ge-40\)

\(\Rightarrow4A\ge-40\)

\(\Rightarrow A\ge-10\)

Dấu "=" xảy ra \(\Leftrightarrow\hept{\begin{cases}2x+y-6=0\\y-2=0\end{cases}\Leftrightarrow\hept{\begin{cases}2x=6-y\\y=2\end{cases}\Leftrightarrow}\hept{\begin{cases}x=2\\y=2\end{cases}}}\)

Vậy \(A_{min}=-10\Leftrightarrow x=y=2\)

P/S: cách giải trên gọi là cách chung riêng !

\(A=\left(x^2+2\cdot\dfrac{3}{2}x+\dfrac{9}{4}\right)-\dfrac{5}{4}=\left(x+\dfrac{3}{2}\right)^2-\dfrac{5}{4}\ge-\dfrac{5}{4}\\ A_{min}=-\dfrac{5}{4}\Leftrightarrow x=-\dfrac{3}{2}\\ B=\left(x^2+2xy+y^2\right)+\left(x^2+6x+9\right)+3\\ B=\left(x+y\right)^2+\left(x+3\right)^2+3\ge3\\ B_{min}=3\Leftrightarrow\left\{{}\begin{matrix}x+y=0\\x+3=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\y=-3\end{matrix}\right.\\ C=-\left(x^2-2x+1\right)+1=-\left(x-1\right)^2+1\le1\\ C_{max}=1\Leftrightarrow x=1\)

a, \(A=-x^2-2x+3=-\left(x^2+2x-3\right)=-\left(x^2+2x+1-4\right)\)

\(=-\left(x+1\right)^2+4\le4\)

Dấu ''='' xảy ra khi x = -1 

Vậy GTLN là 4 khi x = -1 

b, \(B=-4x^2+4x-3=-\left(4x^2-4x+3\right)=-\left(4x^2-4x+1+2\right)\)

\(=-\left(2x-1\right)^2-2\le-2\)

Dấu ''='' xảy ra khi x = 1/2 

Vậy GTLN B là -2 khi x = 1/2 

c, \(C=-x^2+6x-15=-\left(x^2-2x+15\right)=-\left(x^2-2x+1+14\right)\)

\(=-\left(x-1\right)^2-14\le-14\)

Vâỵ GTLN C là -14 khi x = 1

Bài 8 : 

b, \(B=x^2-6x+11=x^2-6x+9+2=\left(x-3\right)^2+2\ge2\)

Dấu ''='' xảy ra khi x = 3

Vậy GTNN B là 2 khi x = 3 

c, \(x^2-x+1=x^2-x+\dfrac{1}{4}+\dfrac{3}{4}=\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4}\)

Dấu ''='' xảy ra khi x = 1/2 

Vậy ...

c, \(x^2-12x+2=x^2-12x+36-34=\left(x-6\right)^2-34\ge-34\)

Dấu ''='' xảy ra khi x = 6

Vậy ...

có làm mới có ăn nha em

Lời giải:

$A=(x^2+4y^2+4xy)+y^2+6x+16y+32$

$=(x+2y)^2+6(x+2y)+(y^2+4y)+32$

$=(x+2y)^2+6(x+2y)+9+(y^2+4y+4)+19$

$=(x+2y+3)^2+(y+2)^2+19\geq 0+0+19=19$

Vậy $A_{\min}=19$. Giá trị này đạt tại $x+2y+3=y+2=0$

$\Leftrightarrow y=-2; x=1$

`A=x^2+6x+y^2+4y+15`

`=(x^2+6x+9)+(y^2+4y+4)+2`

`=(x+3)^2+(y+2)^2+2`

Vì `(x+3)^2+(y+2)^2 >=0 forall x,y`

`=>A_(min)=2 <=> x=-3; y=-2`.

Ta có: \(A=x^2+6x+y^2+4y+15\)

\(=x^2+6x+9+y^2+4y+4+2\)

\(=\left(x+3\right)^2+\left(y+2\right)^2+2\ge2\forall x,y\)

Dấu '=' xảy ra khi (x,y)=(-3;-2)