tìm a,b sao cho A chia hết cho B
A=x4+ax3+bx -1
B=x2-1
Tìm a,b để đa thức : x4+ax3+bx-1 chia hết cho x2-1
MN làm giúp mình nha càng nhanh thì càng xing gái đẹp trai :3333
\(x^4+ax^3+bx-1=\left(x^2-1\right)\left(x^2+1\right)+ax\left(x^2-1\right)+\left(a+b\right)x\)
\(\Rightarrow x^4+ax^3+bx-1\) chia hết cho \(x^2-1\) khi \(a+b=0\)
\(\Leftrightarrow b=-a\)
(Chỉ cần a; b là 2 số đối nhau là đủ, có vô số cặp a;b thỏa mãn đề bài, ví dụ (a;b)=(1;-1); (2;-2); (3;-3)... đều đúng)
Tìm nÎZ để giá trị của biểu thức n3 -2n2 + 3n + 3 chia hết cho giá trị của biểu thức n-1
b) Tìm a để đa thức x4 + 6x3 + 7x2 - 6x + a chia hết cho đa thức x2 + 3x - 1
\(a,n^3-2n^2+3n+3=n^3-n^2-n^2+n+2n-2+5\\ =\left(n-1\right)\left(n^2-n+2\right)+5\\ \Leftrightarrow n^3-2n^2+3n+3⋮\left(n-1\right)\\ \Leftrightarrow5⋮n-1\\ \Leftrightarrow n-1\in\left\{-5;-1;1;5\right\}\\ \Leftrightarrow n\in\left\{-4;0;2;6\right\}\)
\(b,\Leftrightarrow x^4+6x^3+7x^2-6x+a\\ =x^4+3x^3-x^2+3x^3+9x^2-3x-x^2-3x+1-1+a\\ =\left(x^2+3x-1\right)\left(x^2+3x-1\right)-1+a\\ =\left(x^2+3x-1\right)^2+a-1\)
Để \(x^4+6x^3+7x^2-6x+a⋮x^2+3x-1\)
\(\Leftrightarrow a-1=0\Leftrightarrow a=1\)
tìm a,b sao cho f(x)= ax3 +bx2 +10x - 4 chia hết cho đa thức g(x) = x2 +x - 2
BIết x4+x2+1-(x2+ax+1)(x2+bx+1). Tính T-a+b+ab
A.T=-1
B.1
C.5
D.6
tìm a và b để
a) x4-9x3+21x2+ax+b chia hết cho x2-x-2
b) x4-7x3+10x2+(a-1)x+b-a chia hết cho x2-6x+5
xác định hệ số a,b để:
a) 4x3+ax+b chia hết cho x-2 và x+1
b) x4+ax+b chia hết cho x2-x+1
Cảm ơn nhiều ví đã giúp em ạ
\(a,4x^3+ax+b⋮x-2\\ \Leftrightarrow4x^3+ax+b=\left(x-2\right)\cdot a\left(x\right)\)
Thay \(x=2\Leftrightarrow32+2a+b=0\Leftrightarrow2a+b=-32\left(1\right)\)
\(4x^3+ax+b⋮x+1\\ \Leftrightarrow4x^3+ax+b=\left(x+1\right)\cdot b\left(x\right)\)
Thay \(x=-1\Leftrightarrow-4-a+b=0\Leftrightarrow a-b=-4\left(2\right)\)
Từ \(\left(1\right)\left(2\right)\) ta có hệ \(\left\{{}\begin{matrix}2a+b=-32\\a-b=-4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3a=-36\\b=a+4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=-12\\b=-8\end{matrix}\right.\)
Tìm a sao cho biểu thức A chia hết cho B(tìm a sao cho A:B ∈ Z)
1)A=x3-3x2-ax+3;B=x-1
2)A=3x3-16x2+25x+a;B=x2-4x+3
3)A=x4-x3+6x2-x+a;B=x2-x+5
\(1,A⋮B\Leftrightarrow x^3-3x^2-ax+3=\left(x-1\right)\cdot a\left(x\right)\)
Thay \(x=1\)
\(\Leftrightarrow1-3-a+3=0\\ \Leftrightarrow a=1\)
\(2,A⋮B\Leftrightarrow3x^3-16x^2+25x+a=\left(x^2-4x+3\right)\cdot b\left(x\right)\\ \Leftrightarrow3x^3-16x^2+25x+a=\left(x-3\right)\left(x-1\right)\cdot b\left(x\right)\)
Thay \(x=1\)
\(\Leftrightarrow3-16+25+a=0\\ \Leftrightarrow a=-12\)
Thay \(x=3\)
\(\Leftrightarrow3\cdot27-16\cdot9+25\cdot3+a=0\\ \Leftrightarrow81-144+75+a=0\\ \Leftrightarrow12+a=0\Leftrightarrow a=-12\)
Vậy \(a=-12\)
a) Tìm số nguyên âm x để đa thức:
f(x)= -x4+2x2-3x+5 chia hết cho g(x)= x-1
b) Tìm giá trị nhỏ nhất của biểu thức:
B= x2+5y2-2xy+4x-4y-2020
\(a,f\left(x\right)⋮g\left(x\right)\\ \Leftrightarrow\dfrac{-x^4+2x^2-3x+5}{x-1}\in Z\\ \Leftrightarrow\dfrac{-x^4+x^3-x^3+x^2+x^2-x-2x+2+3}{x-1}\in Z\\ \Leftrightarrow\dfrac{-x^3\left(x-1\right)-x^2\left(x-1\right)+x\left(x-1\right)-2\left(x-1\right)+3}{x-1}\in Z\\ \Leftrightarrow-x^3-x^2+x-2+\dfrac{3}{x-1}\in Z\\ \Leftrightarrow3⋮x-1\\ \Leftrightarrow x-1\inƯ\left(3\right)=\left\{-3;-1;1;3\right\}\\ \Leftrightarrow x\in\left\{-2;0;2;4\right\}\\ Mà.x< 0\\ \Leftrightarrow x=-2\\ b,B=\left(x^2-2xy+y^2\right)+4\left(x-y\right)+4+4y^2-2024\\ B=\left(x-y\right)^2+4\left(x-y\right)+4+4y^2-2024\\ B=\left(x-y-2\right)^2+4y^2-2024\ge-2024\\ B_{min}=-2024\Leftrightarrow\left\{{}\begin{matrix}x=y+2\\y=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=0\end{matrix}\right.\)
tìm a b c sao cho đa thức x4 + ax2 + bx + c chia hết cho đa thuc ( x - 3 )3
(Câu trả lời của alibaba nguyễn đúng mà hài!!!)
Sơ đồ Horner hoạt động như sau:
| 1 | 0 | a | b | c | |
| 3 | 1 | 3 | a+9 | 3a+b+27 | 9a+3b+c+27 |
| 3 | 1 | 6 | a+27 | 6a+b+108 | 27a+6b+c+351 |
| 3 | ... | ... | ... | ... | ... |
Cho đa thức A = x4 + x3 – 2x – 2
a) Tìm đa thức B sao cho A + B = x3 + 3x + 1
b) Tìm đa thức C sao cho A – C = x5
c) Tìm đa thức D biết rằng D = (2x2 – 3) . A
d) Tìm đa thức P sao cho A = (x+1) . P
e) Có hay không một đa thức Q sao cho A = (x2 + 1) . Q?
a) Ta có:
B = (A + B) – A
= (x3 + 3x + 1) – (x4 + x3 – 2x – 2)
= x3 + 3x + 1 – x4 - x3 + 2x + 2
= – x4 + (x3 – x3) + (3x + 2x) + (1 + 2)
= – x4 + 5x + 3.
b) C = A - (A – C)
= x4 + x3 – 2x – 2 – x5
= – x5 + x4 + x3 – 2x – 2.
c) D = (2x2 – 3) . A
= (2x2 – 3) . (x4 + x3 – 2x – 2)
= 2x2 . (x4 + x3 – 2x – 2) + (-3) .(x4 + x3 – 2x – 2)
= 2x2 . x4 + 2x2 . x3 + 2x2 . (-2x) + 2x2 . (-2) + (-3). x4 + (-3) . x3 + (-3). (-2x) + (-3). (-2)
= 2x6 + 2x5 – 4x3 – 4x2 – 3x4 – 3x3 + 6x + 6
= 2x6 + 2x5 – 3x4 + (-4x3 – 3x3) – 4x2+ 6x + 6
= 2x6 + 2x5 – 3x4 – 7x3 – 4x2+ 6x + 6.
d) P = A : (x+1) = (x4 + x3 – 2x – 2) : (x + 1)
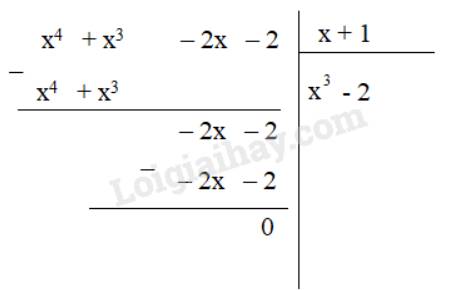
Vậy P = x3 - 2
e) Q = A : (x2 + 1)
Nếu A chia cho đa thức x2 + 1 không dư thì có một đa thức Q thỏa mãn
Ta thực hiện phép chia (x4 + x3 – 2x – 2) : (x2 + 1)
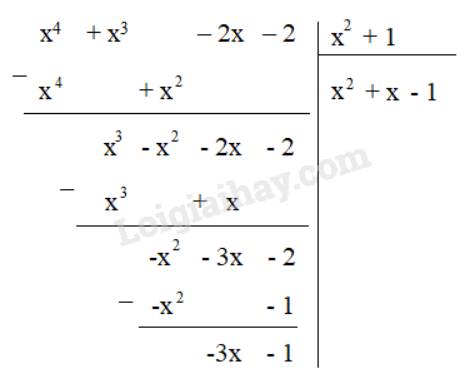
Do phép chia có dư nên không tồn tại đa thức Q thỏa mãn