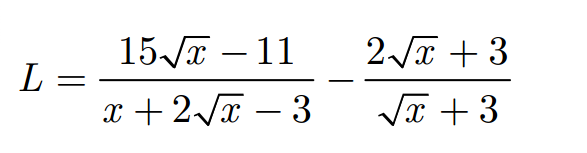\(\left(\dfrac{x\sqrt{x}+1}{x-\sqrt{x}}-\dfrac{x\sqrt{x}+1}{x+\sqrt{x}}+\dfrac{x+1}{\sqrt{x}}\right)\) điều kiện xát định :(x > 0 ; x \(\ne\) 1 )
= \(\left(\dfrac{x\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}-\dfrac{x\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}+1\right)}+\dfrac{x+1}{\sqrt{x}}\right)\)
= \(\dfrac{\left(x\sqrt{x}+1\right)\left(\sqrt{x}+1\right)-\left(x\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{\sqrt{x}\left(x-1\right)}+\dfrac{x+1}{\sqrt{x}}\)
= \(\dfrac{x^2+x\sqrt{x}+\sqrt{x}+1-\left(x^2-x\sqrt{x}+\sqrt{x}-1\right)}{\sqrt{x}\left(x-1\right)}+\dfrac{x+1}{\sqrt{x}}\)
= \(\dfrac{x^2+x\sqrt{x}+\sqrt{x}+1-x^2+x\sqrt{x}-\sqrt{x}+1}{\sqrt{x}\left(x-1\right)}+\dfrac{x+1}{\sqrt{x}}\)
= \(\dfrac{2x\sqrt{x}+2}{\sqrt{x}\left(x-1\right)}+\dfrac{x+1}{\sqrt{x}}\) = \(\dfrac{2x\sqrt{x}+2+\left(\left(x+1\right)\left(x-1\right)\right)}{\sqrt{x}\left(x-1\right)}\)
= \(\dfrac{2x\sqrt{x}+2+x^2-1}{\sqrt{x}\left(x-1\right)}\) = \(\dfrac{2x\sqrt{x}+x^2+1}{\sqrt{x}\left(x-1\right)}\)