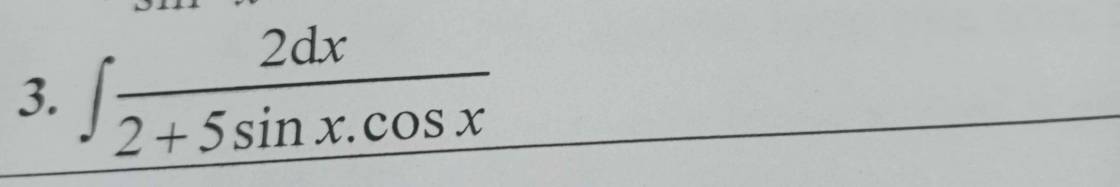a. \(\int cosx.sin^3xdx=\int sin^3x.d\left(sinx\right)=\dfrac{sin^4x}{4}+C\)
b. \(\int sinx.cos^5xdx=-\int cos^5x.d\left(cosx\right)=-\dfrac{cos^6x}{6}+C\)
c. \(\int x\left(x^2+1\right)^{10}dx=\dfrac{1}{2}\int\left(x^2+1\right)^{10}d\left(x^2+1\right)=\dfrac{\left(x^2+1\right)^{11}}{22}+C\)
d. \(\int x\left(2-x\right)^5dx\)
Đặt \(\left\{{}\begin{matrix}u=x\\dv=\left(2-x\right)^5dx\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}du=dx\\v=-\dfrac{1}{6}\left(2-x\right)^6\end{matrix}\right.\)
\(\Rightarrow I=-\dfrac{x\left(2-x\right)^6}{6}+\dfrac{1}{6}\int\limits\left(2-x\right)^6dx=-\dfrac{x\left(2-x\right)^6}{6}+\dfrac{1}{42}\left(x-2\right)^7+C\)
e.
\(I=\int\dfrac{2x+1}{\left(x-3\right)^3}dx=\int\dfrac{2\left(x-3\right)+6}{\left(x-3\right)^3}dx=2\int\dfrac{dx}{\left(x-3\right)^2}+6\int\dfrac{dx}{\left(x-3\right)^3}\)
\(=-\dfrac{2}{x-3}-\dfrac{3}{\left(x-3\right)^2}+C\)
f.
\(I=\int\dfrac{\left(2x-1\right)^4}{\left(x+3\right)^6}dx=\int\dfrac{\left[2\left(x+3\right)-7\right]^4}{\left(x+3\right)^6}dx=\int\left(2-\dfrac{7}{x+3}\right)^4.\dfrac{1}{\left(x+3\right)^2}dx\)
Đặt \(2-\dfrac{7}{x+3}=u\Rightarrow du=\dfrac{7}{\left(x+3\right)^2}dx\Rightarrow\dfrac{1}{\left(x+3\right)^2}dx=\dfrac{1}{7}du\)
\(\Rightarrow I=\dfrac{1}{7}\int u^4du==\dfrac{1}{35}.u^5+C=\dfrac{1}{35}\left(2-\dfrac{7}{x+3}\right)^5+C\)
g.
\(I=\int x\sqrt{1-x}dx\)
Đặt \(\sqrt{1-x}=u\Rightarrow x=1-u^2\Rightarrow dx=-2u.du\)
\(I=\int\left(1-u^2\right).u.\left(-2u.du\right)=\int\left(2u^4-2u^2\right)du=\dfrac{2}{5}u^5-\dfrac{2}{3}u^3+C\)
\(=\dfrac{2}{5}\sqrt{\left(1-x\right)^5}-\dfrac{2}{3}\sqrt{\left(1-x\right)^3}+C\)
h.
\(I=\int\dfrac{\left(1-2e^x\right)e^x}{e^x+1}dx\)
Đặt \(e^x+1=u\Rightarrow e^xdx=du\)
\(I=\int\dfrac{\left(1-2\left(u-1\right)\right)}{u}du=\int\dfrac{3-2u}{u}du=\int\left(\dfrac{3}{u}-2\right)du=3lnu-2u+C\)
\(=3ln\left(e^x+1\right)-2\left(e^x+1\right)+C=3ln\left(e^x+1\right)-2e^x+C\)
i.
Đặt \(lnx=u\Rightarrow\dfrac{dx}{x}=du\)
\(I=\int\left(u^2-3u\right)du=\dfrac{u^3}{3}-\dfrac{3u^2}{2}+C=\dfrac{ln^3x}{3}=\dfrac{3ln^2x}{2}+C\)

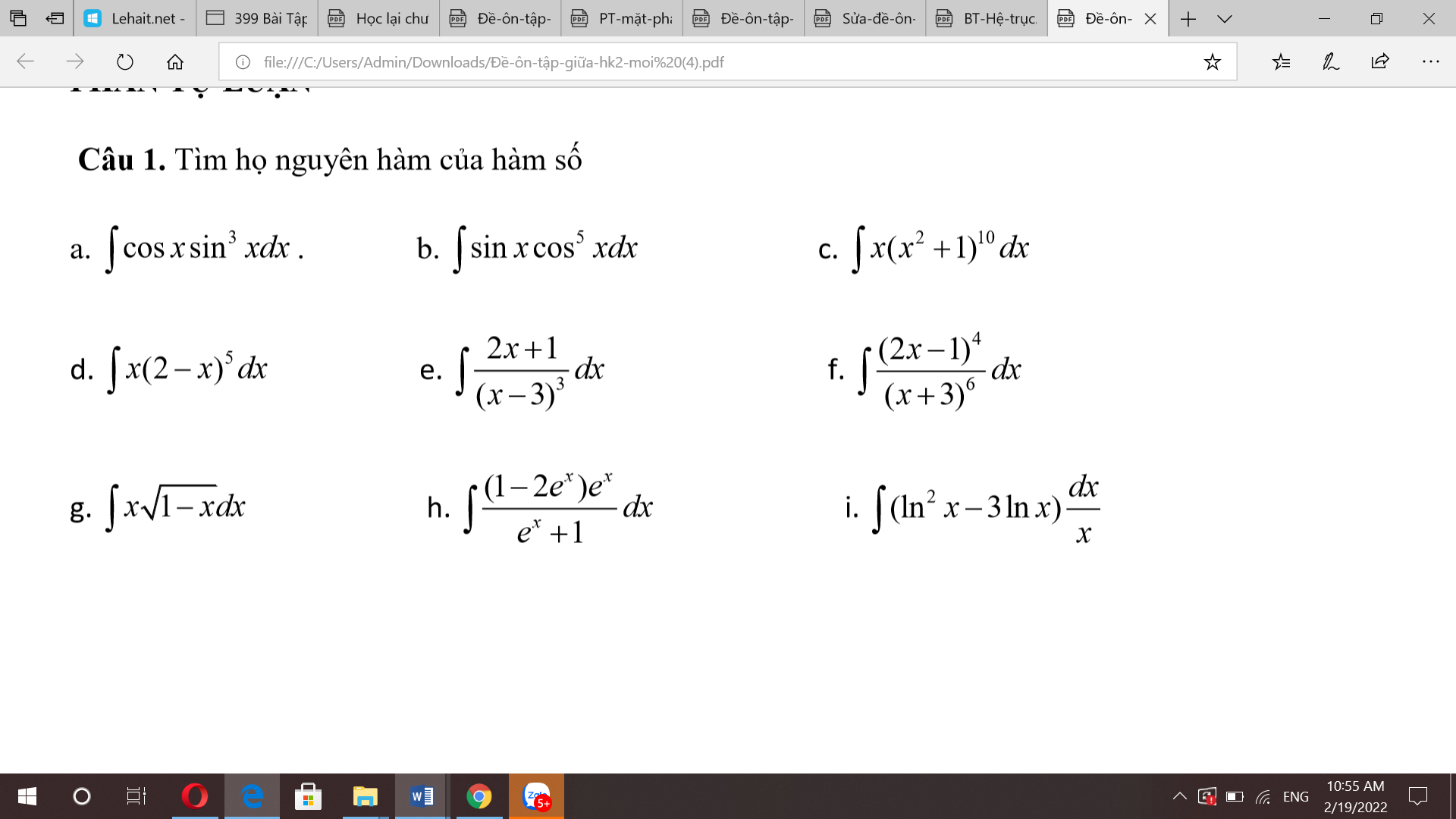








 Giúp em bài 3 với ạ
Giúp em bài 3 với ạ