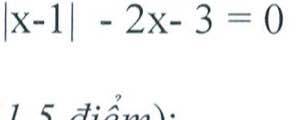1.\(ĐK:x\ne0;1\)
\(\dfrac{x+1}{x-1}-\dfrac{1}{x}=\dfrac{2}{x\left(x-1\right)}\)
\(\Leftrightarrow\dfrac{x\left(x+1\right)-\left(x-1\right)}{x\left(x-1\right)}=\dfrac{2}{x\left(x-1\right)}\)
\(\Leftrightarrow x\left(x+1\right)-\left(x-1\right)=2\)
\(\Leftrightarrow x^2+x-x+1-2=0\)
\(\Leftrightarrow x^2-1=0\)
\(\Leftrightarrow x^2=1\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\left(ktm\right)\\x=-1\left(tm\right)\end{matrix}\right.\)
2.\(ĐK:x\ne0;6\)
\(\dfrac{x+6}{x-6}-\dfrac{1}{x}=\dfrac{6}{x\left(x-6\right)}\)
\(\Leftrightarrow\dfrac{x\left(x+6\right)-\left(x-6\right)}{x\left(x-6\right)}=\dfrac{6}{x\left(x-6\right)}\)
\(\Leftrightarrow x\left(x+6\right)-\left(x-6\right)=6\)
\(\Leftrightarrow x^2+6x-x+6-6=0\)
\(\Leftrightarrow x^2+5x=0\)
\(\Leftrightarrow x\left(x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(ktm\right)\\x=-5\left(tm\right)\end{matrix}\right.\)
1: \(\Leftrightarrow x\left(x+1\right)-x+1=2\)
\(\Leftrightarrow x^2+x-x+1=2\)
\(\Leftrightarrow x^2=1\)
=>x=1(loại) hoặc x=-1(nhận)
2: \(\Leftrightarrow x\left(x+6\right)-x+6=6\)
\(\Leftrightarrow x^2+6x-x+6-6=0\)
=>x(x+5)=0
=>x=0(loại) hoặc x=-5(nhận)