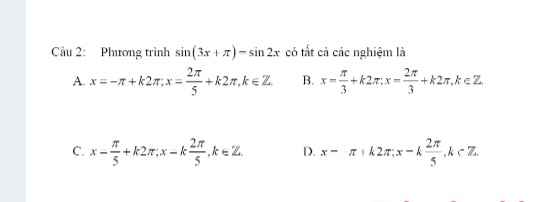\(4\sin^22x-4\cos2x-1=0\)
\(\Leftrightarrow4\left(1-\cos^22x\right)-4\cos2x-1=0\)
\(\Leftrightarrow4-4\cos^22x-4\cos2x-1=0\)
\(\Leftrightarrow-4\cos^22x-4\cos2x+3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cos2x=\dfrac{1}{2}\\cos2x=\dfrac{-3}{2}\left(L\right)\end{matrix}\right.\Leftrightarrow\cos2x=\dfrac{1}{2}\Leftrightarrow\left[{}\begin{matrix}2x=\dfrac{\pi}{3}+k2\pi\\2x=-\dfrac{\pi}{3}-k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k\pi\\x=-\dfrac{\pi}{6}-k\pi\end{matrix}\right.\left(k\in Z\right)\)
Chọn A
4.(1-cos22x) - 4cos2x -1 =0
-4cos22x - 4cos2x + 3 = 0
bấm máy tính giải phương trình bậc 2
cos x = \(\dfrac{1}{2}\) => cos x = cos \(\dfrac{\pi}{3}\) => x = \(\dfrac{\pi}{3}\) + k2\(\pi\)
x = - \(\dfrac{\pi}{3}\)+ k2\(\pi\)
cos x = \(\dfrac{-3}{2}\) ( vô nghiệm)