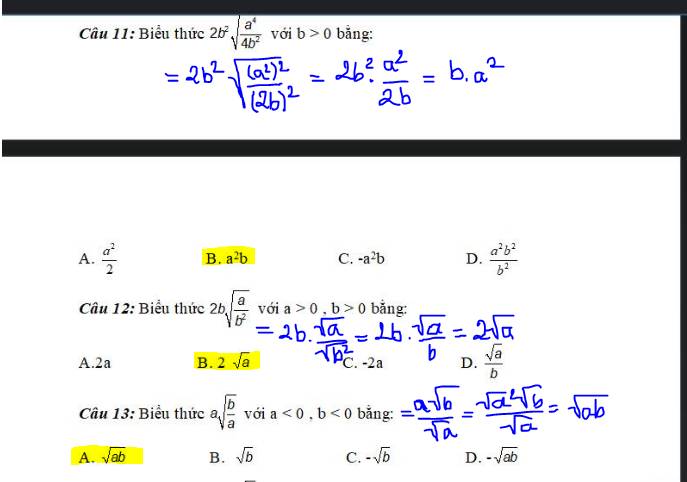
11
Với `b>0` thì:
\(2b^2\sqrt{\dfrac{a^4}{4b^2}}\\ =\sqrt{\dfrac{a^4.2b^2.2b^2}{4b^2}}\\ =\sqrt{\dfrac{a^4b^2}{1}}\\ =\sqrt{a^4b^2}\\ =a^2b\)
chọn B
12
Với `a>0`, `b>0` thì:
\(2b\sqrt{\dfrac{a}{b^2}}\\ =\sqrt{\dfrac{a.2b.2b}{b^2}}\\ =\sqrt{\dfrac{4ab^2}{b^2}}\\ =\sqrt{4a}=2\sqrt{a}\)
chọn B
13
Với `a<0`, `b<0` thì:
\(a\sqrt{\dfrac{b}{a}}\\ =\sqrt{\dfrac{b.a^2}{a}}\\ =\sqrt{b.a}\)
chọn A
Căn bậc 12, cộng 2 căn bậc 35 trừ căn bậc 12 trừ 2 căn bậc 35
Bài 1 Tìm điều kiện để căn thức \(\sqrt{-3x+6}\) có nghĩa 2) Tính a)\(\left(\sqrt{7}-\sqrt{5}\right)^2+2\sqrt{35}\) b) \(3\sqrt{8}-\sqrt{50}-\sqrt{\left(\sqrt{2-1}\right)^2}\) 3)Cho hệ phương trình \(\left\{{}\begin{matrix}4x+ay=b\\x-by=a\end{matrix}\right.\) Tìm a,b để hệ đã cho có nghiệm duy nhất (x,y)=(2;-1) Bài 2 Cho hàm số y=(2m-1)x+m-3 a) Tìm m để đồ thị hàm số đi qua điểm (2;5) b) Tìm m để đồ thị của hàm số cắt trục hoành tại điểm có hoành độ \(x=\sqrt{2}-1\) Bài 3 \(M=\frac{\sqrt{a}+3}{\sqrt{a}-2}-\frac{\sqrt{a}-1}{\sqrt{a}+2}+\frac{4\sqrt{a}-4}{4-a}\) (a>0;a khác 4) a) Rút gọn M b) Tìm a sao cho m<-2 Bài 4 Tính (a)\(\sqrt{313^2-312^2}+\sqrt{17^{2-8^2}}\left(b\right)\frac{2+\sqrt{2}}{1+\sqrt{2}}\) 2) Giai hệ phương trình\(\left\{{}\begin{matrix}2x+y=3\\3x-2y=1\end{matrix}\right.\) 3) Tìm X biết \(\sqrt{9\left(x-1\right)}=21\) Bài 5 Cho hàm số y=(m-1)x+m+3 a) Tìm giá trị của m để đồ thị của hàm số song song với đồ thị hàm số y=-2x+1 b) Tìm giá trị của m để đồ thị hàm số đi qua điểm (1;-4 ) Bài 6 Cho biểu thức \(A=\left(\frac{\sqrt{x}}{\sqrt{x-1}}+\frac{2}{x-\sqrt{x}}\right):\frac{1}{\sqrt{x}-1}\) a) Tìm đkxđ ,Rút gọn A b) Tính giá trị của A khi \(x=3-2\sqrt{2}\) Bài 7 1) Tính( a)\(\frac{\sqrt{5}}{4}-\frac{1}{\sqrt{5}+1}\left(b\right)\left(8\sqrt{27}-6\sqrt{48}\right):\sqrt{3}\) 2) Cho\(A=\left(1-\frac{4}{\sqrt{x}+1}+\frac{1}{x-1}\right):\frac{x-2\sqrt{x}}{x-1}\) Với x>0 ,x khác 1, x khác 4 a)rút gọn b) Tìm x để \(A=\frac{1}{2}\) Bài 8 Cho hàm số Y=(m-2)x+n (a)Đi qua điểm A (-1;2) và B(3;-4) (b) Cắt Oy tại điểm có tung độ bằngà cắt Ox tại điểm có hoành độ bắngìm các giá trị của m và n để đồ thị (d) của hàm số( xin cảm ơn )
\(\sqrt{29-12\sqrt{5}}\)
Cho biểu thức
A=\((\frac{1}{\sqrt{x-3}}\)+\(\frac{1}{\sqrt{x}+3})\).\(\frac{\sqrt{x+3}}{\sqrt{x}}\)
Tìm x để A nguyên
9
Chứng minh các BĐT sau: ko dùng máy tính
\(\sqrt{6}-\sqrt{2}>1\)
\(\sqrt{7}-\sqrt{2}>1\)
\(\sqrt{7}-\sqrt{6}< \sqrt{6}-\sqrt{5}\)
\(\sqrt{5}-\sqrt{3}>\frac{1}{2}\)
rút gọn biểu thức căn 2x+4 với x<-2
a, \(A=\left(\sqrt{2}+1\right)[\left(\sqrt{2}\right)^2+1][(\sqrt{2})^4+1][\left(\sqrt{2}\right)^8+1][1\left(\sqrt{2}\right)^{16}+1]\)
b, \(B=\dfrac{1}{1+\sqrt{2}}+\dfrac{1}{\sqrt{2}+\sqrt{3}}+...+\dfrac{1}{\sqrt{2019}+1\sqrt{2020}}\)
c,\(C=^3\sqrt[]{26+15\sqrt{3}}+\sqrt[3]{26-15\sqrt{3}}\)
Giải phương trình
a, \(2\sqrt{x-2}=16\)
b, \(\sqrt{x-1}>3\)
c, \(-5\sqrt{2x+4}\le-10\)
d, \(7-5\sqrt{2-x}=-8\)
e, \(\sqrt{x^2-14x+49}+x=7\)
A = \(\dfrac{3\sqrt{x}}{\sqrt{x}-6}\) với đkxđ : \(x\ge0\); x#1;x#36
B =\(\dfrac{x-6\sqrt{x}}{\sqrt{x}-1}\) với đkxđ : \(x\ge0\); x#1;x#36
Đặt T = \(\sqrt{AB}\). Tìm giá trị nhỏ nhất của biểu thức T
4x + x^2/3 +48x = 1440
