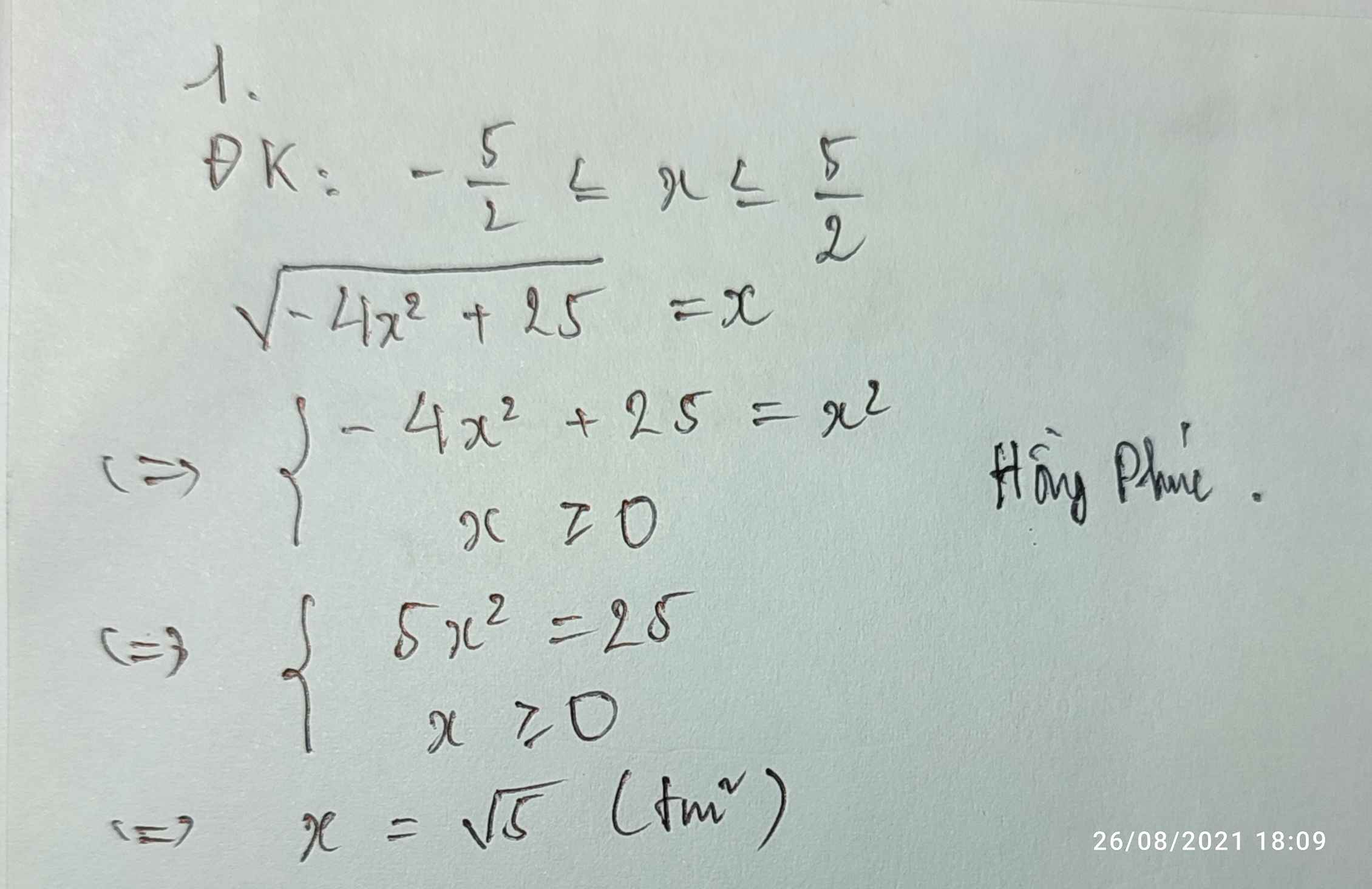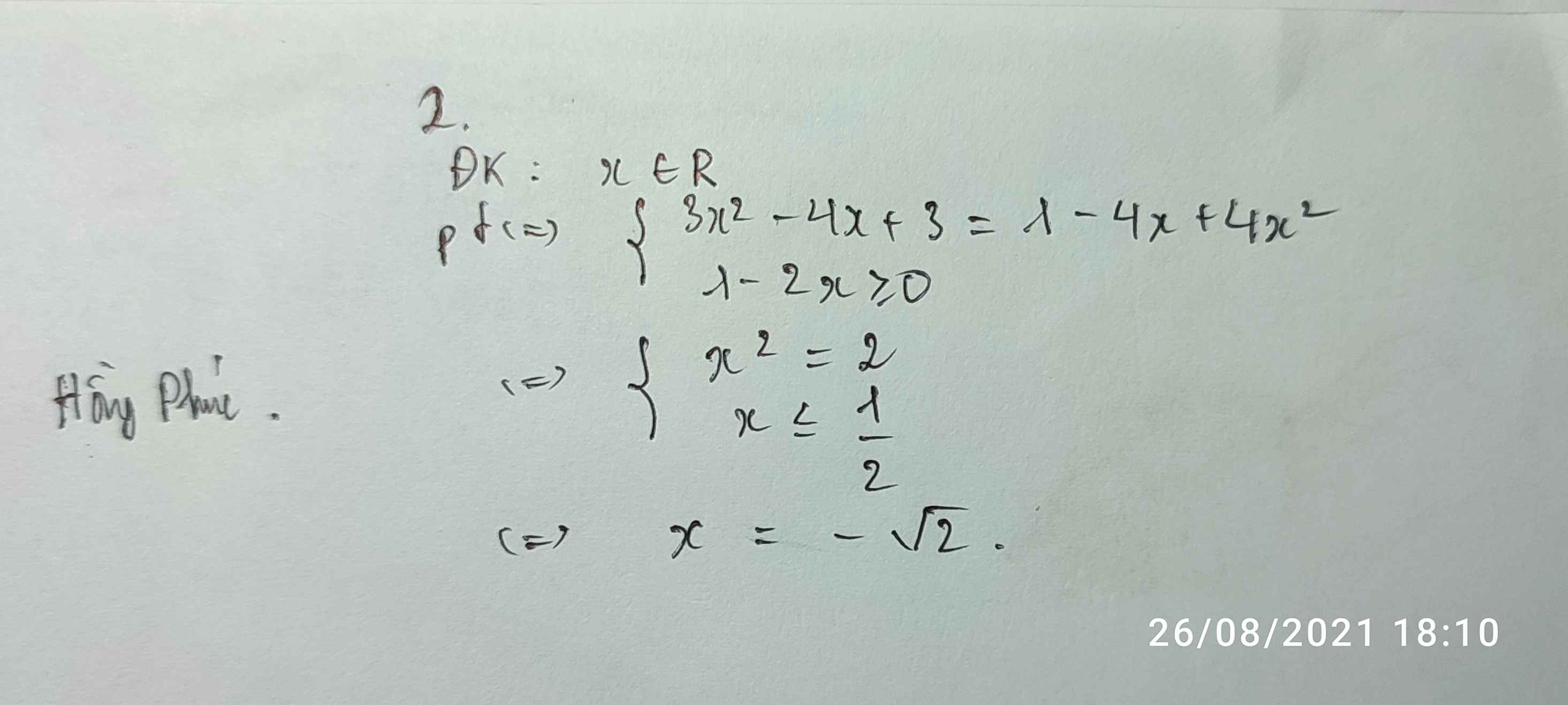Bài làm:

Ta có: \(\frac{x^2+4x^2}{\left(x+2\right)^2}=12\)

\(\Leftrightarrow5x^2=12\left(x^2+4x+4\right)\)

\(\Leftrightarrow7x^2+48x+48=0\)

\(\Leftrightarrow7\left(x^2+\frac{48}{7}x+\frac{576}{49}\right)-\frac{240}{7}=0\)

\(\Leftrightarrow\left(x+\frac{24}{7}\right)^2-\left(\frac{4\sqrt{15}}{7}\right)^2=0\)

\(\Leftrightarrow\left(x+\frac{24-4\sqrt{15}}{7}\right)\left(x+\frac{24+4\sqrt{15}}{7}\right)=0\)

\(\Leftrightarrow\orbr{\begin{cases}x=\frac{4\sqrt{15}-24}{7}\\x=-\frac{24+4\sqrt{15}}{7}\end{cases}}\)

*Bổ sung : Thiếu ĐK rồi : \(x\ne-2\)

\(ĐKXĐ:x\ne-2\)

\(Pt\Leftrightarrow x^2+4x+4+\frac{4x^2}{\left(x+2\right)^2}-4x=9\)

   \(\Leftrightarrow\left(x+2\right)^2-4x+\frac{4x^2}{\left(x+2\right)^2}=9\)

  \(\Leftrightarrow\left(x+2-\frac{2x}{x+2}\right)^2=9\)

  \(\Leftrightarrow x+2-\frac{2x}{x+2}=\pm3\)

*Với \(x+2-\frac{2x}{x+2}=3\)

\(\Leftrightarrow x^2+4x+4-2x=3\left(x+2\right)\)

\(\Leftrightarrow x^2+2x+4=3x+6\)

\(\Leftrightarrow x^2-x-2=0\)

\(\Leftrightarrow\left(x+1\right)\left(x-2\right)=0\)

\(\Leftrightarrow\orbr{\begin{cases}x=-1\\x=2\end{cases}}\)(Tm ĐKXĐ)

trường hợp còn lại làm tương tự nhé!

a.

$x^2-11=0$

$\Leftrightarrow x^2=11$

$\Leftrightarrow x=\pm \sqrt{11}$

b. $x^2-12x+52=0$

$\Leftrightarrow (x^2-12x+36)+16=0$

$\Leftrightarrow (x-6)^2=-16< 0$ (vô lý)

Vậy pt vô nghiệm.

c.

$x^2-3x-28=0$

$\Leftrightarrow x^2+4x-7x-28=0$

$\Leftrightarrow x(x+4)-7(x+4)=0$

$\Leftrightarrow (x+4)(x-7)=0$

$\Leftrightarrow x+4=0$ hoặc $x-7=0$

$\Leftrightarrow x=-4$ hoặc $x=7$

d.

$x^2-11x+38=0$

$\Leftrightarrow (x^2-11x+5,5^2)+7,75=0$

$\Leftrightarrow (x-5,5)^2=-7,75< 0$ (vô lý)

Vậy pt vô nghiệm

e.

$6x^2+71x+175=0$

$\Leftrightarrow 6x^2+21x+50x+175=0$

$\Leftrightarrow 3x(2x+7)+25(2x+7)=0$

$\Leftrightarrow (3x+25)(2x+7)=0$

$\Leftrightarrow 3x+25=0$ hoặc $2x+7=0$

$\Leftrightarrow x=-\frac{25}{3}$ hoặc $x=-\frac{7}{2}$

f.

$x^2-(\sqrt{2}+\sqrt{8})x+4=0$

$\Leftrightarrow x^2-\sqrt{2}x-2\sqrt{2}x+4=0$

$\Leftrightarrow x(x-\sqrt{2})-2\sqrt{2}(x-\sqrt{2})=0$

$\Leftrightarrow (x-\sqrt{2})(x-2\sqrt{2})=0$

$\Leftrightarrow x-\sqrt{2}=0$ hoặc $x-2\sqrt{2}=0$

$\Leftrightarrow x=\sqrt{2}$ hoặc $x=2\sqrt{2}$

g.

$(1+\sqrt{3})x^2-(2\sqrt{3}+1)x+\sqrt{3}=0$

$\Leftrightarrow (1+\sqrt{3})x^2-(1+\sqrt{3})x-(\sqrt{3}x-\sqrt{3})=0$

$\Leftrightarrow (1+\sqrt{3})x(x-1)-\sqrt{3}(x-1)=0$

$\Leftrightarrow (x-1)[(1+\sqrt{3})x-\sqrt{3}]=0$

$\Leftrightarrow x-1=0$ hoặc $(1+\sqrt{3})x-\sqrt{3}=0$

$\Leftrightarrow x=1$ hoặc $x=\frac{3-\sqrt{3}}{2}$

1,\(K=\sqrt{3-\sqrt{5}}+\sqrt{3+\sqrt{x}}\)

\(=\dfrac{1}{\sqrt{2}}\left(\sqrt{6-2\sqrt{5}}+\sqrt{6+2\sqrt{5}}\right)\)\(=\dfrac{1}{\sqrt{2}}\left(\sqrt{\left(\sqrt{5}-1\right)^2}+\sqrt{\left(\sqrt{5}+1\right)^2}\right)\)

\(=\dfrac{1}{\sqrt{2}}\left(\left|\sqrt{5}-1\right|+\sqrt{5}+1\right)\)\(=\dfrac{1}{\sqrt{2}}\left|\sqrt{5}-1+\sqrt{5}+1\right|=\dfrac{1}{\sqrt{2}}.2\sqrt{5}\)\(=\sqrt{10}\)

2, \(\sqrt{x-3}-2\sqrt{x^2-3x}=0\left(đk:x\ge3\right)\)

\(\Leftrightarrow\sqrt{x-3}\left(1-2\sqrt{x}\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x-3}=0\\1-2\sqrt{x}=0\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=3\left(tm\right)\\x=\left(\dfrac{1}{2}\right)^2=\dfrac{1}{4}\left(ktm\right)\end{matrix}\right.\)

Vậy pt có nghiệm x=3

3, \(\dfrac{9x-7}{\sqrt{7x+5}}=\sqrt{7x+5}\left(đk:x>-\dfrac{5}{7}\right)\)

\(\Leftrightarrow9x-7=7x+5\)

\(\Leftrightarrow x=6\left(tm\right)\)

4, \(x-5\sqrt{x}+4=0\)(đk: \(x\ge0\))

\(\Leftrightarrow\left(\sqrt{x}-1\right)\left(\sqrt{x}-4\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x}=1\\\sqrt{x}=4\end{matrix}\right.\)\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=16\end{matrix}\right.\) (tm)

Vậy...

1) Bạn tự làm

2) ĐK: \(x\ge3\)

PT \(\Leftrightarrow\sqrt{x-3}\left(1-2\sqrt{x}\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x-3}=0\\2\sqrt{x}=1\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=\dfrac{1}{4}\left(loại\right)\end{matrix}\right.\)

  Vậy ...

3) ĐK: \(x>-\dfrac{5}{7}\)

PT \(\Rightarrow9x-7=7x+5\) \(\Leftrightarrow x=6\)

  Vậy ...

4) ĐK: \(x\ge0\)

PT \(\Leftrightarrow x-4\sqrt{x}-\sqrt{x}+4=0\)

      \(\Leftrightarrow\left(\sqrt{x}-4\right)\left(\sqrt{x}-1\right)=0\)

      \(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x}=4\\\sqrt{x}=1\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x=16\\x=1\end{matrix}\right.\)

  Vậy ...

a) Ta có: \({\Delta _1}:3\sqrt 2 x + \sqrt 2 y - \sqrt 3  = 0 \Leftrightarrow \sqrt 2 \left( {3\sqrt 2 x + \sqrt 2 y - \sqrt 3 } \right) = 0 \Leftrightarrow 6x + 2y - \sqrt 6  = 0\)

Do đó hai đường thẳng trùng nhau.

b) Ta có: \(\frac{1}{{\sqrt 3 }} = \frac{{ - \sqrt 3 }}{{ - 3}} \ne \frac{2}{2}\), do đó hai đường thẳng song song với nhau.

c) Ta có: \(\frac{1}{3} \ne \frac{{ - 2}}{1}\), do đó hai đường thẳng cắt nhau. 

\(a,\dfrac{3}{\sqrt{x}-5}+\dfrac{20-2\sqrt{x}}{x-25}\\ =\dfrac{3}{\sqrt{x}-5}+\dfrac{20-2\sqrt{x}}{\left(\sqrt{x}-5\right)\left(\sqrt{x}+5\right)}\\ =\dfrac{3\left(\sqrt{x}+5\right)}{\left(\sqrt{x}-5\right)\left(\sqrt{x}+5\right)}+\dfrac{20-2\sqrt{x}}{\left(\sqrt{x}-5\right)\left(\sqrt{x}+5\right)}\\ =\dfrac{3\sqrt{x}+15+20-2\sqrt{x}}{\left(\sqrt{x}-5\right)\left(\sqrt{x}+5\right)}\\ =\dfrac{\sqrt{x}+35}{\left(\sqrt{x}-5\right)\left(\sqrt{x}+5\right)}\)

\(b,\dfrac{\sqrt{x}}{\sqrt{x}-3}+\dfrac{2\sqrt{x}-2}{x-9}\\ =\dfrac{\sqrt{x}}{\sqrt{x}-3}+\dfrac{2\sqrt{x}-2}{x-9}\\ =\dfrac{\sqrt{x}\left(\sqrt{x}+3\right)}{x-9}+\dfrac{2\sqrt{x}-2}{x-9}\\ =\dfrac{x+3\sqrt{x}+2\sqrt{x}-2}{x-9}\\ =\dfrac{x-5\sqrt{x}-2}{x-9}\)

a: \(\dfrac{3}{\sqrt{x}-5}+\dfrac{20-2\sqrt{x}}{x-25}\)

\(=\dfrac{3\sqrt{x}+15+20-2\sqrt{x}}{x-25}=\dfrac{\sqrt{x}+35}{x-25}\)

b: \(\dfrac{\sqrt{x}}{\sqrt{x}-3}+\dfrac{2\sqrt{x}-2}{x-9}\)

\(=\dfrac{x+3\sqrt{x}+2\sqrt{x}-2}{x-9}=\dfrac{x+5\sqrt{x}-2}{x-9}\)

c: \(\dfrac{\sqrt{x}-1}{\sqrt{x}+2}+\dfrac{5\sqrt{x}-2}{x-4}\)

\(=\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)+5\sqrt{x}-2}{x-4}\)

\(=\dfrac{x-3\sqrt{x}+2+5\sqrt{x}-2}{x-4}=\dfrac{x+2\sqrt{x}}{x-4}\)

\(=\dfrac{\sqrt{x}}{\sqrt{x}-2}\)

d: \(\left(\dfrac{x-2}{x+2\sqrt{x}}+\dfrac{1}{\sqrt{x}+2}\right)\cdot\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\)

\(=\dfrac{x-2+\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+2\right)}\cdot\dfrac{\sqrt{x}+1}{\sqrt{x}-1}\)

\(=\dfrac{\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+2\right)\left(\sqrt{x}-1\right)}=\dfrac{\sqrt{x}+1}{\sqrt{x}}\)

c: \(\Leftrightarrow x-3=0\)

hay x=3

c: 

hay x=3