Tập xác định của hàm số y=2sinx là
A. [0;2]
B. [-1;1]
C. R
D. [-2;2]
Tập xác định của hàm số y = 2 sin x là
A. [0;2]
B. [-2;2]
C. R
D. [-1;1]
Tập xác định của hàm số y=2sinx là
A. [0;2]
B. [-2;2]
C. R
D. [-1;1]
Tập xác định của hàm số là y = 2 sin x + π 4 ?
A. R
B. [-1;1]
C. (-1;1)
D. - 2 ; 2
Tập xác định của hàm số y = 2 sin x + 1 1 - cos x là
![]()
![]()
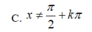
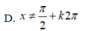
tìm tập xác định của hàm số sau: \(\dfrac{1-2sinx}{cos2x}\)
cos2x ≠ 0
⇔ cos 2x ≠ \(\dfrac{\pi}{2}+k\pi\)
⇔ x ≠ \(\dfrac{\pi}{4}+k\dfrac{\pi}{2}\)
Cho hàm số y=2x. Khẳng định nào sau đây là sai?
A. Tập xác định của hàm số là R.
B. Tập giá trị của hàm số là (0;+∞).
C. Đồ thị của hàm số cắt trục Ox tại đúng một điểm.
D. Hàm số đồng biến trên tập xác định của nó.
Đồ thị này cắt trục Ox tại rất nhiều điểm chứ không phải chỉ có 1 điểm
=>Chọn C
Câu 1: Tìm tập xác định của hàm số y=\(\dfrac{cosx-2}{1-2sinx}\)
Câu2 : Tìm m để hàm số y=\(\sqrt{m-1+2cosx}\) xác đinh trên R
câu3 : Tìm số điểm biểu diễn nghiệm của pt: 2cos5x+1
giúp e với mn ơi
1.
Hàm số xác định khi: \(1-2sinx\ne0\Leftrightarrow sinx\ne\dfrac{1}{2}\Leftrightarrow\left[{}\begin{matrix}x\ne\dfrac{\pi}{6}+k2\pi\\x\ne\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
2.
Đặt \(t=cosx\left(t\in\left[-1;1\right]\right)\)
Hàm số xác định trên R khi:
\(m-1+2cosx\ge0\forall x\in R\)
\(\Leftrightarrow m\ge f\left(t\right)=1-2t\forall x\in R\)
\(\Leftrightarrow m\ge maxf\left(t\right)=f\left(-1\right)=3\)
Vậy \(m\ge3\)
tìm tập xác định của các hàm số:
1.y=sin2x
2.y=\(\dfrac{1-cosx}{sinx}\)
3.y=\(\dfrac{1-2sinx}{cos2x}\)
4.y=tan\(\left(x+\dfrac{\pi}{4}\right)\)
1. \(D=R\)
2. \(sinx\ne0\Leftrightarrow x\ne k\pi\Rightarrow D=R\backslash\left\{k\pi|k\in R\right\}\)
3. \(cos2x\ne0\Leftrightarrow2x\ne\dfrac{\pi}{2}+k\pi\Leftrightarrow x\ne\dfrac{\pi}{4}+\dfrac{k\pi}{2}\Rightarrow D=R\backslash\left\{\dfrac{\pi}{4}+\dfrac{k\pi}{2}|k\in R\right\}\)
4. \(cos\left(x+\dfrac{\pi}{4}\right)\ne0\Leftrightarrow x+\dfrac{\pi}{4}\ne\dfrac{\pi}{2}+k\pi\Leftrightarrow x\ne\dfrac{\pi}{4}+k\pi\Rightarrow D=R\backslash\left\{\dfrac{\pi}{4}+k\pi|k\in R\right\}\)
tìm tất cả giá trị của m để hàm số sau có tập xác định R
a)y=\(\sqrt{m-cosx}\)
b)y=\(\sqrt{2sinx-m}\)
c)y=\(\dfrac{sinx-1}{cosx+m}\)
a.
\(\Leftrightarrow m-cosx\ge0\) ; \(\forall x\)
\(\Leftrightarrow m\ge max\left(cosx\right)\)
\(\Leftrightarrow m\ge1\)
b.
\(\Leftrightarrow2sinx-m\ge0\) ; \(\forall x\)
\(\Leftrightarrow m\le2sinx\) ; \(\forall x\)
\(\Leftrightarrow m\le\min\limits_{x\in R}\left(2sinx\right)\)
\(\Leftrightarrow m\le-2\)
c.
\(\Leftrightarrow cosx+m\ne0\) ; \(\forall x\)
\(\Leftrightarrow\left[{}\begin{matrix}m>\max\limits_R\left(cosx\right)\\m< \min\limits_R\left(cosx\right)\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}m>1\\m< -1\end{matrix}\right.\)