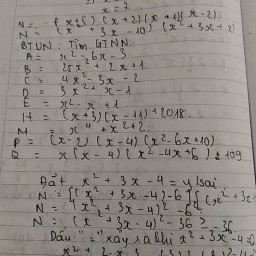f) (2x - 8)(4x + 16) = 0

<=> 2x - 8 = 0 hoặc 4x + 16 = 0

<=> 2x = 0 + 8 hoặc 4x = 0 - 16

<=> 2x = 8 hoặc 4x = -16

<=> x = 4 hoặc x = -4

g) 5x(6x - 12) = 0

<=> 5x = 0 hoặc 6x - 12 = 0

<=> x = 0 hoặc 6x = 0 + 12

<=> x = 0 hoặc 6x = 12

<=> x = 0 hoặc x = 2

h) 7(9 - x)(12 - 6x) = 0

<=> 9 - x = 0 hoặc 12 - 6x = 0

<=> -x = 0 - 9 hoặc -6x = 0 - 12

<=> -x = -9 hoặc -6x = -12

<=> x = 9 hoặc x = 2

tích mình đi

ai tích mình 

mình tích lại 

thanks

\(x\left(x-3\right)+x-3=0\)

\(\left(x-3\right)\left(x+1\right)=0\)

\(\Rightarrow\orbr{\begin{cases}x-3=0\\x+1=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=3\\x=-1\end{cases}}}\)

KL:......................

\(x^3-5x=0\)

\(x\left(x^2-5\right)=0\)

Làm  tương tự như câu a

@_@ n...h..i......ề....u  q...u.....................á!

\(-5x^2-6x-12\)

\(=-5\left(x^2+\frac{6}{5}x+\frac{12}{5}\right)\)

\(=-5\left(x^2+2\cdot x\cdot\frac{3}{5}+\frac{9}{25}+\frac{51}{25}\right)\)

\(=-5\left[\left(x+\frac{3}{5}\right)^2+\frac{51}{25}\right]\)

Vì \(\left(x+\frac{3}{5}\right)^2+\frac{52}{25}>0\forall x\)

\(\Rightarrow-5\left[\left(x+\frac{3}{5}\right)^2+\frac{52}{25}\right]< 0\forall x\)

\(-5x^2-6x-12\)

nhìn ở đây ta sẽ biết :

\(-5x^2\)sẽ là một số âm

\(6x\) có thể âm hoặc dương

Ta thấy : \(-5x^2\le6x\)

và lại biểu thức trên còn có -12 nữa

=> \(-5x^2-6x-12< 0\) ( với mọi \(x\))

\(\Leftrightarrow x\in\left\{2;-2\right\}\)

\(\Rightarrow\left(x-2\right)\left(x+2\right)=0\\ \Rightarrow\left[{}\begin{matrix}x=2\\x=-2\end{matrix}\right.\)

(5x - 10) . (6x + 12) = 0 

=>(5x - 10) = 0 hoặc (6x + 12) = 0 

 * TH1: (5x - 10) = 0           * TH2: (6x + 12) = 0 

             =>x = 2                             => x = -2

                     Vậy x=2 hoặc x = -2

\(\Leftrightarrow30x^2-72x+55x-132-42x+66=0\\ \Leftrightarrow30x^2-59x-66=0\\ \Delta=3481-4\cdot\left(-66\right)\cdot30=11401\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{59-\sqrt{11401}}{60}\\x=\dfrac{59+\sqrt{11401}}{60}\end{matrix}\right.\)

\(\Leftrightarrow x^4-2x^3-\left(m+3\right)x^2-4x^3+8x^2+4\left(m+3\right)x+mx^2-2mx-m^2-3m=0\)

\(\Leftrightarrow x^2\left(x^2-2x-m-3\right)-4x\left(x^2-2x-m-3\right)+m\left(x^2-2x-m-3\right)=0\)

\(\Leftrightarrow\left(x^2-4x+m\right)\left(x^2-2x-m-3\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x^2-4x+m=0\\x^2-2x-m-3=0\end{matrix}\right.\)

Pt có 4 nghiệm khi: \(\left\{{}\begin{matrix}\Delta'_1=4-m\ge0\\\Delta'_2=1+m+3\ge0\end{matrix}\right.\)

\(\Leftrightarrow-4\le m\le4\)

a, đặt ( x²+x)=y ta có :

y²+4y=12 <=> y²+4y-12=0

<=> y²+4y+4-16 =0

<=>(y²+4y+4)-16+=0

<=> (y+2)²-16=0

<=>(y-2)(y+6)=0

<=>y-2=0 hoặc y+6=0

<=> y=2 hoặc y=-6

<=> x²+x=2 hoặc x²+x=-6

<=> x²+x -2=0 hoặc x²+x+6=0(vô lý)

<=> (x-1)(x+2)=0 <=> x-1=0 hoặc x+2=0

<=> x=1 hoặc x=-2

vậy pt có nghiệm là x=1 và x=-2

b,6x⁴-5x³-38x²-5x+6=0

<=>6x⁴-18x³+13x³-39x²+x²-3x-2x+6=0

<=>6x³(x-3)+13x²(x-3)+x(x-3)-2(x-3)=0

<=>(x-3)(6x³+13x²+x-2)=0

<=>(x-3)(6x³+12x²+x²+2x-x-2)=0

<=>(x-3)(6x²(x+2)+x(x+2)-(x+2))=0

<=>(x-3)(x+2)(6x²+x-1)=0

<=>(x-3)(x+2)(3x-1)(2x+1)=0

tới đây tự làm

1/ x²-3x+2=0

⇒ (x²-2x)-(x-2)=0

⇒ x(x-2)-(x-2)=0

⇒ (x-1)(x-2)=0

\(\Rightarrow\left[{}\begin{matrix}x-1=0\\x-2=0\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=1\\x=2\end{matrix}\right.\)

2) x²-6x+5=0

⇒x²-6x+9-4=0

⇒(x²-6x+9)-2²=0

⇒(x-3)²-2²=0

⇒(x-3-2)(x-3+2)=0

⇒(x-5)(x-1)=0

\(\Rightarrow\left[{}\begin{matrix}x-1=0\\x-5=0\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=1\\x=5\end{matrix}\right.\)

3) 2x²+5x+3=0

⇒ (2x²+2x)+(3x+3)=0

⇒ 2x(x+1)+3(x+1)=0

⇒ (x+1)(2x+3)=0

\(\Rightarrow\left[{}\begin{matrix}x+1=0\\2x+3=0\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=-1\\x=-1,5\end{matrix}\right.\)

4) x²-8x+15=0

⇒ (x²-8x+16)-1=0

⇒ (x-4)²-1²=0

⇒ (x-4-1)(x-4+1)=0

⇒ (x-5)(x-3)=0

\(\Rightarrow\left[{}\begin{matrix}x-3=0\\x-5=0\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=3\\x=5\end{matrix}\right.\)

5) x²-x-12=0

⇒ (x²-4x)+(3x-12)=0

⇒ x(x-4)+3(x-4)=0

⇒ (x-4)(x+3)=0

\(\Rightarrow\left[{}\begin{matrix}x+3=0\\x-4=0\end{matrix}\right.\\ \Rightarrow\left[{}\begin{matrix}x=-3\\x=4\end{matrix}\right.\)

1: Ta có: \(x^2-3x+2=0\)

\(\Leftrightarrow\left(x-1\right)\left(x-2\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=2\end{matrix}\right.\)

2: Ta có: \(x^2-6x+5=0\)

\(\Leftrightarrow\left(x-1\right)\left(x-5\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=5\end{matrix}\right.\)

3: Ta có: \(2x^2+5x+3=0\)

\(\Leftrightarrow\left(x+1\right)\left(2x+3\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-\dfrac{3}{2}\end{matrix}\right.\)

4: Ta có: \(x^2-8x+15=0\)

\(\Leftrightarrow\left(x-3\right)\left(x-5\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=3\\x=5\end{matrix}\right.\)

5: Ta có: \(x^2-x-12=0\)

\(\Leftrightarrow\left(x-4\right)\left(x+3\right)=0\)

\(\Leftrightarrow\left[{}\begin{matrix}x=4\\x=-3\end{matrix}\right.\)